1
$\begingroup$

My question is quite fundamental - I've read plenty of texts that report a critical magnetic field for AFM materials. If I'm right, these materials have spins pointing in opposite directions in adjacent magnetic atoms in a unit cell (consider say intralayer AFM for simplicity). My question is, what happens on application of a magnetic field? Is there always a lower energy configuration that could be achieved by application of a critical magnetic field?

Any help would be much appreciated.

Improve this question
$\endgroup$

1 Answer 1

Reset to default
1
+50
$\begingroup$

With antiferromagnetic materials, what happens when you apply an external magnetic field depends upon the direction the field is applied relative to the spin axis.

The response of a material to an external magnetic field is the magnetic susceptibility, $\chi$. It is the proportionality constant between magnetization, M, the magnetic moment per unit volume, and the applied magnetic field. $$M=\chi B$$ For an antiferromagnetic material, there are two fundamental directions to study: perpendicular to the spin axis and parallel to the spin axis.

Here is what $\chi$ looks like for $MnF_2$ in both of these directions:

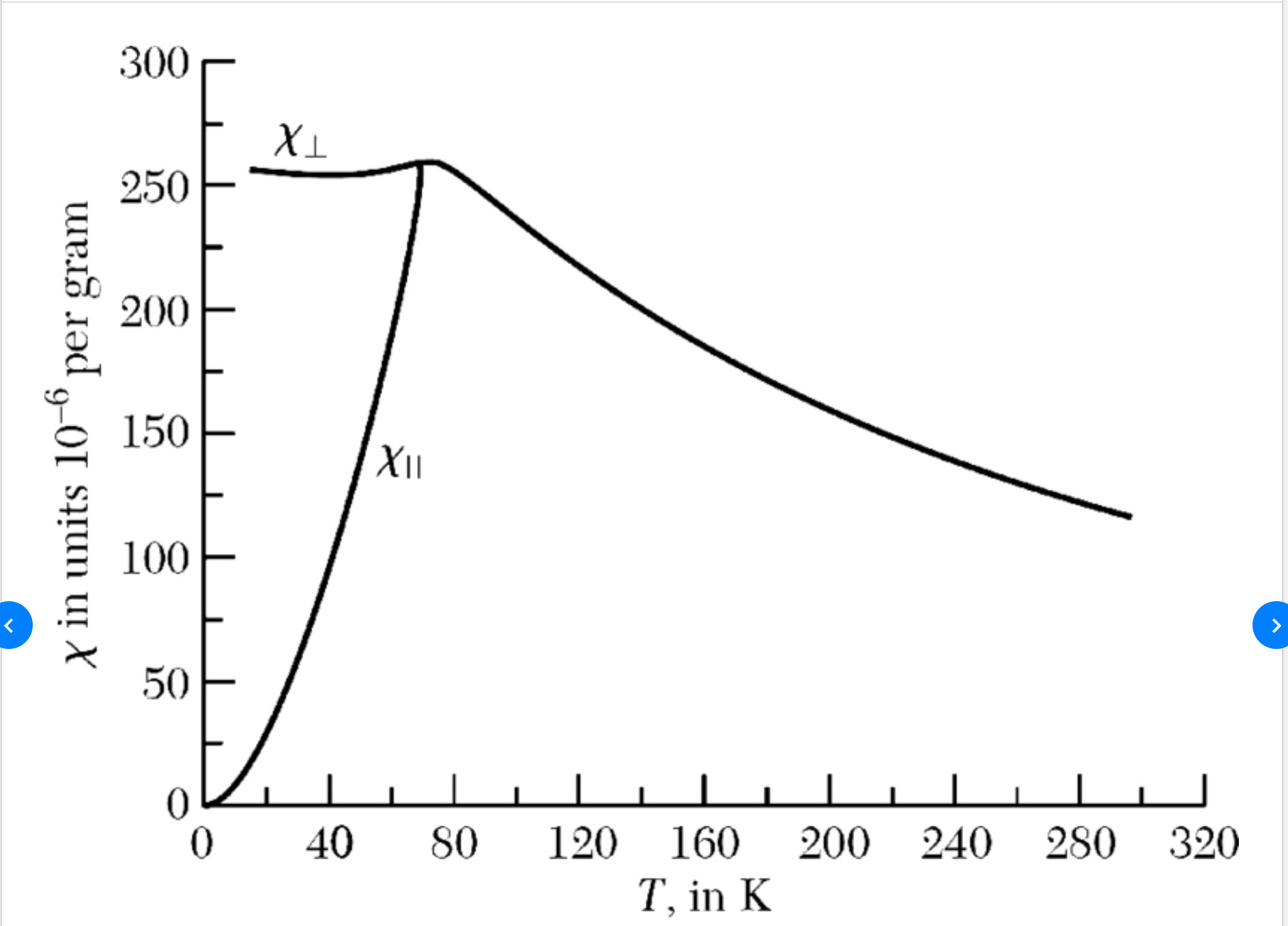
Magnetic susceptibility of manganese flouride parallel and perpendicular to the AFM spin axis, from S. Foner, Antiferromagnetic and ferrimagnetic resonance, Magnetism I, 384

Kittel, in Introduction to Solid State Physics, reports that with a strong magnetic field applied in the parallel direction, the spin system will turn from the parallel orientation to the perpendicular orientation because that lowers the energy. This change happens discontinuously, not continuously. If the magnetic field is already pointing perpendicular to the spin axis, there is no lower energy configuration. NOTE: This is not a change to a ferromagnetic state!

But this paper: How to manipulate magnetic states of antiferromagnets DOES report on page 11 on the change from an AFM state to a FM state in FeRh films at 350K. This is called a Metamagnetic transition. (This term it seems generally refers any sudden change in magnetization with a small change in applied magnetic field.)

Here too is a report on single crystals of $UAu_{0.8}Sb_2$ also on a similar transition, but at much colder temperatures (34K). The metamagnetic transitions can also be induced by the application of a magnetic field. They see two transitions at 0.9 and 1.9T. A chart here gives relative strengths of magnets.

Sorry, I can't find a general reference on Metamagnetic transitions.

Improve this answer
$\endgroup$
10
  • $\begingroup$ Thanks for the answer @CGS. For a person who's fairly new to magnetic theory, would you be able to comment how big 9Tesla actually is? $\endgroup$
    – livars98
    Commented Dec 29, 2020 at 4:39
  • $\begingroup$ Hi @livars98. I've updated my answer. Hope it helps! If you have more questions, let me know! $\endgroup$
    – CGS
    Commented Dec 29, 2020 at 12:28
  • $\begingroup$ Hi@livars98. I'm glad I re-read the title to your question! It made me look deeper into this subject and discover the concept of metamagnetic transitions, which is I think what you are referring to. I hope this helps! $\endgroup$
    – CGS
    Commented Dec 29, 2020 at 13:38
  • $\begingroup$ @CGS I think there is an error in the answer. Applying a magnetic field parallel to spins doesn't impart a torque and hence there is no precession of spins (if you assume zero temperature, no magnons etc). Applying a perpendicular magnetic field will slowly rotate the spins. I think you got these two mixed up. $\endgroup$
    – Xivi76
    Commented Jan 15, 2021 at 8:14
  • 1
    $\begingroup$ Hi @Xivi76 , my apologies, you are correct that what I wrote in my last comment is not what Kittel meant. What I understand Kittel to have meant is exactly what I wrote in the original post. The quote from Kittel above comes at the end of a section where he is writing about the magnetic susceptibility in AFM materials when the magnetic field is parallel to the spin axis. Unfortunately there is no reference. I can't tell you if he has made a mistake, but I doubt it. I can only urge you to get your own copy of Kittel and read the section for yourself. $\endgroup$
    – CGS
    Commented Jan 17, 2021 at 13:45

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.