0
$\begingroup$

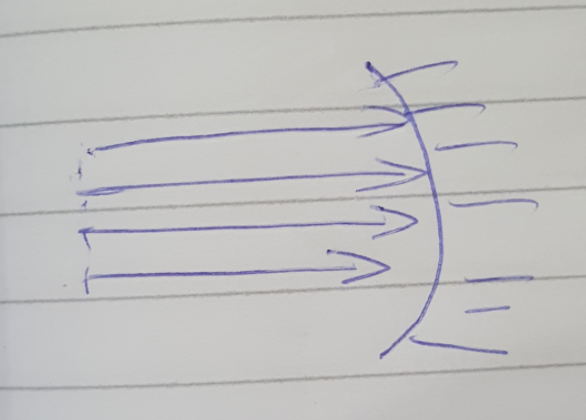
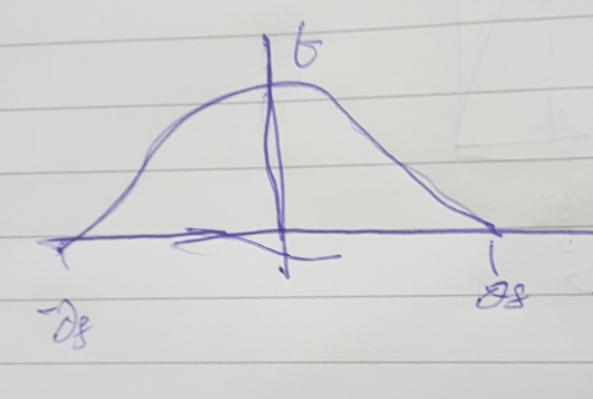
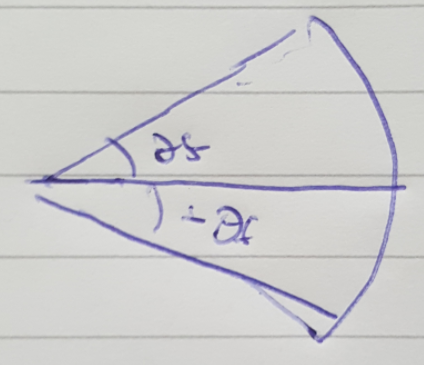
enter image description here

Suppose a light source is kept from a mirror at a distance x from the topmost part of the mirror(*). As we take the distance of points that are more and more interior to the mirror, we find that the distance to it from the light source is increased from the topmost to the middlemost point, after which the distance drops off again. If we were to plot the time taken by light rays to reach the points as a function of angle $\theta$ where $\theta $ is the angle relative to a horizontal line that passes through the pole of the mirror. The graph would look something like this:

enter image description here

$ \theta_f$ being the maximum angle attainable:

enter image description here

Now, what are the consequence of light rays in a parallel beam taking a longer time to hit some spots on a mirror and less time to hit others?

Improve this question
$\endgroup$
5
  • $\begingroup$ Hard to give you an answer until you say a bit more about what you already know. Are you familiar with the least-time principle in optics for example? $\endgroup$
    – Andrew Steane
    Commented Dec 14, 2020 at 10:44
  • $\begingroup$ Yes I'm aware of the least time principle $\endgroup$
    – Brian
    Commented Dec 14, 2020 at 10:59
  • $\begingroup$ @AndrewSteane fermat's principle? $\endgroup$
    – Anonymous
    Commented Dec 14, 2020 at 11:23
  • 1
    $\begingroup$ The consequence is that you don't get a geometric "perfect dot" image at focus, but rather the diffraction pattern due to the phase mismatch over all path lengths in the cone defined by your $\theta$ $\endgroup$
    – Carl Witthoft
    Commented Dec 14, 2020 at 15:52
  • $\begingroup$ @CarlWitthoft interesting. $\endgroup$
    – Brian
    Commented Dec 15, 2020 at 0:03

1 Answer 1

Reset to default
0
$\begingroup$

The least time principle (Fermat's principle) in optics says that a ray path between given points lies at a local minimum (or a local maximum, but that case is rare) of the time taken by light to travel between those points by all available paths. For example, in free space with no reflection or refraction a straight line is such a path.

In the presence of a mirror, we are interested in paths which may go from $A$ to $B$ via a reflection off the mirror. Say the reflection might be at either of points $P_1$ or $P_2$ on the mirror surface. Then if $AP_1$ is longer than $AP_2$, then $AP_1B$ will be longer than $AP_2B$ unless $P_2B$ is longer than $P_1A$. With the curved mirror, choose for $A$ a point a long way away, and for $B$ a point at one focal length in front of the mirror. Let's call that point $F$. Then the shape of the mirror is such that the distances $APF$ are all the same, for $P$ anywhere on the mirror surface. For central parts of the mirror surface, $AP$ is long and $PF$ is short. For outer parts, $AP$ is short and $PF$ is long. By arranging that $APF$ is constant for all reflection points $P$ on the mirror surface, you arrange that light arriving from any part of the collimated beam will all be directed to $F$, because all the paths involved are equally the shortest path.

Improve this answer
$\endgroup$
2
  • $\begingroup$ ", you arrange that light arriving from any part of the collimated beam will all be directed to F, because all the paths involved are equally the shortest path." Woah, this is really a big idea to digest. I liked this answer that the reflected path offsets the increased distance to reached the mirror but I think this answer really needs a diagram to go with it. $\endgroup$
    – Brian
    Commented Dec 14, 2020 at 12:19
  • $\begingroup$ While this is true, it could be more simply stated this way: Yes, there is a path-length difference, which leads to a phase difference at the focal point. This is where simple raytracing fails, and we have to use diffraction theory, leading to things like Cornu spiral and Airy disks to describe the intensity pattern at the theoretical focus point. $\endgroup$
    – Carl Witthoft
    Commented Dec 14, 2020 at 15:50

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.