EM Waves: Are $k$ and $E$ always perpendicular?

Question

I was learning about plane waves in class and it was stated that $k$-vector is perpendicular to $E$ which is also perpendicular to $B$-field.

But is this always the case?

Answer 1

$\vec{k}$ and $\vec{E}$ are perpendicular if and only if the charge density in the region is zero. This follows from Gauss' law:

Let $\vec{E}(\vec{r},t) = \vec{E}_0 e^{i (\vec{k}\cdot\vec{r} - \omega t)}$. Then $\vec{\nabla}\cdot\vec{E} = i\vec{k}\cdot\vec{E}$.

Gauss' law says $\vec{\nabla}\cdot\vec{E} = \rho/\epsilon_0$, and $\vec{k}\cdot\vec{E}$ is zero for nonzero $\vec{k}$ and $\vec{E}$ if and only if $\vec{k} \perp \vec{E}$, so $\vec{k} \perp \vec{E} \iff \rho = 0.$

Answer 2

The idea of the electric and magnetic fields being perpendicular to the wavevector can be seen quite nice in the Fourier representation of the fields, i.e. $$F(\vec r) = \int_{\mathbb{R}^3} F(\vec k) e^{i\vec k \vec r}$$ where $F$ stands for the fields $\vec E$ and $\vec B$, the charge density $\rho$ and the current density $\vec j$. We now express the Maxwell equations in this Fourier representation. The equations $\nabla \cdot \vec B(\vec r) = 0$ and $\nabla \cdot \vec D(\vec r) = \rho(\vec r)$ then read $$i\vec k \cdot \vec B(\vec k) = 0 \quad\text{ and }\quad i\vec k \cdot \vec D(\vec k) = \rho(\vec k).$$ We can already see the strict orthogonality of the wavevector $\vec k$ and the amplitude of the corresponding magnetic field mode $\vec B(\vec k)$. On the other hand, the electric displacement field $\vec D$ can be seen to be orthogonal to the wavevector if the charge density $\rho(\vec k)$ vanishes. When thinking about a "free wave" travelling through space with some charges around, one can think of this as a superposition effect, as those charges induce an electric field that is added to the wave's field.

We can already conclude, that in a linear isotropic medium (one satisfying $\vec D=\epsilon \vec E$ and $\vec B = \mu \vec H$) without free charges, both fields are orthogonal to the wave vector $\vec k$ in the sense that the respective field modes $\vec E(\vec k),\vec B(\vec k) \perp \vec k$ respectively. This, however, is not true in general. In a linear anisotropic medium (for example here) the fields $E$ and $B$ are connected to the effectiv fields $D$ and $H$ by matrix equations, i.e. $\epsilon$ and $\mu$ are not scalars anymore but matrices. Still, in this case the displacement field $\vec D$ and the magnetic field $\vec B$ are orthogonal to $\vec k$, but not necessarily the electric field $\vec E = \epsilon^{-1} \vec D$. In this case, the eigenvectors of $\epsilon$ play an important role.

Anyway, we still don't know anything about the directional relation of the electric and magnetic field to each other. For this, we transform the other two Mexwell equations, $\nabla\times\vec H(\vec r) = \partial_t \vec D(\vec r) + \vec j(\vec r)$ as well as $\nabla \times \vec E(\vec r) = -\partial_t \vec B(\vec r)$, into Fourier representation. These yield $$i\vec k \times \vec H(\vec k) = \partial_t \vec D(\vec k) + \vec j(\vec k)\quad\text{ and }\quad i\vec k \times \vec E(\vec k) = - \partial_t \vec B(\vec k).$$

We again assume a linear isotropic medium without free charge or current density. We will focus on the electric field for simplicity. From the properties of the vector product follows that its change in time $\partial_t E(\vec k)$ is perpendicular to both $\vec k$ and $\vec B(\vec k)$. On the other hand we can show that $$\epsilon\mu\partial_t^2 \vec E(\vec k) = - (i\vec k)^2 \vec E(\vec k)$$ which is the Fourier representation of the wave equation. From these points, it's a straight foreward chain of implications to $\vec E(\vec k) \perp \vec B(\vec k)$. However, this is again not true in general if the medium is not isotropic.

To conclude, in a linear homogeneous and isotropic medium it is true that the vectors $\vec k$, $\vec E$ and $\vec B$ are perpendicular to each other in the mode decomposition, i.e. Fourier transformed representation. Free charges disturb this characteristic, but due to the linearity of electrodynamics we can extract the wave part for which this is true. I hope this helps understanding the foundations of the orthoganality. Cheers!

Answer 3

In the theory of "linear wire antennas", it is pointed out that longitudinal electric field (Er) is not zero close to radiating wire antenna. See the equation (4-10a) of Balanis's book1.　See below.

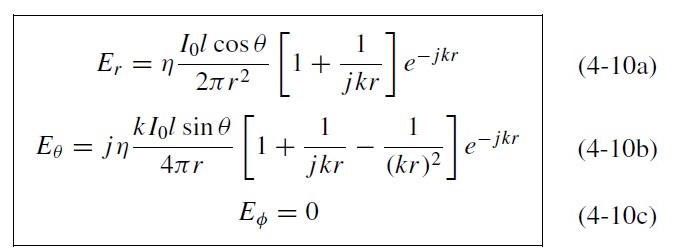
Of course, the charge distribution is non-zero on the metal antenna.

1 C. A. Balanis, Antenna Theory, Analysis and Design, Third Edition, Wiley.