Why doesn't Gaussian wavepacket broadening in position mean there will be a shortening in momentum?

Question

Many sources that say in free broadening of a Gaussian wavepacket, the momentum uncertainty (I think defined in terms of the range of 'significant' momentum amplitudes) is time invariant even as the Gaussian wavepackets broadens in position.

I'm trying to reconcile how this is consistent with the uncertainty principle.

My idea is that the uncertainty principle only loosely forces the 'bulk area' of the momentum distribution to have a spread that is inversely proportional to the 'bulk area' of the position distriubtion, but in special cases like this maybe the 'bulk area' is rearranged in such a way that the amplitudes of the momenta inside the original 'significant amplitude region' gets much larger, but not much wider?

Any thoughts?

Extras:

The reason I had this thinking about 'bulk areas' was because I was wondering how exactly the fourier transform of the position wavefunction (i.e. giving wavefunction in momentum space) is changed after this wavepacket broadening. Obviously its standard deviation hasn't changed, but I would expect there to be some difference since I though the FT doesn't lose any information from its input and its input has definitely changed. Otherwise you wouldn't be able to get back to this 'broadened' position wavefunction from the momentum wavefunction using an inverse FT.

Answer 1

the momentum uncertainty (I think defined in terms of the range of 'significant' momentum amplitudes)

I'm not sure what you mean by this "range of significant momentum amplitudes". Uncertainty has an exact definition in QM. If you have a state vector $|\psi\rangle$ then the average of some observable $A$ is $\langle A\rangle=\langle\psi|A|\psi\rangle$, and the uncertainty is just the square root of the variance:

$$(\Delta A)^2=\left\langle \left(A-\langle A\rangle\right)^2\right\rangle$$

My idea is that the uncertainty principle only loosely forces the 'bulk area' of the momentum distribution to have a spread that is inversely proportional to the 'bulk area' of the position distriubtion, but in special cases like this maybe the 'bulk area' is rearranged in such a way that the amplitudes of the momenta inside the original 'significant amplitude region' gets much larger, but not much wider?

Here too, I'm not sure how useful this "bulk area" idea is. You can directly calculate how the uncertainties of position and momentum evolve over time for the free Gaussian wave packet evolving according to the Schrodinger equation. Then you can show that the position uncertainty increases while the momentum uncertainty remains constant.

As pointed out in other answers, this doesn't violate the uncertainty principle. A violation would consist of decreasing uncertainties such that $\Delta x\Delta p\geq\hbar/2\pi$ is no longer valid.

To add some intuition here, the free particle Hamiltonian has no position dependence. Therefore, the propagator commutes with the momentum operator, and so any expectation values, variances, etc. for momentum measurements must be time independent.

The reason I had this thinking about 'bulk areas' was because I was wondering how exactly the fourier transform of the position wavefunction (i.e. giving wavefunction in momentum space) is changed after this wavepacket broadening. Obviously its standard deviation hasn't changed, but I would expect there to be some difference since I though the FT doesn't lose any information from its input and its input has definitely changed. Otherwise you wouldn't be able to get back to this 'broadened' position wavefunction from the momentum wavefunction using an inverse FT.

We need to be careful here in distinguishing the wave functions (in terms of position or momentum) to the probability distributions, which involves the squares of the wave functions. Indeed, the wave functions are related by Fourier Transforms, but the probability amplitudes are not.

Assuming an initial wave packet of the form $\Psi(x,0)=Ae^{-ax^2}$ one can determine $\Psi(x,t)$ and $\Phi(p,t)$ for the free particle. I will not go through derivations, but this should be covered in various QM texts, or online. For reference here I was using Griffith's QM book section 2.4 and problem 2.22. Below are the real and imaginary parts of both $\Psi(x,t)$ and $\Phi(p,t)$ evolving over time (I have set all constants to $1$, so the actual numbers are not important):

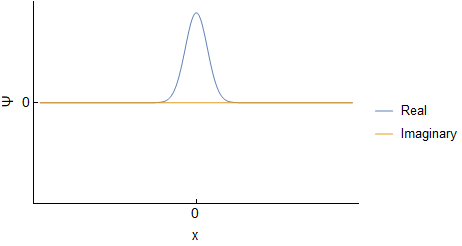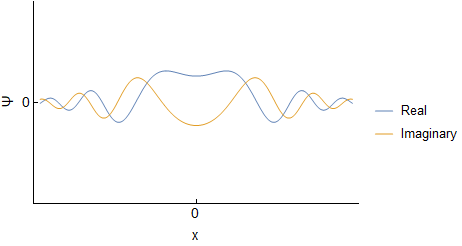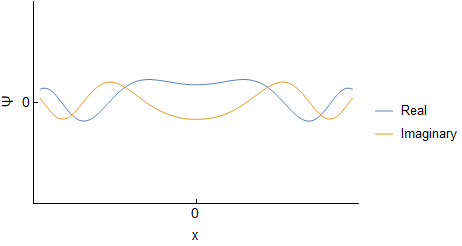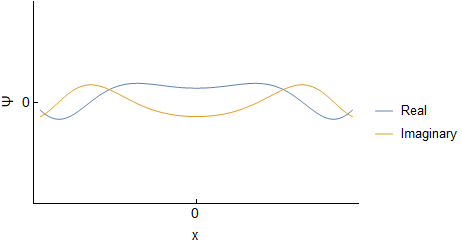
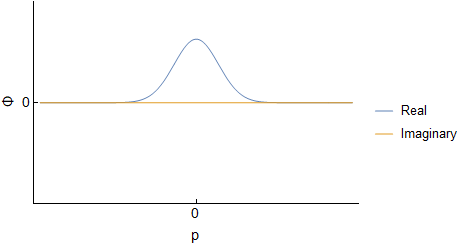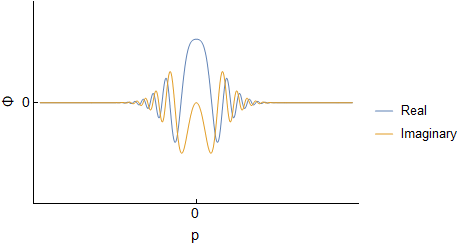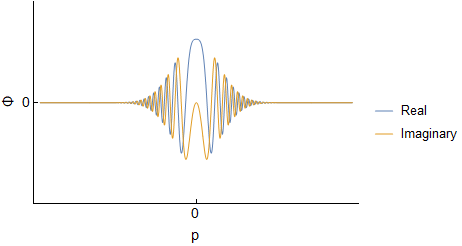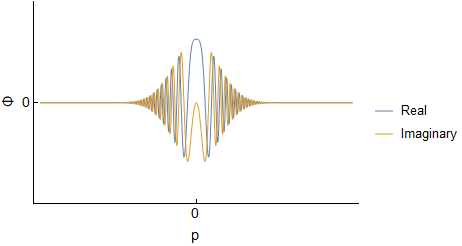
We can see that both $\Psi$ and $\Phi$ are changing, and at each time they are related through a Fourier Transform. However, this idea does not carry over into $|\Psi|^2$ and $|\Phi|^2$; they are not related via direct Fourier transform:

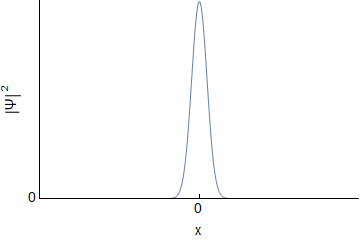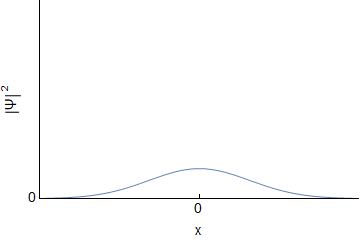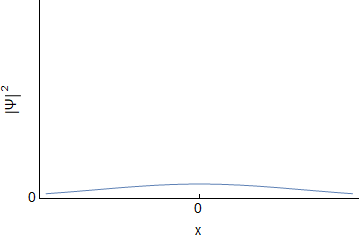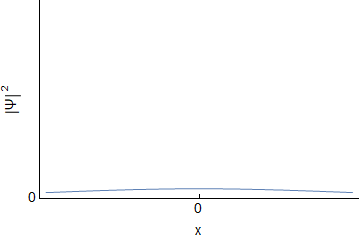
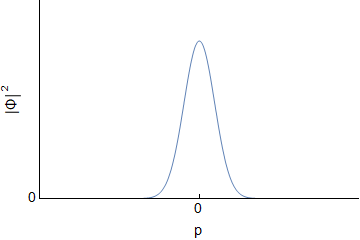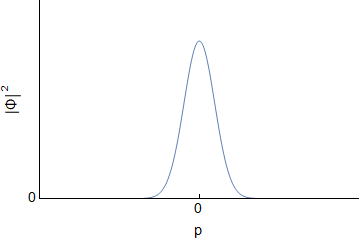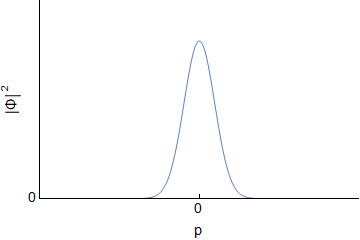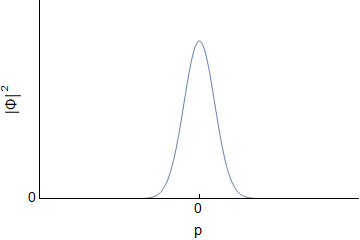
Since the probabilities determine the statistics, we can see that the uncertainty doesn't change for momentum, but it gets larger for position. The uncertainty principle is still followed.

Answer 2

The uncertainty principle is simply $$\Delta X\Delta P\geq\frac{\hbar}{2}$$ or $$\Delta P\geq\frac{\hbar}{2\Delta X}$$ If you increase $\Delta X$, the bound on $\Delta P$ is strictly lowered, not raised. Thus, $\Delta P$ staying constant as $\Delta X$ increases is a perfectly viable situation according to the uncertainty principle.

Answer 3

I’ll just say a word on Fourier transforms, which might help clear up your intuition:

Fourier transforms are complex.

This means, for example, when transforming from position space to momentum space, there is a phase associated with each momentum value, in addition to amplitude.

If the momentum phase is uniform, this means the position spread is “transform limited”, i.e. minimum according to uncertainty principle. If, on the other hand, the momentum phase is non-uniform, then the position is “chirped” or worse. Then the position will be further spread out for the same momentum amplitude spectrum.

Edit to directly answer the question:

“Why doesn’t Gaussian waveform broadening in position mean there will be a shortening in momentum?”

Because the broadening in position can, in certain circumstances, be represented by a change only in the phases of the momentum spectrum (like a chirped wave, for example). Then the amplitudes of the momentum spectrum would remain the same.