1
$\begingroup$

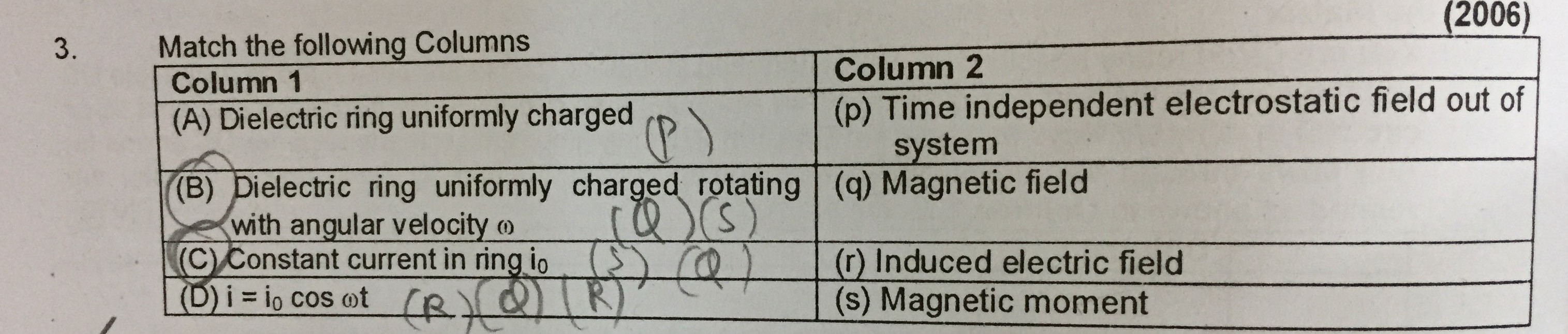
Consider a non-conducting ring with positive charge fixed on uniformly . This arrangement is rotated about the axis of the ring with an uniform angular velocity.

I understand that this arrangement will produce both an electric field and an magnetic field. I have further found out somewhere that the electric field will not be "time-independent electrostatic out of system".

My question:

I think that at any time '$t$', the charge distribution though moving will be the same as that when it was at rest or at any other time. Hence the electric field produced should be same at any time '$t$'.

What is the flaw in my understanding?

Edit:

My source - This question was asked in IIT JEE Physics 2006 as follows:

Improve this question
$\endgroup$
4
  • 2
    $\begingroup$ Do you have a source saying that the electric field is time-dependent? I see no reason for it to be, in this case. $\endgroup$
    – Philip
    Commented Sep 7, 2020 at 5:38
  • 1
    $\begingroup$ The question (if it is 39 of the link that you've posted) seems extremely badly posed. It is not clear what the options mean (for example, what on Earth is $\Delta Q>0$, and why is it present in both Q38 and Q39?). Is this the only place you've seen it? Because if so, I wouldn't consider it to be very authoritative. Your own analysis seems fine. $\endgroup$
    – Philip
    Commented Sep 7, 2020 at 7:45
  • $\begingroup$ @Philip The link was sloppy. I have inserted the question from my book. $\endgroup$
    – Tony Stark
    Commented Sep 7, 2020 at 14:55
  • 1
    $\begingroup$ Hmm. I would suggest not paying any attention to this, frankly. It's a badly worded question in general. Clearly the right hand sides are not mutually exclusive, as you seem to have discovered yourself: options (B), (C), and (D) could all be associated with "magnetic field" option. The fact that two of them are not (because of the constraints of the "match the following" game) does not mean that magnetic fields don't exist in the other situations. Similarly, just because option (B) does not match to (p) does not mean that the field produced by the phenomenon described in (p) is time dependent! $\endgroup$
    – Philip
    Commented Sep 7, 2020 at 15:06

1 Answer 1

Reset to default
1
$\begingroup$

As stated by @Philip, both the $E$ and induced $B$ fields are time-independent if the charge density is uniform enough. However, if the charge distribution is somehow discrete, the mentioned fields are time-dependent. For instance, if you have only one point charge moving uniformly in a circular path, the fields are time-dependent everywhere in the plane of rotation except for the center about which the charge revolves. At the center of rotation, both the direction and strength of the induced $B$ field, as well as the strength of the $E$ field, are constant, whereas the direction of $E$ is changing.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.