Will the medium boundary act like a spherical mirror?

Question

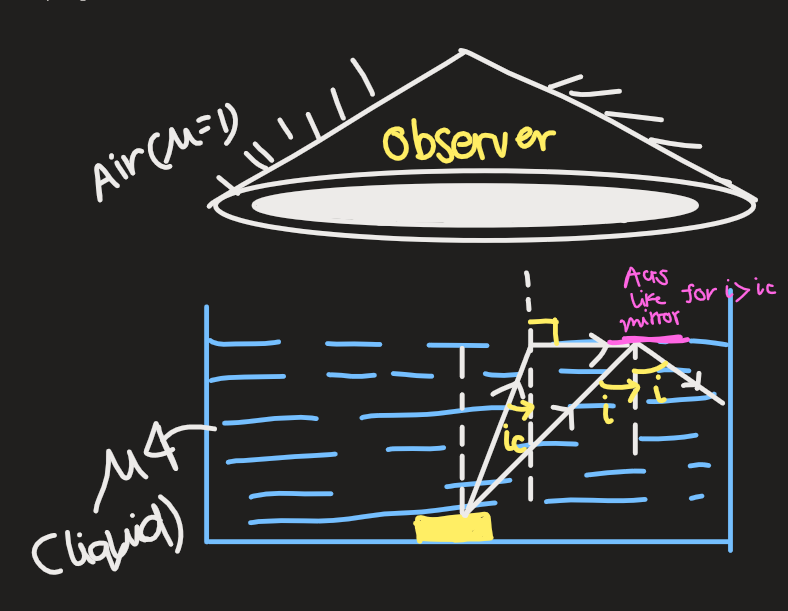
I was studying geometrical optics the other day and my teacher told me about the concept of critical angle and total internal reflection.

He had taken an example in which a beaker was filled with a liquid whose refractive index was $\mu$ and above the beaker after some gap was the eye of an observer in the air (refractive index = 1). And an object was placed at the bottom of the beaker.

NOTE: $\mu > 1$

I know that for $\angle~i>\angle~i_c$ the boundary of the medium(i.e. the liquid in this case) behaves like a plane mirror and the incident rays just reflect back into the medium.

But If we take into account the meniscus of the liquid at the surface, say some transparent liquid which has a convex meniscus, so won't the surface behave like a spherical mirror for $\angle~i>\angle~i_c$ ?

Answer 1

If the container is small enough so that the meniscus forms a well-defined curved surface then, yes, the light that is reflected backward should pick up a converging phase factor. However, if the container is large, as is usually the case in scenarios (experiments) where internal reflections is relevant, then the role of the meniscus is negligible. Then you won't notice any change in the converge or divergence of the light beam.