1
$\begingroup$

elec

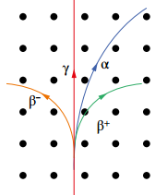
The following is the description for this figure provided by my textbook:

The paths of different types of radiation in a magnetic field. Using the right-hand slap rule, we see that positively charged particles are forced to the right. [...]

Why are the positively charged particles going to the right? I think there isn't enough information. Based on the figure, one can only deduces that magnetic field is going out from the screen or page.

It still isn't to me that why the positive charges move to the right? I do know that whatever the direction in which the positive charges move, the electrons will move directly opposite.

How can I figure out where is the direction of the lorentz force? Subsequently, how can I figure out the direction of the individual charged particle?

Improve this question
$\endgroup$
0

4 Answers 4

Reset to default
3
$\begingroup$

The hidden assumption is that the particles enter from the bottom of the diagram and are moving (initially) towards the top. That gives the direction of $\vec v$. You've correctly read the direction of $\vec B$, so you're ready to find $\vec v\times\vec B$.

Improve this answer
$\endgroup$
2
  • 3
    $\begingroup$ I wouldn't call it "hidden" since there are arrowheads there :-) $\endgroup$
    – Carl Witthoft
    Commented Aug 11, 2020 at 14:52
  • $\begingroup$ What's obvious to the expert isn't necessarily obvious to the beginner. I have a few complaints about this figure; the placement of the arrowheads is one of them. $\endgroup$
    – rob ♦
    Commented Aug 11, 2020 at 18:43
0
$\begingroup$

If I understand your question, you don't see why the positive charges go to the right and the negative charges go to the left, instead of the other way round.

The thing is, the magnetic field also has a direction. The magnetic field lines can be facing either up or down.

And that's what makes the difference. When they're facing up the positive charges go right, when they face down the positive charges go left.

If the charges came from the right, then with the magnetic field facing up the positive charges would go toward the top of the page. With the magnetic field facing down the positive charges would go to the bottom of the page.

Why is it that way? I don't know. The math describes how it happens and doesn't say why.

Is the convention that magnetic field lines face from north to south or from south to north? I don't know, I always get those confused and have to look it up if I need it. It's only a convention.

Improve this answer
$\endgroup$
1
  • $\begingroup$ Thanks for attempting to answer the question, but I already accepted the answer provided by rob. Also I believe answers need to be facts based not opinions based. $\endgroup$
    – CountDOOKU
    Commented Aug 12, 2020 at 9:08
0
$\begingroup$

The cross product right hand rule applied to the charges moving in a magnetic field is:

$$\mathbf F=q(\mathbf v\times \mathbf B)$$

where q is the charge and v and B are vectors

In a magnetic field perpendicular to the page in the image, a charged particle moving with a vector velocity v will balance the magnetic force with the centrifugal force

$$q(\mathbf v\times\mathbf B)=\frac{mv^2}{r} \hat{\mathbf r}$$

That is why the charged tracks are turning in a circular path. q, the charge being positive or negative will define how the charged track will turn. So the figure caption is missing the definition of the direction of B. see here for a definition. If I am not getting my fingers mixed up, the definition of the image requires a magnetic field out of the page.

In real experiments life is simpler one has only to look at the electrons to know which charge tracks have

proton

The little tiny circles are electrons kicked off the atoms, so one knows which way charges go without needing to bother with whether the field goes into or out of the plane.

Improve this answer
$\endgroup$
0
0
$\begingroup$

Well, the charges seem to come from the bottom of the page, going up: using the right-hand rule you place the velocity vector on the palm of your hand and close your hand towards the direction of the field, here directed upwards. And there it is, the force $\mathbf{F}\propto q(\mathbf{v}\times\mathbf{B})$ is directed as your thumb, to the right for positive charges

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.