I've been teaching myself physics through the internet and I came to a question about ballistic pendulums. Usually, I have seen these questions asked in regards to energy, but not forces. Assuming that the block has mass, immediately after a bullet strikes a mass hanging from a string (which before the collision, the two would have a net force of 0), does the tension force acting on the block outweigh the force of gravity acting on the block? If not, what causes the block to move in the upwards directions?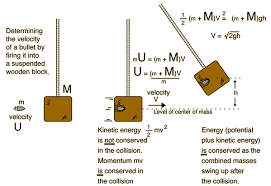 
$\begingroup$
$\endgroup$
2
-
$\begingroup$ Sorry, could you be a bit more specific? Your question seems incomplete (add a little graph or a more detailed explanation). $\endgroup$– Álvaro LuqueCommented Jun 11, 2020 at 16:08
-
$\begingroup$ Hopefully that helps, sorry for being vague. $\endgroup$– Ben YCommented Jun 11, 2020 at 19:09
Add a comment
|
3 Answers
$\begingroup$
$\endgroup$
11
With the figure everything makes more sense, thanks for editing your post. So, if you're asking why the block moves upwards, you have to think that when the bullet impacts the block, there are more forces into play than just gravity and tension. The general "definition" of force is given by Newton's $2^{nd}$ law $$\vec{F}=\frac{d\vec{p}}{dt}$$ where $\vec{p}$ is the lineal momentum. So when the bullet impacts the block, the momentum of the block changes, thus the bullet exerts a force on it with the same direction as the bullet's speed. In a situation where the block is not constrained, i.e. free on a smooth horizontal surface, as you can imagine the block just moves in the direction in which the bullet was moving, with a lower speed (because total energy is conserved and mass is higher). In here, the block is not free, but its movement is constrained by the rope. It doesn't just move upwards, but upwards and to the right, actually in a circular motion. This is because you have the tension "pulling" the block upwards (compensated by gravity) and you have a perpendicular speed to that force, which acts like a centripetal force for this system.
-
$\begingroup$ So because it is in circular motion IMMEDIATELY after the impulse is delivered, does tension outweigh gravity? Is it appropriate to say that Ft = mg + mv^2/r? $\endgroup$– Ben YCommented Jun 12, 2020 at 20:47
-
$\begingroup$ I think I'm not catching what you mean when you use outweigh. If you mean that there is a force compensation, then yes, but only in the vertical axis where there is no acceleration. The equation you used is not correct because the centripetal force is not directed totally in the direction of the weight force and the tension. It depends on an angle shown in Gert's answer. $\endgroup$ Commented Jun 12, 2020 at 20:52
-
$\begingroup$ But immediately after the impact, isn't the angle theta very close to 0 which would mean that cos(~0) = 1? Let me clarify a little more: instantly after the bullet has delivered the force, is the force of tension acting on the block greater than the force of gravity acting on the block? $\endgroup$– Ben YCommented Jun 12, 2020 at 21:02
-
$\begingroup$ Could you specifically explain why that is? It just isn't clicking yet. $\endgroup$– Ben YCommented Jun 12, 2020 at 21:05
-
$\begingroup$ I'm sorry the last coment mislead you. I was wrong. The centripetal force (which is the tension) is the same along all the movement for a rigid rope. But at no point this is greater than the corresponding gravity component. $\endgroup$ Commented Jun 12, 2020 at 21:20
$\begingroup$
$\endgroup$
2
The block is moved by the bullet striking it. Momentum and energy are conserved. After the bullet strikes the block, the only forces are gravity and tension. Assume in this problem the sting is strong enough to hold on after bullet strikes.
-
$\begingroup$ I understand this. However, the question I would like answered is whether or not tension outweighs gravity after the impulse of the bullet is delivered to the block. And also, is the block accelerating upwards at that instant or does it begin to accelerate upwards after this point? $\endgroup$– Ben YCommented Jun 12, 2020 at 20:49
-
$\begingroup$ It's pointless to look at 'that instant or just after'. 'just after' means ' in the limit for $\theta \to 0$. But that doesn't look very nice as a FBD, hence I'm looking at it a little later. $\endgroup$– GertCommented Jun 12, 2020 at 21:13
$\begingroup$
$\endgroup$
1
[...] does the tension force acting on the block outweigh the force of gravity acting on the block? If not, what causes the block to move in the upwards directions?
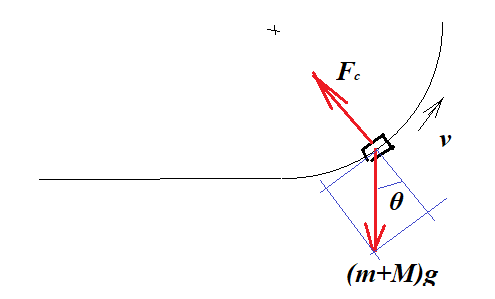
Consider the free body diagram of the pendulum plus bullet:
The pendulum plus bullet (P+b) is on a circular trajectory which requires a centripetal force $F_c$, provided by the tension in the rope:
$$F_c=\frac{(m+M)v^2}{r}=(m+M)g\cos \theta$$
$r$ is the length of the rope.
(The $\sin$ component of $(m+M)g$ provides the restoring force, ensuring the P+b will 'fall back', once all its kinetic energy has been converted to potential energy)
-
$\begingroup$ Thank you. However, this seems to be much time after the bullet struck the block. What I mean is instantly after the bullet has delivered its impulse. What does the FBD look like at that point in time? $\endgroup$– Ben YCommented Jun 12, 2020 at 20:44