How to calculate the fraction of light rays reflected?

Question

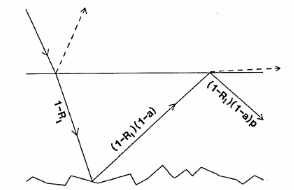
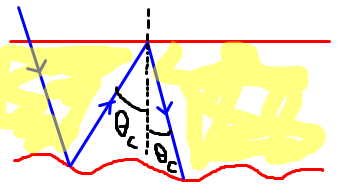
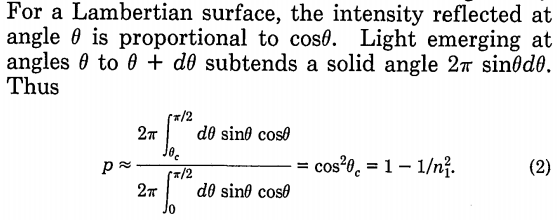The smooth surface is air-liquid interface and the rough surface is let's say a t-shirt.

The image above shows the probability of each reflection, and the $p$ value is the probability of the light rays totally reflected by the air-liquid interface. So this means $p$ represents the fraction of light rays that goes in an angle greater than the critical angle and we are after the intensity (or amount I don't really know how I should call it) of light in the yellow shaded area:

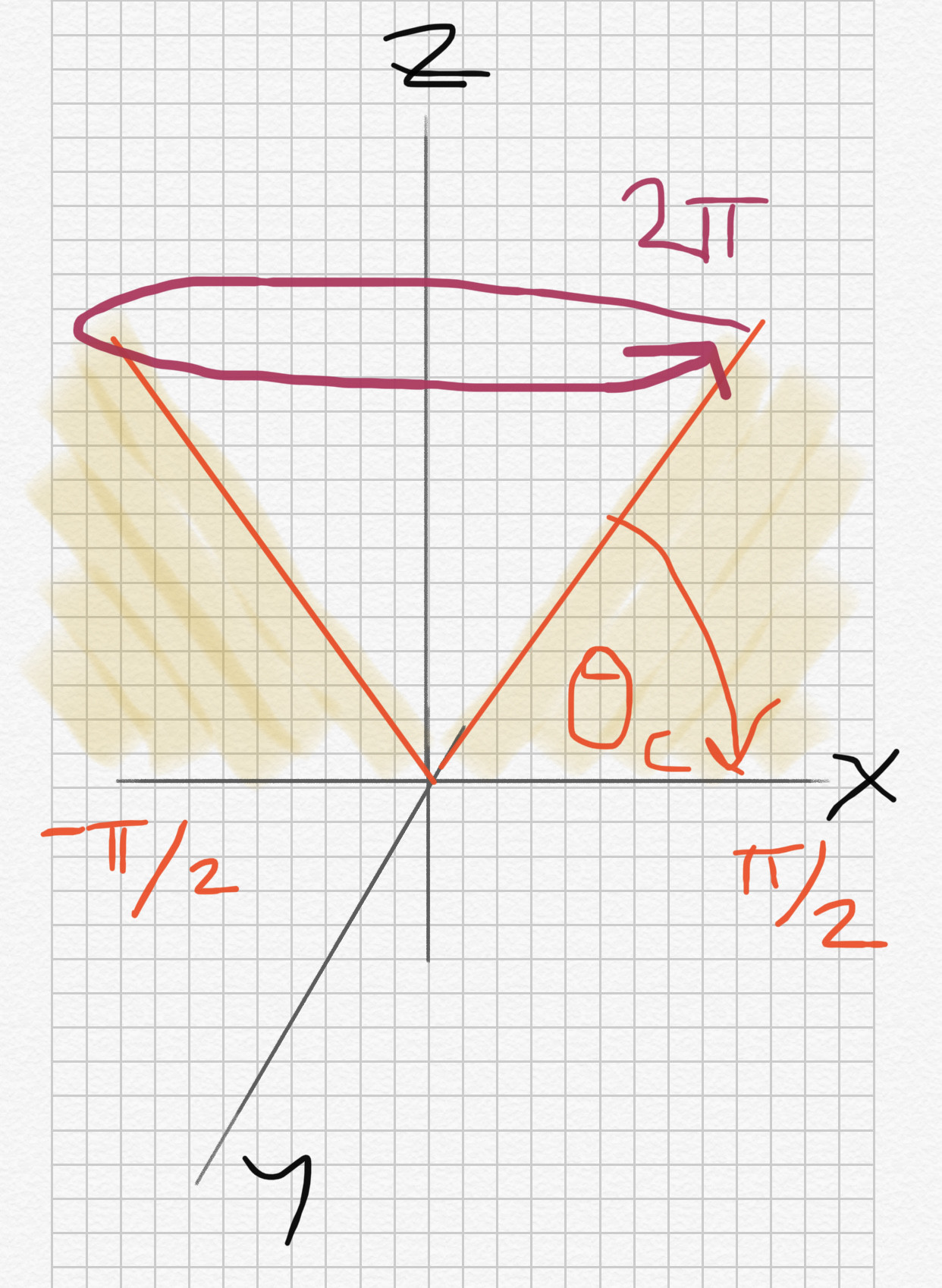
The article I am reading finds $p$ in the following way but I do not understand it. Can anyone please explain it in a way that can be clearly understood?

Answer 1

Lambertian phase function is,

$$ \Phi(\theta) = \cos(\theta) $$

This means at normal $\theta=0$ maximum intensity is reflected form the cloth surface, which falls of to zero when $\theta=\pi/2$.

Reflection from the surface is diffuse, meaning that a beam of light entering at a constant angle, will be reflected into all angles and will be distributed according to the phase function.

This requires integrating over all angles to calculate to total intensity reflected.

The integral in the numerator looks like this,

The integrals adds up all possible directions of reflected light outside the cone.

$$ \int \Phi(\theta) d\Omega $$

Element of solid angle is defined as

$$ d\Omega = \sin(\theta)d\theta d\phi $$

Substituting in and including the correct limits,

$$ \int_0^{2\pi} \int_{\theta_c}^{\pi/2} \cos(\theta)\sin(\theta) d\theta d\phi $$

We can integrate directly in $\phi$,

$$ 2\pi \int_{\theta_c}^{\pi/2} \cos(\theta)\sin(\theta) d\theta $$

This is what is written above.

The second integrals is just adding up all possible reflected directions in the hemisphere; it’s used to normalise the reflected value to give a probability.