To determine whether this 1% fraction is a reasonable estimate, we will first estimate the total noise floor for an antenna at $100\,\mathrm{MHz}$, and then compare that to the total radiation incident on such an antenna's effective area from the CMB.
We can first establish a thermal noise floor for our system via $N_T=k_BTB$ for a temperature $T$ and bandwidth $B$. Let's assume $293\,\mathrm{K}$ for our temperature, and take the size of an FM radio channel for our bandwidth - about $200\,\mathrm{kHz}$. Then we have a thermal noise floor of roughly $-120\,\mathrm{dBm}$. To this, we will need to add the effects of galactic noise, man-made noise, atmospheric noise, etc.
In 1963, the CCIR (Consultative Committee on International Radio, which would eventually become the ITU-R) published their Report 322 - World Distribution and Characteristics of Atmospheric Radio Noise (link here). This document contains estimates for the total noise floor, given in $\mathrm{dB}$ above the thermal noise floor $kTB$. It does not provide estimates at frequencies as high as $100\,\mathrm{MHz}$, but we can extrapolate that $20\,\mathrm{dB}$ above thermal could be reasonable at that frequency. Although this source is old and the ambient noise situation is likely to have changed since then (due to shifts in man-made noise, primarily), I think this would still provide us with a sensible estimate.
Fortunately, I was able to find a more recent estimate which actually includes our frequency of interest - the ITU-R's recommendation P.372-16, published in August 2022. If we ignore the Sun (their estimate is for a narrow beam pointing directly at it), then the primary contributor is man-made noise with a power of about $20\,\mathrm{dB}$ above thermal noise. This agrees with our extrapolation from the above source, so even if this number is not exactly right, it should at least provide us with a reasonable order of magnitude estimate. From these sources, we can say that the noise floor in our scenario will be in the neighborhood of $-100\,\mathrm{dBm}$.
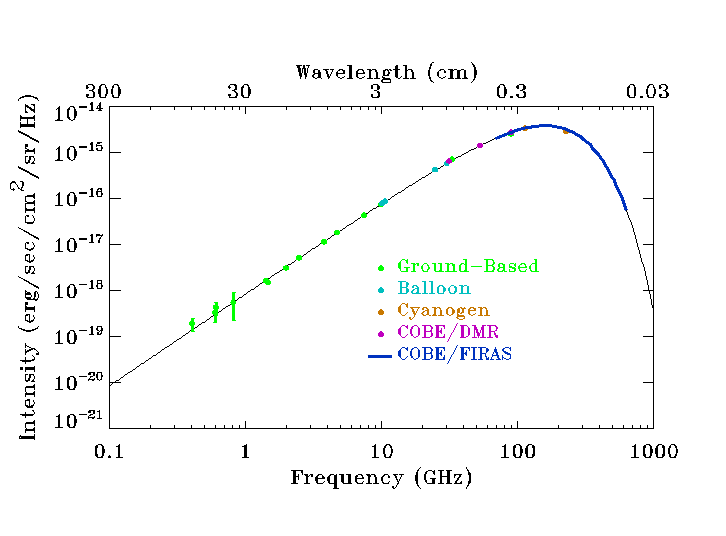
Next, we should estimate the total power that will couple into our antenna from the CMB. We can do this simply by estimating that the antenna has an "effective area" which is roughly given by the square of the wavelength of interest. The wavelength of a $100\,\mathrm{MHz}$ signal is about $3\,\mathrm{m}$, so our effective area will be of order $10\,\mathrm{m}^2$. We can use your provided spectral radiance of $I=10^{-20}\,\mathrm{erg}\,\mathrm{s}^{-1}\,\mathrm{cm}^{-2}\,\mathrm{sr}^{-1}\,\mathrm{Hz}^{-1}$ to calculate the power the CMB delivers to the antenna:
$$P_{CMB}=I\times(200\,\mathrm{kHz})\times(2\pi\,\mathrm{sr})\times(10\,\mathrm{m}^2)\approx10^{-15}\,\mathrm{W}$$
Now, let us compare to our calculated total noise floor. $-100\,\mathrm{dBm}$ is about $10^{-13}\,\mathrm{W}$, so by this estimation the CMB contributes a fraction of the noise:
$$\frac{P_{CMB}}{P_{Noise}}\approx\frac{10^{-15}}{10^{-13}}=\boxed{1\%}$$