As Wikipedia says:
[...] the kinetic energy of a non-rotating object of mass $m$ traveling at a speed $v$ is $\frac{1}{2}mv^2$.
Why does this not increase linearly with speed? Why does it take so much more energy to go from $1\ \mathrm{m/s}$ to $2\ \mathrm{m/s}$ than it does to go from $0\ \mathrm{m/s}$ to $1\ \mathrm{m/s}$?
My intuition is wrong here, please help it out!
The previous answers all restate the problem as "Work is force dot/times distance". But this is not really satisfying, because you could then ask "Why is work force dot distance?" and the mystery is the same.
The only way to answer questions like this is to rely on symmetry principles, since these are more fundamental than the laws of motion. Using Galilean invariance, the symmetry that says that the laws of physics look the same to you on a moving train, you can explain why energy must be proportional to the mass times the velocity squared.
First, you need to define kinetic energy. I will define it as follows: the kinetic energy $E(m,v)$ of a ball of clay of mass $m$ moving with velocity $v$ is the amount of calories of heat that it makes when it smacks into a wall. This definition does not make reference to any mechanical quantity, and it can be determined using thermometers. I will show that, assuming Galilean invariance, $E(v)$ must be the square of the velocity.
$E(m,v)$, if it is invariant, must be proportional to the mass, because you can smack two clay balls side by side and get twice the heating, so
$$ E(m,v) = m E(v)$$
Further, if you smack two identical clay balls of mass $m$ moving with velocity $v$ head-on into each other, both balls stop, by symmetry. The result is that each acts as a wall for the other, and you must get an amount of heating equal to $2m E(v)$.
But now look at this in a train which is moving along with one of the balls before the collision. In this frame of reference, the first ball starts out stopped, the second ball hits it at $2v$, and the two-ball stuck system ends up moving with velocity $v$.
The kinetic energy of the second ball is $mE(2v)$ at the start, and after the collision, you have $2mE(v)$ kinetic energy stored in the combined ball. But the heating generated by the collision is the same as in the earlier case. So there are now two $2mE(v)$ terms to consider: one representing the heat generated by the collision, which we saw earlier was $2mE(v)$, and the other representing the energy stored in the moving, double-mass ball, which is also $2mE(v)$. Due to conservation of energy, those two terms need to add up to the kinetic energy of the second ball before the collision:
$$ mE(2v) = 2mE(v) + 2mE(v)$$
$$ E(2v) = 4 E(v)$$
which implies that $E$ is quadratic.
Here is the non-circular version of the force-times-distance argument that everyone seems to love so much, but is never done correctly. In order to argue that energy is quadratic in velocity, it is enough to establish two things:
The result then follows.
That the energy in a constant gravitational field is proportional to the height is established by statics. If you believe the law of the lever, an object will be in equilibrium with another object on a lever when the distances are inversely proportional to the masses (there are simple geometric demonstrations of this that require nothing more than the fact that equal mass objects balance at equal center-of-mass distances). Then if you tilt the lever a little bit, the mass-times-height gained by 1 is equal to the mass-times-height gained by the other. This allows you to lift objects and lower them with very little effort, so long as the mass-times-height added over all the objects is constant before and after.This is Archimedes' principle.
Another way of saying the same thing uses an elevator, consisting of two platforms connected by a chain through a pulley, so that when one goes up, the other goes down. You can lift an object up, if you lower an equal amount of mass down the same amount. You can lift two objects a certain distance in two steps, if you drop an object twice as far.
This establishes that for all reversible motions of the elevator, the ones that do not require you to do any work (in both the colloquial sense and the physics sense--- the two notions coincide here), the mass-times-height summed over all the objects is conserved. The "energy" can now be defined as that quantity of motion which is conserved when these objects are allowed to move with a non-infinitesimal velocity. This is Feynman's version of Archimedes.
So the mass-times-height is a measure of the effort required to lift something, and it is a conserved quantity in statics. This quantity should be conserved even if there is dynamics in intermediate stages. By this I mean that if you let two weights drop while suspended on a string, let them do an elastic collision, and catch the two objects when they stop moving again, you did no work. The objects should then go up to the same total mass-times-height.
This is the original demonstration of the laws of elastic collisions by Christian Huygens, who argued that if you drop two masses on pendulums, and let them collide, their center of mass has to go up to the same height, if you catch the balls at their maximum point. From this, Huygens generalized the law of conservation of potential energy implicit in Archimedes to derive the law of conservation of square-velocity in elastic collisions. His principle that the center of mass cannot be raised by dynamic collisions is the first statement of conservation of energy.
For completeness, the fact that an object accelerates in a constant gravitational field with uniform acceleration is a consequence of Galilean invariance, and the assumption that a gravitational field is frame invariant to uniform motions up and down with a steady velocity. Once you know that motion in constant gravity is constant acceleration, you know that
$$ mv^2/2 + mgh = C $$
so that Huygens dynamical quantity which is additively conserved along with Archimedes mass times height is the velocity squared.
The question is especially relevant from a didactical point of view because one has to learn to distingish between energy (work) and momentum (quantity of motion).
The kinematic property that is proportional to $v$ is nowadays called momentum, it is the "quantity of motion" residing in a moving object, its definition is $p:= mv$.
The change of momentum is proportional to the impulse: impulse is the product of a force $F$ and the timespan $\Delta t$ it is applied. This relation is also known as the second law of Newton: $F \Delta t = \Delta p$ or $F dt = dp$. When one substitutes $mv$ for $p$ one gets its more common form: $F= m \frac{\Delta v}{\Delta t} = ma$.
Now for an intuitive explanation that an object with double velocity has four times as much kinetic energy.
Say A has velocity $v$ and B is an identical object with velocity $2v$.
B has a double quantity of motion (momentum) - that's were your intuition is correct!
Now we apply a constant force $F$ to slow both objects down to standstill. From $F \Delta t = \Delta p$ it follows that the time $\Delta t$ needed for B to slow down is twice as much (we apply the same force to A and B). Therefore the braking distance of B will be a factor of 4 bigger then the braking distance of A (its starting velocity, and therefore also its mean velocity, being twice as much, and its time $\Delta t$ being twice as much, so the distance, $s = \bar{v}\Delta t$, increases 2 x 2 = 4 times).
The work $W$ needed to slow down A and B is calculated as the product of the force and the braking distance $W=Fs$, so this is also four times as much. The kinetic energy is defined as this amount of work, so there we are.
Let me just throw in an intuitive explanation. You could re-phrase your question as:
Why does velocity only increase as the square root of kinetic energy, not linearly?
Well, drop a ball from a height of 1 meter, and it has velocity v when it hits the ground.
Now, drop it from a height of 2 meters. Will it have a velocity of 2v when it hits the ground?
No, because it travels the second meter in a lot less time (because it's already moving), so it has less time to gain speed.
The only real physical reason (which is not really a fully satisfying answer) is that $E \sim v^2$ is what experiments tell us. For example, gravitational potential energy on the Earth's surface is proportional to height, and if you drop an object, you can measure that the height it falls is proportional to the square of its speed. Thus, if energy is to be conserved, the kinetic energy has to be proportional to $v^2$.
Of course, you could question why gravitational potential energy is proportional to height, and once that was resolved, question why some other kind of energy is proportional to something else, and so on. At some point it becomes a philosophical question. The bottom line is, defining kinetic energy to be proportional to the square of the speed has turned out to make a useful theory. That's why we do it.
On the other hand, you could always say that if it were linear in velocity, it would be called momentum ;-)
P.S. It may be worth mentioning that kinetic energy is not exactly proportional to $v^2$. Special relativity gives us the following formula:
$K = mc^2\left(1/\sqrt{1 - v^2/c^2} - 1\right)$
For low speeds, this is essentially equal to $mv^2/2$.
Just to post another, more mathematical, version of this that is not dependent on thermodynamics, but rather just vector calculus and Newton's laws, let's consider Newton's second law:
$$\sum {\vec F} = m{\vec a}$$
Now, apply the definition of work, $W = \int d{\vec s} \cdot{\vec F}$
We have, assuming that $s$ is the actual path traveled by the particle, and using some clever changes of variables:
$$\begin{align} \sum W &= m\int d{\vec s(t)}\cdot {\vec a}\\ &=m\int dt\frac{d{\vec s}}{dt}\cdot {\vec a}\\ &= m\int dt \,{\vec v} \cdot {\vec a}\\ &= m\int dt\,{\vec v}\cdot \frac{d{\vec v}}{dt}\\ &= m\int {\vec v} \cdot d{\vec v}\\ &= \frac{1}{2}m\left(v_{f}^{2} - v_{i}^{2}\right)\\ &= \Delta {\rm KE} \end{align}$$
So, we see that the definition of work is synonymous with quadratic dependence on the velocity. Who cares? Well, now, we fix some requirements on the force. Namely, we assume our forces are conservative. What does this mean? Well, it means that our force is curl free $\rightarrow {\vec \nabla} \times {\vec F}=0$. This is mathematically equivalent to many things, but the most important two are that $\int d{\vec s}\cdot {\vec F}$ doesn't depend on the path you integrate over, but only the endpoints of the curve, and second, that ${\vec F} = -{\vec \nabla}\phi$ for some function $\phi(x,y,z,t)$. Once you know this, it's relatively easy to show that $\int {\vec ds}\cdot {\vec F} = \phi_{0} - \phi_{f}$
Then, you have:
$$0 = \Delta {\rm KE} + \sum \Delta {\rm PE}_{i}$$
where the sum is over the potentials for the various forces (and I deviously substituted PE for $\phi$, since we are obviously talking about potential energy now.) We have now proved that the total energy does not change. Therefore, the standard definition of work gives us a conserved quantity, which we can call the energy (so long as we assume the absence of nonconservative forces, but in the presence of these, energy is not conserved, and we start having to worry about losses to heat and radiation).
As Piotr suggested, accepting the definition of work $W=\mathbf{F}\cdot d\mathbf{x}$, it follows that the kinetic energy increases quadratically. Why? Because the force and the infinitesimal interval depend linearly on the velocity. Therefore, it is natural to think that if you multiply both quantities, you need to end up with something like $K v^{2}$, where $K$ is an 'arbitrary' constant.
A much more interesting question is why the Lagrangian depends on the velocity squared. Given the homogeneity of space, it can not contain explicitly $\mathbf{r}$ and given the homogeneity of time, it can not depend on the time. Also, since space is isotropic, the Lagrangian can not contain the velocity $\mathbf{v}$. Therefore, the next simplest choice should be that the Lagrangian must contain the velocity squared. I do think that the Lagrangian is more fundamental in nature than the other quantities, however, its derivation involves the definition of work or equivalently, energy. So probably you won't buy the idea that this last explanation is the true cause of having the kinetic energy increasing quadratically, although, I think it is much more satisfactory than the first explanation.
In comes down to definitions.
Momentum is defined as $p = mv$. Momentum grows linearly with velocity making momentum a quantity that is intuitive to understand (the more momentum the harder an object is to stop). Kinetic energy is a less intuitive quantity associated with an object in motion. KE is assigned such that the instantaneous change in the KE yields the momentum of that object at any given time:
$\frac{dKE}{dv} = p$
A separate question one might ask is why do we care about this quantity? The answer is that in a system with no friction, the sum of the kinetic and potential energies of an object is conserved:
$\frac{d(KE + PE)}{dt} = 0 $
For every relatively equal (in percents) increase of the speed, the applied force must be present over increasingly (quadratically) long travel distance. F=m*a. At the same time force*distance=work, where work=energy.
I think it follows from the first law of Thermodynamics. It turns your definition of work into a conserved property called energy. If you define work in the $Fdx$ style (as James Joule did) then the quadratic expression for kinetic energy will follow with the symmetry arguments.
In his excellent answer, Ron Maimon cleverly suggests using heat to avoid a reference to work. To determine the number of calories he uses a thermometer. A perfect thermometer will measure $\partial{E}/\partial{S}$ so when he's done defining entropy, he still needs a non-mechanical definition of work. (In fact, I believe it is Joule's contribution to show that the calorie is a superfluous measure of energy.) The weakness in Ron's answer is that he also needs the second law of thermodynamics to answer the question.
To see this explicitly, write the first law in terms of the Gibbs equation: $$ dE = TdS + vdp + Fdx $$ This equation defines $v = \partial{E}/\partial{p}$. For a conservative system set $dE=0$ and to follow Huygens, set $dS=0$ to get $vdp = - Fdx$ and to follow Maimon we set $dx=0$ to get $vdp = -TdS$. These are two ways of measuring kinetic energy.
Now to integrate. Huygens assumes $p$ is only a function of $v$. For small changes in $v$ we make the linear approximation $p = mv$, where $m \equiv dp/dv$. Plug that in, integrate, and you get the quadratic dependence. In fact, it's not too hard to see that if you use gravity for the force that $F = mg$ which leads to $$ \frac{1}{2} m v^2 + mgh = C . $$ Raimon also has to assume the independence of $p$ on $S$. To integrate he will have to evaluate $T$ as a function of $S$ (and possibly $p$) or use the heat capacity.
Now notice that we required the changes in $v$ to be small. In fact, kinetic energy is not always proportional to $v^2$. If you go close to the speed of light the whole thing breaks down and for light itself there is no mass, but photons do have kinetic energy equal to $c p$ where $c$ is the speed of light. Therefore, it's better to think of kinetic energy as $$ E_{kin} = \int v dp $$ and just carry out the integration to find the true dependence on $v$.
So, in summary, I suggest the "why" of the question is the same as the "why" of the first law.
The general form of the kinetic energy includes higher order corrections due to relativity. The quadratic term is only a Newtonian approximation valid when velocities are low in comparison to the speed of light c.
There is another fundamental reason for which kinetic energy cannot depend linearly with the velocity. Kinetic energy is a scalar, velocity is a vector. Moreover, if the dependency was linear this would mean that the kinetic energy would vary by substituting $\mathbf{v}$ by $-\mathbf{v}$. I.e. the kinetic energy would depend of the orientation, which again makes no sense. The Newtonian quadratic dependence and the relativistic corrections $v^4$, $v^6$... satisfy both requirements: kinetic energy is a scalar and invariant to substituting $\mathbf{v}$ by $-\mathbf{v}$.
Kinetic energy is defined as $\frac{1}{2}mv^2$ (in classical mechanics at least).
When the motion of an object is subjected to a physical law that is constant through time (for instance $\ddot{r}=-\frac{GM}{r^2}$ where GM is a constant), then when you integrate both sides with respect to distance and multiply by the mass $m$ of the object you get:
$$\frac{1}{2}mv_2^2 - \frac{GMm}{r_2} = \frac{1}{2}mv_1^2 - \frac{GMm}{r_1}$$
Assuming that the law is constant through time, then between the initial and final states the object's quantity $\frac{1}{2}mv^2 - \frac{GMm}{r}$ is conserved through time as well.
If instead of $-\frac{GM}{r^2}$ the physical law is some other function $f(r)$ constant through time, then the object's quantity $\frac{1}{2}mv^2 - F(r)$ where F is a primitive of f is conserved through time as well.
That quantity is called energy. Then we give a name to the two terms: the term that depends on velocity ($\frac{1}{2}mv^2$) is referred to as kinetic energy, and the term that depends on distance ($-F(r)$) is referred to as potential energy.
It is useful to define these quantities, because if we assume that the acceleration of an object is a function of distance constant through time (as is the case with the law of gravitation, Coulomb's law, Hooke's law, ...), and if we know the value of $F(r)$ and the value of the velocity at a given distance $r_1$ (which are both derived from measurements), then we can deduce directly the velocity of the object at any other distance without having to calculate the integral of $f(r)$ every time.
Since kinetic energy is a defined quantity it is meaningless to ask why it increases quadratically with velocity, it does because it is defined that way. The above argument gives a reason as to why it is defined that way.
Why does it take so much more energy to go from 1 m/s to 2 m/s than it does to go from 0 m/s to 1 m/s?
It isn't harder to accelerate something from 1 m/s to 2 m/s than from 0 m/s to 1 m/s, at a constant acceleration it takes the same time, however it takes 3 times more distance (so it takes 4 times more distance to accelerate from 0 m/s to 2 m/s than from 0 m/s to 1 m/s).
Let's say you accelerate your object at some constant rate so that it takes a time $\tau$ to go from 0 m/s to 1 m/s. Then it will take the same time $\tau$ to go from 1 m/s to 2 m/s.
Its velocity as a function of time will be $v(t) = \frac{1}{\tau}t$. In particular, $v(\tau) = 1$ and $v(2\tau) = 2$. Its distance traveled as a function of time will be $d(t) = \frac{1}{2\tau}t^2$
It takes a distance $d(\tau) = \frac{\tau}{2}$ to accelerate it from 0 m/s to 1 m/s, while it takes a distance $d(2\tau) = 2\tau$ to accelerate it from 0 m/s to 2 m/s.
As you can see, $d(2\tau) = 4d(\tau)$. At no point do you need to invoke kinetic energy to explain this observation, it takes 4 times more distance because the object is moving faster between $\tau$ and $2\tau$ than between $0$ and $\tau$. Similarly, at a constant deceleration rate it takes 4 times more distance to brake to a stop at velocity $2v$ than at velocity $v$, not because kinetic energy makes it somehow harder to brake when we are going faster, but simply because it takes twice longer to brake (the time to go from $2v$ to $v$ is the same as the time to go from $v$ to $0$), and because we are moving faster than $v$ (hence covering more distance) during half the braking time.
Basically, momentum is related to force times time, and KE is related to force times distance. It is all a metter of frame of reference, either time or distance. The relationship between time and distance for a starting velocity of zero is $d = \frac{at^2}{2}= \frac{tV}{2}$. Plug this into the the equations you get the KE$ = \frac{pV}{2} = \frac{p^2}{2m}$
Woolah - magic!
The quadratic variation of kinetic energy with velocity can be explained by the symmetry properties of space and time. The lagrangian function is defined as $\mathcal{L}=T-U$, where $T$ is the kinetic energy and $U$ is the potential energy.
We know that space is homogeneous and isotropic, and time is homogeneous. For a free particle, it follows that the lagrangian $\mathcal{L}$ should have the following properties:
So the general form of the lagrangian for a free particle is $$\mathcal{L}(x,v,t)=\alpha v^n$$ where $\alpha$ is a constant independent of the coordinates, velocities and time. Now, the momentum can be calculated by using the relation $$p=\frac{\partial\mathcal{L}}{\partial v}=\alpha nv^{n-1}$$ However, momentum is always a linear function of velocity which can easily be proved by dimensional analysis. This is possible only when $n=2$ in the above expression.
Since we are considering a free particle (which has only kinetic energy), the lagrangian (choosing $n=2$) is $$\mathcal{L}=T=\alpha v^2$$ Thus, the kinetic energy is proportional to $v^2$ and not any other power of $v$.
The purpose of this answer is to build an intuition for what kinetic energy is. The intended audience of this narrative is beginners who are getting their first introduction to the concept of kinetic energy.
(The narrative may come across as too slow. The idea is: this is how I would introduce this subject to a inquisitive smart youngster.)
As a basis I will first discuss our common sense notions of force and motion.
In our daily lives we are dealing with roughly three types of forces, which I will refer to as 'gravity', 'elastic forces', and 'friction forces'.
Friction
At slow velocity friction tends to be proportional to velocity. There is the trope of a bartender sliding a glass over to a guest. As we know: that's doable; you can feel how much of a push to give so that the glass comes to a standstill within arms reach of the customer.
Elasticity
As we know, elastic force tends to be proportional to how much deformation is caused.
Gravity
Gravity is always present, gravity is never not there. Our experience with gravity is so internalized that we tend to be unaware of how unique the properties of gravity are.
Unlike friction the effect of gravity is independent of your current velocity. Whatever your current velocity is, your change of velocity is the same.
Unlike elasticity the effect of gravity is independent of your current position. (Daily life is at the Earth's surface, where to all intents and purposes gravity is uniform.)
In order to focus on what kinetic energy is I use a force that allows F=ma to act in the purest possible form: a force that is independent of current velocity, and independent of position: Gravity.
I allow an object to be dropped down from a height of 16 meters.
(I choose 16 units instead of 4 because 4 has an ambiguity. The sum of 2 and 2 and the product of 2 and 2 are both 4. 16 avoids that.)
To make the numbers simple I set the force to cause an acceleration of 2 $m/s^2$
As we know: the resulting trajectory arises from the property of Nature that relates Force and Acceleration: $F=ma$
The velocity increases linear with time: after 2 seconds the object has a velocity of 4 m/s, after 4 seconds the object has a velocity of 8 m/s
The distance traveled increases quadratic with time: after 2 seconds the object has traveled 4 meters of distance, after 4 seconds the object has traveled 16 meters of distance.
Throwing stuff upwards
As we know, acceleration and deceleration are in a sense mirror images of each other, mirrored with respect to the direction of time.
Using the same rate of change of velocity: 2 $m/s^2$
If the object is thrown upwards with a starting velocity of 4 meters per second it will reach a height of 4 meters.
If the object is thrown upwards with a starting velocity of 8 meters per second it will reach a height of 16 meters.
So: when thrown upwards at double the velocity the height reached increases quadratically.
In our daily lives we experience elastic forces and friction forces all the time, and consequently we tend to expect that when you throw something with twice the velocity it will have twice as much punch to it. But the amount of "punch" that the object has goes up with the square of the velocity.
F=ma
The quadratic relation originates from the fact that acceleration is the second derivative of position. There is a fundamental relation between the operation of taking a second derivative and the operation of squaring.
If your starting velocity is 8 m/s and you decelerate at 2 $m/s^2$:
From t=0 to t=2 you travel 3/4th of the total stopping distance.
From t=2 to t=4 you travel the remaining 1/4th of the total stopping distance.
This ratio of 3/4th to 1/4th generalizes to any uniform acceleration/deceleration.
Collision of cars
In car collision the amount of damage rapidly escalates with increasing velocity.
The reason for that is the nature of deceleration.
During the first half of the deceleration duration the distance traveled is 3/4th of the total stopping distance. Crucially, the amount of damage done is proportional to the distance traveled, not proportional to the duration of the deceleration.
Kinetic energy
Kinetic energy expresses the amount of damage that is inflicted when there is a destructive collision.
Work-Energy theorem
The amount of change of kinetic energy as a consequence of force acting over distance is given by the Work-Energy theorem.
I think part of the difficulty is the issue of defining energy or at least characterizing something about energy. More broadly, I think it is worth considering why we care about energy to begin with. I will present two intuitive arguments that lead us to conclude the proportion $KE\propto v^{2}$. For these arguments, I will explicitly write out what intuitive features of energy I am invoking that I think many people would accept as reasonable.
The most important key feature of energy that I invoke throughout these arguments is that energy is something that can be converted from kinetic energy to potential energy and vice-versa, and you can store potential energy. I would argue this is precisely the reason we care about energy to begin with.
The key facts about energy is that it is "conserved" and it can be "converted" between various forms of energy between wildly disparate systems. Based on these facts, we can reasonably say the following:
Additionally, I think it's worth pointing out some more assumptions that I will use:
I haven't explicitly defined kinetic energy here, but I gave a useful characterization of some features of it. There are many other assumptions that go into my argument, but I won't make them explicit for the sake of brevity. It would be an interesting exercise to see how many assumptions I haven't stated explicitly.
Scenario: Suppose we have two boxes, each of mass $m$, moving at speed $v$ to the right with a compressed spring in-between (or anything else that could potentially separate the two boxes). This system has total energy $KE(2m, v) + U$ where $KE(\cdot, \cdot)$ is the kinetic energy and $U$ is the potential energy of the spring.
Now imagine we let go of the spring (maybe we cut the rope holding the two boxes together), and suppose the two boxes are pushed apart from one another in just the right way that the left box comes to a standstill. By conservation of momentum, the right box must be boosted to be moving at speed $2v$ to the right.
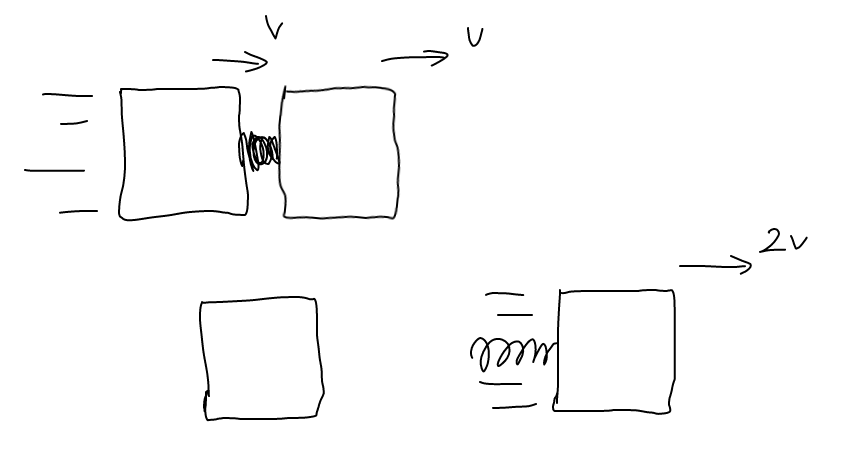
From this we get $$ KE(2m, v) + U = KE(m, 2v). $$ By assuming energy is additive with respect to mass, we get $$ 2KE(m, v) + U = KE(m, 2v). $$ By this alone, we see that energy cannot be linearly proportional to the speed $v$, because $U > 0$ here.
To get the exact proportionality relationship between energy and velocity, I will consider Galilean invariance just like in Ron Maimon's post. Given the current coordinate system, consider a new coordinate system moving to the right at velocity $v$ relative to the old one. By going to the new reference frame, the two boxes are initially at rest (so the kinetic energy is $KE(2m, 0) = 0$) and the spring is compressed with potential energy $U$. After release, both boxes move in opposite directions at speed $v$.
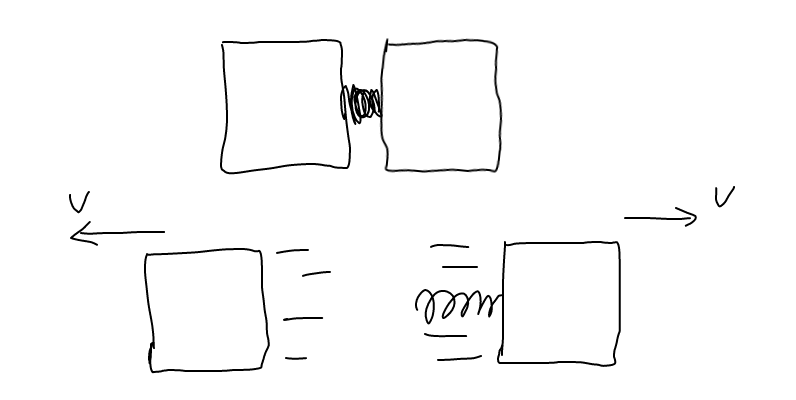
In this case, we find $$ U = 2KE(m, v). $$ Assuming potential energy is Galilean invariant, we can plug this into the previous equations, and we get $$ KE(m, 2v) = 4KE(m, v). $$ This demonstrates a quadrupling of kinetic energy with respect to a doubling of velocity. By answers in this MSE post, the general $KE\propto v^{2}$ proportion follows.
Many arguments for $KE\propto v^{2}$ invoke gravitational potential energy $mgh$, but that further leads us to ask, why is gravitational potential energy given by $mgh$ as opposed to some other expression? A further justification would invoke work $dW = F(x)\,dx$, but then we would be tempted to ask, why would we be interested in defining work?
I will present an argument without invoking work or even gravitational potential energy. First, I need to establish some reasonable features of energy:
I will use extensively the kinematics of objects in a constant gravitational field. You should keep in mind that if an object starts with zero velocity, the relationships between height dropped in free- fall and its velocity are $$ v = \sqrt{2gh} \qquad\text{ and }\qquad h = \frac{v^{2}}{2g}. $$
Scenario: Suppose we are in a (approximately) constant gravitational field like on Earth. Take an object at height $h$ relative to some ground level, and let $v = \sqrt{2gh}$.
Let the object fall $h/4$ distance, catch the object, and absorb its kinetic energy into potential energy of some device. The device has energy $KE(v/2)$. Repeat this three times so that the object ends up at ground level. The device now has energy $$ U_{1} = 4\cdot KE(v/2). $$
Now use the potential energy of the device to launch the object back up. The object cannot travel to a height greater than $h$, or else we would violate energy conservation (we could repeat our cycle to get to progressively higher and higher heights). Thus, it must be launched with some velocity no greater than $v$. Hence our device has potential energy $U_{1}$ no greater than $KE(v)$, so $$ U_{1} = 4\cdot KE(v/2) \le KE(v). $$
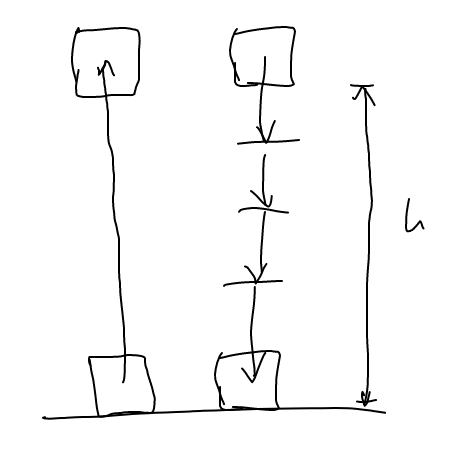
Now consider the following. Suppose we again take an object at height $h$ relative to some ground level. Let the object fall $h$ distance to the ground, catch the object, and absorb its kinetic energy into potential energy of some device. The device has energy $$ U_{2} = KE(v). $$
Now use $1/4$th of potential energy of the device to launch the object up, and repeat this three more times. Again, we cannot end up at a height greater than $h$, so, assuming each of the four upwards kicks are identical, each of the kicks must provide an upward velocity less than or equal to $v/2$. Thus, each of the kicks should be worth potential energy $U_{2}/4\le KE(v/2)$, so $$ U_{2} = KE(v) \le 4\cdot KE(v/2). $$
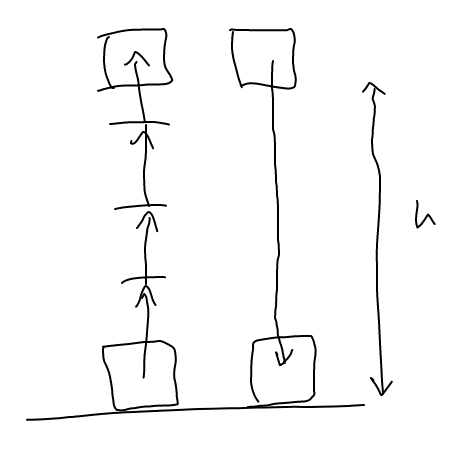
By the above inequalities, we are forced to conclude $$ KE(v) = 4\cdot KE(v/2). $$ This is equivalent to $KE(2v) = 4KE(v)$, and by answers in this MSE post, the general $KE\propto v^{2}$ proportion follows.
I have a quantitative answer which is a thought experiment avoiding all but the simplest equations.
An object going from velocity $v=0$ to $v=1$ needs to be pushed or pulled in some way. In my explanation I will use the same method to push the object from $v=0$ to $v=1$, then from $v=1$ to $v=2$, then from $v=2$ to $v=3$, etc. I will show how the energy of movement embodied in the object goes up from $0$ to $1$ to $4$ to $9$, etc.
Start with two identical balls, $m_1$ and $m_2$. Between the two balls there is a spring, $s_1$, which is held in compression. Assume the mass of the spring is very small. The potential energy in the spring is $\text{PE}=2$ and all $3$ actors have velocity $v=0$.
A. $v=0$. All objects have $0$ velocity, so kinetic energy $\text{KE}=0$.
B. $v=1$. Release the spring and $m_1$ shoots off to the left with velocity $v=1$. $m_2$ goes in the opposite direction with $v=-1$. The kinetic energy of both balls is the same and it is $\text{KE}=1$ because all of the potential energy of the spring has been transferred symmetrically to the balls.
C. $v=2$. Now place another identical ball, $m_3$, just to the right of $m_1$, and also travelling at $v=1$ and with a compressed spring, $s_2$, between them. Nothing has changed about $m_1$, it is still happily travelling at $v=1$. So what's the total energy of the $m_1, \ s_2$ and $m_3$ system? It's $1+2+1=4$ being $m_1$'s $\text{KE}$, $s_2$'s $\text{KE}$ and $m_3$'s $\text{KE}$.
Now release the spring, $m_1$ shoots off to the left with $v=2$ and $m_3$'s velocity goes from $v=1$ to $v=0$ making its $\text{KE}=0$. Because we've said that the mass of the spring is very small so its $\text{KE}$ is almost zero, then all of the energy which was in the system before the spring was released is now in $m_1$. So the $\text{KE}$ of $m_1$ is $\text{KE}=4$. Phew, $\text{KE}$ is proportional to $v$ squared!
D. $v=3$. Simply repeat the process to make $m_1$ go from $v=2$ to $v=3$ by pushing off another identical ball, $m_4$. First, work out the total energy of the two ball and spring system before the spring is released. It's $4+2+4=10$. After the spring is released $m_4$ has $v=1$, which we've established is equivalent to $\text{KE}=1$. So $m_1$ has the remaining energy of the system, which is $\text{KE}=9$.
E. $v=4$. Repeat the process. Energy of system before the spring is released is $9+2+9=20$. $\text{KE}$ of $m_1$ after spring is released is $\text{KE}=20-4=16$.
I'm not happy with assuming away the mass of the spring, so a neater explanation has a spring attached to each ball and the balls interacting via their springs, which are in contact.
Throw 3 balls up with equal weight with no air friction.
The ball have up velocity
$$V_{1}$$ $$V_{2}$$ $$V_{3}$$
And let's for simplicity sake we have $$V_{2}=2V_{1}$$ $$V_{3}=3V_{1}$$
Let's for simplicity sake ball 1 travels $$S$$
And stop after time
$$T$$
How far will ball 2 travel?
Notice that $$V_{2}=2V_{1}$$
Also deceleration is constant.
So ball 2 will requires
$$2T$$ to deplete all it's velocity.
However the average speed of ball 2 will also double. So ball 2 will travel 4 times higher.
For the same reason, ball 3 will require
$$3T$$
And the average speed will also be triple.
So ball 3 will go up 9 times higher.
So amount of kinetic energy of the balls will be quadratic amount of the velocity.
In fact, we can measure it right now.
Total potential energy will be
$$mgh$$
The average up velocity will be
$$V_{average}=\frac{1}{2}{V_{0}}$$
Here, $$V_{0}$$ is the initial velocity.
So $$h$$ will simply be time to stop times average speed. Time to stop is simply
$$T=\frac{V_{0}}{g}$$
So, $$h=T V_{average}$$
$$=\frac{V_{0}}{g} \frac{1}{2}{V_{0}}$$
Total kinetic energy of ball moving upward with speed $$V_{0}$$
will simply be the potential energy of the ball when it stops.
So it is $$E_{potential when stop}=E_{kinetic energy at start}$$ $$mgh=m\frac{V_{0}}{g} \frac{1}{2}{V_{0}}$$
$$mgh=\frac{1}{2}m{V_{0}^{2}}$$
One way to look at this question of yours is as follows:
$$ E(v) = \frac{m v^2}{2} \; . $$
So, if we multiply the velocity by a certain quantity, i.e., if we scale the velocity, we get the following,
$$ E(\lambda v) = \frac{m (\lambda v)^2}{2} = \lambda^2 \frac{m v^2}{2} = \lambda^2 E(v)\; . $$
That is, if you scale your velocity by a factor of $\lambda$, your Energy is scaled by a factor of $\lambda^2$ — this should answer your question (just plug in the numbers).
