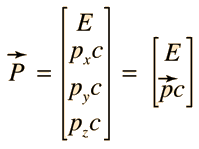Clarification regarding the mass-energy equivalence

Question

In this recent question:

As neutrons are more massive than protons does Sun increase mass while fusioning elements?

It is stated that not only does the Sun not gain mass, but it loses mass at 4 million tons (or tonnes) per second (plus 1.5 tons per second due to the solar wind).

My understanding of the extended version of the mass-energy equivalence equation:

$$ E=\sqrt{(mc^2)^2+(pc)^2} $$

is that it is not a conversion formula, but an equivalence formula: https://en.wikipedia.org/wiki/Mass–energy_equivalence#Meanings_of_the_strict_formula.

In this case, if I imagine an empty universe with the Sun in it. The Sun's invariant mass is being reduced by 4 million tons per second (plus 1.5 tons for the solar wind). But, my understanding is that mass is NOT being converted into energy, but it does seem like the total mass of the system is being reduced.

Some of the mass reduction is carried on the solar wind as actual mass, but most (4/5.5) is carried away by the photons as momentum.

So, the energy of the universe system is conserved, but the mass is not conserved? The scalar momentum seems not to be conserved, but perhaps the vector momentum is conserved?

So, while the equivalence principle is not a conversion formula for mass and energy, could it be considered a relation governing the conversion between mass and photons? Any clarification on any and all of this is appreciated.

Answer 1

In nuclear physics one is in the quantum mechanical regime which is strictly described with four vectors.In this case energy momentum fourvectors,

$$\sqrt {P \cdot P} = \sqrt {E^2-(pc) ^2}= m_0c^2$$

These have a "length" the invariant mass, where E is the total energy of the system, and the second term can be considered the kinetic energy, since if p is zero the system is at rest.

If they are describing an elementary particle, the mass describes the particle. If they are describing a sum of elementary particles, the mass is the invariant mass of the system, and it depends both on the kinetic energy and the momentum vectors describing the system of particles. Because of the kinetic energy the invariant mass of a system of elementary particles is much larger than the sum of the masses.

In nuclear physics, the nucleons, protons and neutrons, have a fixed invariant mass, but the nuclei , because they are the sum of the fourvectors of the nucleons they are composed of, have an invariant mass characterizing them, larger than the sum of the constituents. That is what leads to the binding energy curve:

which allows fusion to happen below the iron nuclei, i.e. energy to be released in radiation and kinetic energy, and fission for higher, because the break up again can release energy.

Four vectors and the equivalence principle are two different frameworks.

As for the mass reduction of the sun, it should be clear that since the invariant masses of the constituents become smaller, and a large amount of particles and radiation leaves the sun, the addition of the four vectors of all the remaining involved constituents will be getting smaller as time goes on; this should be evident by simple vectorial additions.