What will be the mass of the sun when the core is depleted of hydrogen about 5 billion years from now?

Question

Our sun converts 600 million tons of H to He every second, that is 5 million tons of matter into energy through nuclear fission. However, as the core of the sun continues to shrink the outer layers of the sun move closer and experience a stronger gravitational force. This causes more pressure on the core, which is resulted by increases in the rate at which fusion occurs. Hence the rate at which our sun losses its mass is not continuous. I want to know the mass of our sun when the core is depleted of hydrogen about 5 billion years from now (Mass loss as a result of nuclear fusion only an not due to stellar wind). I also want to know its composition, at present 73% of our sun's mass is Hydrogen and 25% is He.

Answer 1

The Sun's mass will be approximately $0.9994M_{\odot}$ at the end of the main sequence.

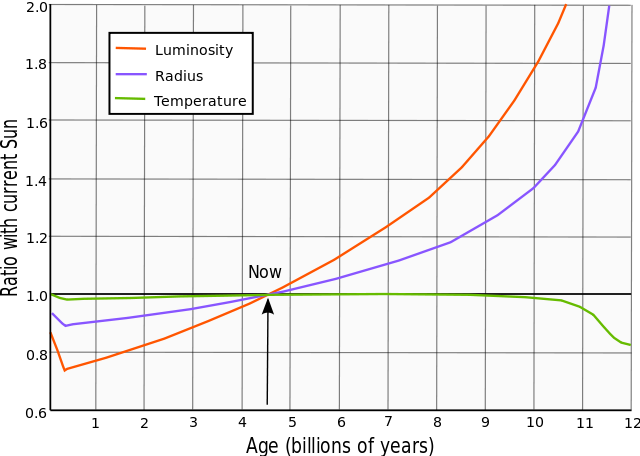
I refer you to https://astronomy.stackexchange.com/questions/35632/replenishing-hydrogen-in-the-core-of-the-sun/35636#35636 where I calculated that approximately $2\times 10^{29}$ kg of H will be turned into He during the main sequence lifetime of the Sun. However, you want to know what changes between now and the end of the main sequence. The plot below (by RJ Hall https://creativecommons.org/licenses/by-sa/3.0/deed.en ) shows a plot of how the solar luminosity (red line) changes with time to the end of the main sequence. If we assume that the avergae luminosity during the period between now and the end of the main sequence is $1.5 L_{\odot}$, then on average, 900 million tonnes of H is turned into He every second and thus $1.4 \times 10^{29}$ kg of H to He is converted between now and the end of the main sequence.

If the Sun is 73% H and 25% He now (with a mass of $2\times 10^{30}$ Kg), then the mass of H decreases by $1.4\times 10^{29}$ kg and the mass of He increases by almost the same amount. Tis changes the abundances to 66% H and 32% He.

Since the pp-chain is 0.7% efficient, the mass lost by the Sun corresponds to 5 billion years $\times 1.5L_{\odot}/c^2 = 10^{27}$ kg (or $5\times 10^{-4}M_{\odot}$)

At the same time, the Sun is losing mass through the solar wind. The mass loss rate is variable, it depends to some exten ton the solar cycle, and probably scales with the magnetic field of the Sun (which in turn depends on rotation) in poorly understood ways.

The current solar mass loss rate probably averages out at something like $2\times 10^{-14} M_{\odot}$/year. If that were maintained, then the Sun would only lose a further $10^{-4}M_{\odot}$ over the next 5 billion years via this route, but in all likelihood the mass-loss rate will diminish with time during the main sequence, so mass loss via a wind is negligible on the (late) main sequence.

However, in post-main-sequence evolution, mass-loss via winds becomes dominant.