I understand that electric potential is defined as the work needed to move a charge from infinity to a specific point in the field.
However, how does this apply for a field which is limited between two plates (a positive and negative plate) ?
How can the concept of electric potential as work to move a charge from infinity make sense in this scenario, as infinity is defined as zero electric potential, but then in the plates, the negative plate is defined as 0V?
I understand that electric potential is defined as the work needed to move a charge from infinity to a specific point in the field
This is the expression for the electric potential as a result of a point charge $Q$ obtained by referencing it to a zero point of potential at infinity. However, the nature of potential is that the zero point is arbitrary, like establishing the origin of a coordinate system. When the zero of potential is established the potential at other points is then measured with respect to that point.
How can the concept of electric potential as work to move a charge from infinity make sense in this scenario, as infinity is defined as zero electric potential...?
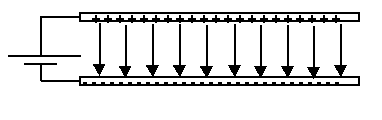
This scenario involves the potential difference between two parallel plates of equal and opposite charge (a capacitor), not the potential of an isolated point charge. The following working definition of potential difference applies to this scenario
The potential difference $V$ between two points is the work required per unit charge to move the charge between the two points
For the capacitor the work in Joules required to move a coulomb of charge between the plates, where $E$ is the uniform electric field between the plates in volts/meter (1 volt = 1 Joule/coulomb) and $d$ is the separation of the plates in meters.
$$W=Ed$$
...but then in the plates, the negative plate is defined as 0V?
One can assign the negative plate as 0V (and since in this case it makes sense since there is only one battery and capacitor), but remember the zero point is arbitrary. Suppose instead of a single capacitor connected to the terminals of a single battery, we had a circuit consisting of multiple components (capacitors, resistors, inductors) and multiple batteries. What would be 0 V then? Generally when doing circuit analysis one designates a convenient point in the circuit to be 0 V. Then the potential at every other point in the circuit is measured with respect to that point.
Hope this helps.
