Energy of Ground State of Quantum Well

Question

I struggle with the following concept.

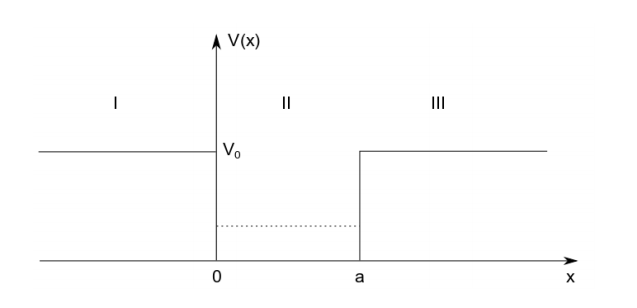
Consider the finite square well potential in the figure below. Consider the case where the electron energy is below the potential ($V(x) = V_0$) outside the well, and above in the potential ($V (x) = 0$) inside the well, as marked by the dashed line.

Our professor said that the following explanation is false:

"The energy of the ground state in the depicted potential is lower that the ground state energy for an infinite square well with length $a$. "

I just want to be sure to understand why that is so because he gave no further explanation. The reason is simply because an infinite well is, by definition, "greater" than a finite well, and thus also has more energy. Is that correct ?

Answer 1

I guess we can try using semi-classical approximation. Notice that in one 'period' the particle moves from $x=0$ to $x=a$ and back. This gives a phase shift $\gamma = -\pi$ since there are two smooth turning points in such an orbit. Use the Bohr-Sommerfeld equation ($n \in \mathbb{N} = \{0,1,2,...\}$):

$$\frac{1}{\hbar}\oint p_x dx = 2\pi n - \gamma = 2\pi(n+1/2).$$

And notice that $p_x = \pm \sqrt{2mE}$ in the well since the particle is free for $x \in (0,a)$. Take the $+$ solution and take the orientation of the integral such that $\mathbf{p}\parallel \hat{\mathbf{x}}$. This gives noticing that $p_x$ is constant in the well

$$\frac{1}{\hbar} (2p_x a) = \frac{2\sqrt{2mE}}{\hbar}a =2\pi (n+1/2).$$

Solving for $E = E_n$ we see that

$$E_n = \frac{h^2 (n+1/2)^2}{8ma^2}.$$

Notice that these are indeed smaller than for the infinite square well of same size $a$ and with $n \in \mathbb{N}$: $E_{n,\infty} = \frac{h^2 (n+1)^2}{8ma^2}$.