13
$\begingroup$

The concept of surface tension doesn't seem to be well explained in the first course on Fluid Mechanics. Fundamentals of Fluid Mechanics writes

A tensile force may be considered to be acting in the plane of the surface along any line in the surface. The intensity of the molecular attraction per unit length along any line in the surface is called the Surface Tenison .

There are a few things that are causing me problems:

  1. The analogy tensile force is quite hard to understand, I mean the force of attraction looks something like this enter image description here. As you can see, the molecules at the top have no upward force acting on them and therefore they form something like a surface (this what others writes). Well, okay there is no upward force but we can certainly go for superposition of forces and from the diagram, we can see that the upper molecule should accelerate downwards but it doesn't, why? How all this have any correlation with tension? (the way I have understood tension till now is the force that a string exerts on an object connected to it).

  2. The phrase along any line in the surface is causing problems because it writes in the surface not on on the surface which is quite hard to comprehend what the book intends.

I request you to please explain the concept of Surface Tension considering the problems that have written over here. If you present your personal understanding of the topic then it will be much appreciated.

Thank you.

EDIT: The concept of surface tension is causing me problem because what I’m thinking of surface tension is something like a stretched bed sheet on which things kept do not fall, but the problem is how this bed sheet analogy has arrived in fluids, I mean at the surface molecules and the mathematical definition of surface tension doesn’t make sense to me.

Improve this question
$\endgroup$
6
  • 1
    $\begingroup$ I was going to speculate that the meniscus (valley shape) at the surface provides the upward force, but menisci can also be dome-shaped or flat. $\endgroup$
    – user25959
    Commented Dec 5, 2019 at 17:12
  • $\begingroup$ Is that downward force at the surface molecule is called surface tension? In my drawing the downward shown at the top molecule, is it the surface tension? $\endgroup$
    – user240696
    Commented Dec 6, 2019 at 16:02
  • 1
    $\begingroup$ Have you got, hc Verma it is very well explained in that $\endgroup$
    – आर्यभट्ट
    Commented Dec 7, 2019 at 10:14
  • 2
    $\begingroup$ I prefer personal understanding because they are so much up to date and intuitive, books are intuitive too but the writers try to explain things with as much indisputably as possible (I know they have to do it) and that extra care sometimes makes a topic harder to comprehend. $\endgroup$
    – user240696
    Commented Dec 7, 2019 at 11:24
  • 1
    $\begingroup$ I will see, if other answers, if I feel I should write an answer, I will surely write an answer. $\endgroup$
    – आर्यभट्ट
    Commented Dec 7, 2019 at 11:32

3 Answers 3

Reset to default
11
$\begingroup$

Separating molecules requires work to be done against the attractive forces. So because molecules in the surface don't have molecules above them, they need less energy to move down into the bulk of the liquid than is needed for molecules to move from bulk to surface. Therefore the rate of movement of molecules due to their random thermal energy is greater surface to bulk than bulk to surface. [Compare Boltzmann factors exp$\left( -\frac{E_{S\ to\ B}}{kT}\right)$ and exp $\left(-\frac{E_{B\ to\ S}}{kT}\right)$.] This tends to deplete the surface layer, which in turn reduces the movement of molecules from surface to bulk, re-establishing (dynamic) equilibrium (equal rates of movement to and from the surface layer).

But with this 'new' dynamic equilibrium, the molecules are further apart in the surface layer than their usual separations so, recalling the intermolecular force curve, they attract each other, in other words the surface is under tension, like a stretched balloon-skin.

Improve this answer
$\endgroup$
19
  • 1
    $\begingroup$ I couldn't understand from This tends to deplete the surface layer.......... (equal rates of movement to and from the surface) . Can you please explain it ? $\endgroup$
    – user240696
    Commented Dec 11, 2019 at 8:31
  • 1
    $\begingroup$ Sorry. I simply meant that if more molecules are leaving the surface layer (in a given time) than are entering it from the bulk of the liquid, the surface layer will come to have fewer molecules in it. But this very thing will reduce the number of molecules per second leaving the surface layer, and this will soon make the number leaving the layer per second equal to the number arriving in the surface layer from the bulk of the fluid, so 'dynamic equilibrium' is re-established, but with molecules further apart in the surface layer than the bulk.. $\endgroup$
    – Philip Wood
    Commented Dec 11, 2019 at 8:48
  • 1
    $\begingroup$ Sir, I must say your logic is of very high level. Your explanation in the comment has really made me to realize the exact thing. $\endgroup$
    – user240696
    Commented Dec 11, 2019 at 8:58
  • 1
    $\begingroup$ Thank you. Suppose the surface layer to have a fixed area and to be two molecules thick. [This is an arbitrary simplification of a more subtle concept.] If that layer has lost more molecules than it has gained, there must be fewer molecules per unit volume, so the molecules must be further apart. $\endgroup$
    – Philip Wood
    Commented Dec 11, 2019 at 9:15
  • 2
    $\begingroup$ @ Knight You are too kind. I've failed to understand more points in Physics on first reading than I care to remember, so I do know the agony! You made a good point in one of your earlier comments about textbook writers being so anxious to avoid anything that could be seen as a mistake, that they can be hard to follow. A textbook that is both crystal clear and authoritative is a thing to be treasured! $\endgroup$
    – Philip Wood
    Commented Dec 17, 2019 at 18:22
7
$\begingroup$

Here are the quick answers to your questions, but if you're interested please read the more detailed explanation below.

1) The figure is incomplete as it doesn't include repulsive forces. The molecules do not fall because repulsive forces balance the attractive forces so there is no net force acting normal to the interface.

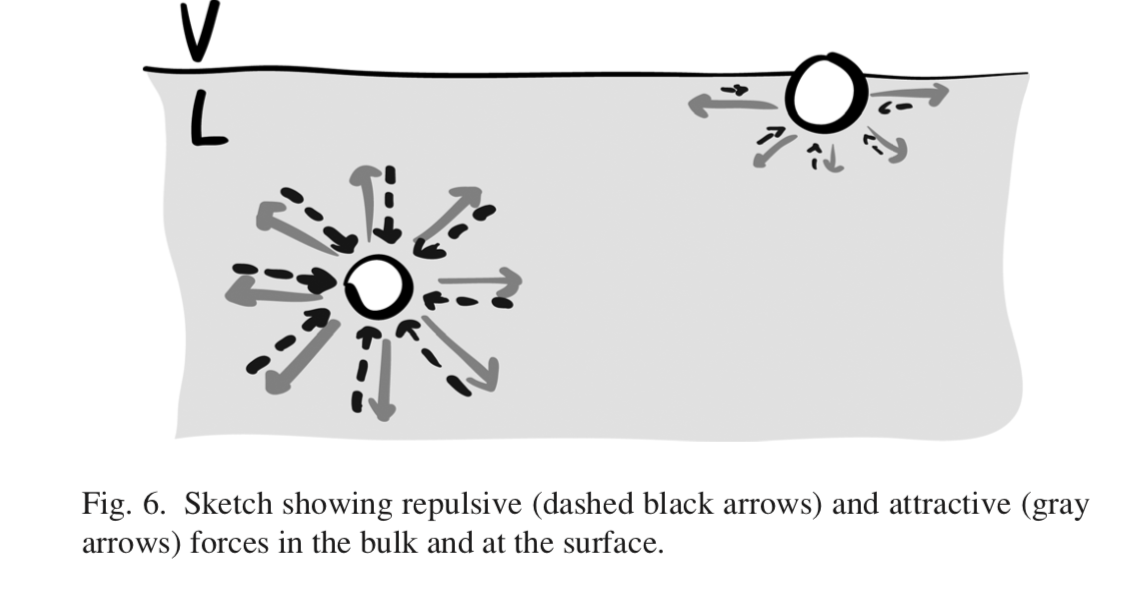enter image description here (Figure taken from Marchand et al 2011)

2) In continuum fluid mechanics, the fluid interface is modeled as a surface of zero thickness. In reality, the interface has some very small thickness and the surface tension can be defined as the integral of fluid stress across this very small thickness. Therefore the surface tension is the net force of the stress in the fluid interface. Typically we don't need this much detail and so we take the continuum approximation of the fluid interface and treat it as a mathematical surface of zero thickness and then state that surface tension acts on the surface.

Detailed explanation:

So to understand surface tension, it might be better to start with an understanding of interfaces and surfaces. At the beginning of any fluid dynamics course, you'll learn that we take a continuum approximation and model fluid molecules as a continuum so that we do not have to track each individual fluid particle. In order to ensure that this continuum model of fluids is accurate, we have defined certain concepts that help this continuum model accurately capture the dynamics of the fluid. Take for example viscosity, this is a continuum constant that essentially describes the how often fluid molecules collide and how momentum is diffused during these collisions.

Now think of a fluid interface between some vapor and liquid. I think most people studying fluid dynamics unintentionally conclude that the interface is some sort of physical sheet with zero thickness (such as the bed sheet analogy you mention). However this isn't exactly correct because the interface is in reality a layer of finite thickness over which the material properties change. Think density of the molecules, as seen in the figure below. If you look at the plot of density on the right, you see that the density of molecules in the interfacial region is not equal to the liquid density or the vapor density. 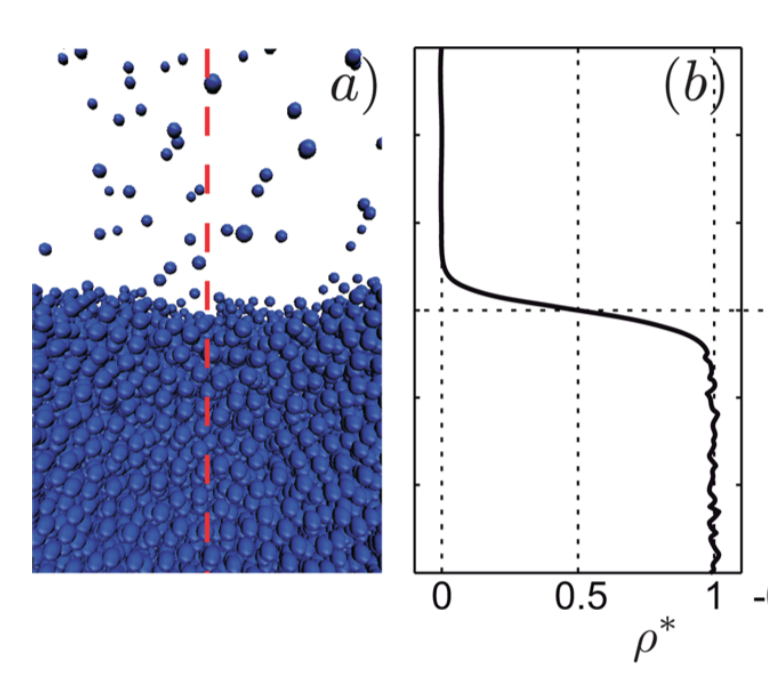enter image description here (Figure taken from Marchand et al 2011)

Now for most problems, we don't really need this much detail and so we've created a continuum approximation of the interfacial region and modeled it as a mathematical surface of zero thickness and zero mass. As mentioned before, the interface most definitely has some mass and volume, so in order to make sure that this continuum approximation of the fluid interface is physically accurate, we give it certain properties that capture the net effect of the molecules inside the interface, enter surface tension.

Surface tension has been defined in various ways (thermodynamic or mechanical) but they are all constistent in the end. The way you have learned is the mechanical definition, but it is somewhat incomplete as it doesn't include the repulsive forces acting on each molecule, see the first figure. In this more complete figure, you can see that all of the forces normal to the interface cancel out.

Lastly, surface tension being in or on a surface doesn't really make that much of a difference if you understand how we arrived at the continuum model of the fluid interface. If we think of interfaces as a region, then surface tension is the net force of the molecules in the interface. If we take the continuum model of the interface, then surface tension is described as the force on the surface.

All figures were taken from this paper:

Marchand, Antonin, et al. "Why is surface tension a force parallel to the interface?." American Journal of Physics 79.10 (2011): 999-1008.

If you have time I would recommend you read this paper as it explains several other topics that helped me better understand surface tension.

Improve this answer
$\endgroup$
6
  • 1
    $\begingroup$ that only tangential force remains ( 7th para, last line) why tangential forces don't get cancelled? $\endgroup$
    – user240696
    Commented Dec 11, 2019 at 8:39
  • $\begingroup$ Ah, my mistake. I should have stated that at the surface, the vertical component of the attractive and repulsive components balance each other. In the tangential direction, the attractive forces balance themselves and the repulsive forces balance themselves due to symmetry. $\endgroup$
    – Ragnar
    Commented Dec 12, 2019 at 8:10
  • $\begingroup$ Since they get balanced, how the tension is there? $\endgroup$
    – user240696
    Commented Dec 12, 2019 at 8:18
  • 1
    $\begingroup$ @Knight in ideal massless string loaded with 10kg tension at each and every point on the string (except endpoint) is equal and opposite. We still say that the string has tension, right? At the endpoint the tension is applied only on one side whereas the weight of the object balances the tension. Similarly if you "break" the surface by placing some object in it, the surface tension will act on the object. $\endgroup$
    – aditya_stack
    Commented Dec 12, 2019 at 12:18
  • 1
    $\begingroup$ @aditya_stack Wow, Thank You. It’s one of the clearest thing I have got on this topic. $\endgroup$
    – user240696
    Commented Dec 12, 2019 at 12:41
2
$\begingroup$

The analogy tensile force is quite hard to understand

Force exerted by anything when it is stretched (or attempted to stretch) is called tension. So not only strings, springs and membranes will also exert tension.

The concept of surface tension is causing me problem because what I’m thinking of surface tension is something like a stretched bed sheet on which things kept do not fall, but the problem is how this bed sheet analogy has arrived in fluids

If you place a piece of wire in water, tension will be exerted on the wire. More complete bedsheet analogy would be that placing an object in water is analogous to weaving something into the fabric of the bedsheet. Here the object very literally has the bedsheet fibres applying tension on it.

As you can see, the molecules at the top have no upward force

Generally air would be present so the molecules at the surface would have adhesive forces.

Well, okay there is no upward force but we can certainly go for superposition of forces and from the diagram, we can see that the upper molecule should accelerate downwards but it doesn't, why?

That is because the diagram is slightly misleading. It only shows attractive forces while both repulsive and attractive forces are acting on every molecule. If we assume that molecules are in static equilibrium (they are not as @Phillip Wood has pointed out and are in dynamic equilibrium but it is still a reasonable assumption to simplify our problem) then we cannot have imbalance of forces. If molecules are attracted to each other they will move towards each other until they are in equilibrium position and stay that way.

The phrase along any line in the surface is causing problems because it writes in the surface not on on the surface which is quite hard to comprehend what the book intends.

If a surface is thin enough lines need not be above or below the surface but can be in the surface just like how y=x is in the xy plane. This is more mathematics really.

Finally, if you place anything else on the water surface, the water molecules will exert tensile (pulling) force on the object. This force will act only at the boundary of the object with the water surface (since this is where the "bedsheet" is connecting to the object).

mathematical definition of surface tension doesn’t make sense to me.

If that object is a wire, the tensile force exerted at a point will be normal to the length of the wire at that point. The ratio of the magnitude of the tensile force to the length of the wire is what we call surface tension.

I hope this clears your doubts.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.