First of all your question is very vague and open to multiple interpretations. Secondly it lacks physical context. Thus I would like to point out the following two things.
Aerodynamics of the flow around a cylinder

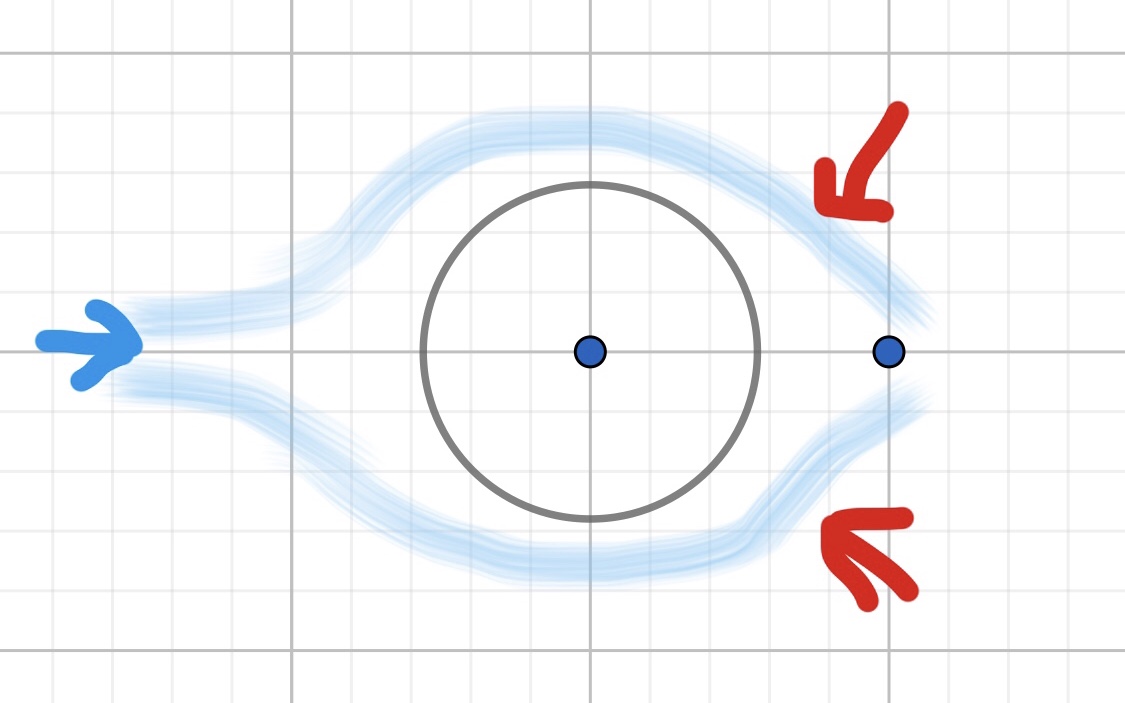
What you stated about the flow around a cylinder is only partially true. The fact that the flow might reattach to the cylinder is not caused by pressure but instead by viscosity. The entire space of a continuum is filled with a continuous mass. Thus the pressure can't lead to a reattachment of the flow - there were particles there the whole time. "Atmospheric" pressure is not a mighty external force, instead it is a measure for how much mass is squeezed into a given space and how fast the corresponding relative motion of those particles is. The important part for reattachment is the sole presence of particles and how these particles interact by means of shear forces. It is similar to rigid mechanics: No matter how hard you press together two plates as long as the friction coefficient is zero there will be no friction force. The "friction coefficient" of a fluid is the viscosity $\mu$ and thus basically describes the diffusion of momentum, how shearing layers transfer their momentum. Just take honey and air as examples: With the same static pressure their flow and reattachment to the cylinder will be different. As a consequence the flow in the wake of a cylinder for a continuum will be significantly more complex than what you would expect. Just because the geometry and boundary conditions are symmetric that does not mean that the solution will be. Depending on the flow regime there will be a perfectly symmetrical solution, an asymmetric steady state solution with standing vortex pairs and various types of oscillating von Kármán vortex streets. All the regimes are sketched here.
$Re << 1$ - creeping Stokes' flow: For very low Reynolds number $Re := \frac{U L}{\nu}$ (where $L$ is the diameter of the bottle $D$) the viscous effects will dominate. The inertia will be negligible and the pressure will press the flow against the bottle. As a result you will have an almost perfectly symmetrical flow in flow direction (similarly to what you have sketched). This is though very unlikely to be the flow you will generate by blowing at a bottle as flow velocities would have to be of order $U = \frac{Re \ \nu}{L} \approx \frac{1.5 \ 10^{-5}}{0.075} \frac{m}{s} \approx \mathcal{O} (10^{-4}) \frac{m}{s}$ for the medium air and a common bottle.
$3 \lesssim Re \lesssim 50$: For higher flow velocities two steady separation bubbles will appear. The Inertia starts to be dominating: The flow can't follow the abrupt change in curvature anymore and will separate. The dead water behind will be characterised by low (almost zero) velocity and higher pressure. The pressure will be so high that the incoming particles will stop or even revert their flow direction (backflow). The different velocities of main flow and flow in the wake will lead to a standing pair of vortices yet the flow will be still perfectly symmetrical.
$Re \gtrsim 50$: For all Reynolds numbers larger than that the flow will become unsteady and develop a Kármán vortex street: A tiny perturbation on one side of the flow will start to wash one of the vortices away. As the flow still has to be vortex free (circulation theorem) this induces a circulation that leads to the separation on the other side. As a result an oscillating flow will establish that restores the symmetry in a statistical sense. For lower Reynolds number this vortex street will separate in tube-like vortex structures but with increasing Reynolds number turbulent fluctuations will appear that destroy symmetry also in axial direction of the cylinder. The flow around your candle will actually look like this. This regime can be further split up depending on the properties of the boundary layer and there is also a phenomenon called turbulent reattachment. Interestingly such oscillating flow instabilities may also appear in geophysical flows such as depicted in the pictures below for the flow around the remote Juan Fernández islands (Chile).
As you can see it really depends on the flow regime (fluid, velocity and length scale) if your candle will be blown out or if it would continue burning in a continuum flow.
Flow in a "vacuum"
Vacuum is by definition space characterised by an absence of matter. So all we know about continuum flow (such as the Navier-Stokes equations) does not hold at all, there is nothing to flow: Mass flow and absence of matter are clearly contradictory concepts. Your piece of paper won't move, your candle won't be blown out - assuming your candle supplies also an oxidiser for the combustion reaction in the first place.
If you slowly lower the static pressure starting from a dense gas that can be described as a continuum (characterised by a Knudsen number $Kn \lesssim 0.01$), your gas will become slowly more dilute, the particles won't stick anymore to the wall: The no-slip condition will not hold anymore and instead you will be left with a slip condition ($0.01 \lesssim Kn \lesssim 0.1$). The fluid will start to behave like single particles moving like billiard balls according to Newton's laws (transitional $0.1 \lesssim Kn \lesssim 10$ and free molecular flow $Kn \gtrsim 10$): They will collide with each other and their surroundings and that will be the only source of momentum that could move your piece of paper.