3
$\begingroup$

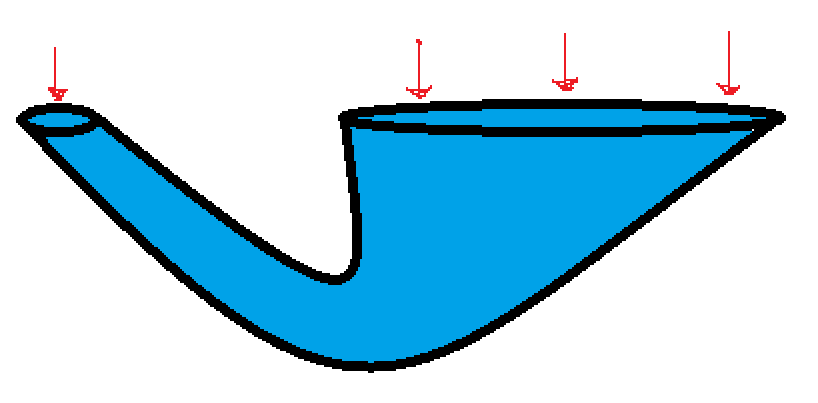
I can not understand why the surface area that atmospheric pressure is acting upon does not matter when calculating pressure.

For example look at the water jug below with 2 openings, one small and one big.

Diagram

The atmospheric pressure per cm2 is the same on both sides. However, since one hole has less surface area and the other has more surface area does that not mean that the total force being exerted on the bigger hole is bigger than the other? If that is the case, why does the water not shoot out of the smaller hole since the total force on the hole to the right is bigger?

I think my problem is that I am thinking of pressure in terms of weight, which is wrong perhaps. I imagine the above image as like a seesaw, where the right is heavier therefore the left should rise up but that is obviously not the case. Why is it not the case?

The same question worded differently would be: Why is the surface area of the mercury exposed to the air in a mercury barometer not taken into equation?

Improve this question
$\endgroup$
1

3 Answers 3

Reset to default
5
$\begingroup$

You have to imagine there is, on top of each holes, a very long air cylinder with a weight given by $$W=\rho V = \rho A h$$ where $V=AH$ is the volume of the cylinder, then rewritten as height time area, and $\rho$ is the density of air. So the pressure on each hole is $W/A$ i.e. $$p=\rho h$$ and $h$ (the height of the cylinder, which is the same in both case and is as high as the atmosphere [neglecting several atmospheric effects, as you can imagine...]) This means that, as you say, the weight of air on top of each hole is very different (because it depends on the surface $A$) but the pressure does not as the weights is distributed over different surfaces, i.e. the bigger weight (right hole) is distributed over a bigger surface.

For the same reason, considering now water cylinders inside your apparatus, a small volume of water "in the middle" will be in equilibrium if water is pressing on both sides with the same pressure and that happens when the height $h_w$ of the water is the same on both sides.

Improve this answer
$\endgroup$
5
  • $\begingroup$ Thank you for your answer. I understand that the pressure(force) on both holes is the same per unit of area. So does this mean that the surface of the water is also 'pushing up' on the air with its own pressure and this causes surface area to not matter since both pressures cancel each other out? $\endgroup$
    – Majiick
    Commented Jul 26, 2019 at 12:02
  • $\begingroup$ Respectfully (really): if you say "Pressure is the same per unit area", it indicates you don't fully understand pressure. $\endgroup$
    – JEB
    Commented Jul 26, 2019 at 12:31
  • $\begingroup$ @JEB Yeah, does not look like it ): I will pick up a physics book. I am thinking of pressure like rain droplets dropping on ground and exerting some force. If you put a 5m radius bucket in the rain, or a 100m radius bucket, the rain level(pressure) will still accumulate at the same rate, but the volume of water will be higher. Would it be more correct to say that pressure exerts the same amount of force per unit of area? Where I am stuck is the bigger bucket will experience more force overall, so just like a seesaw in the OP it should be unbalanced in my mind, but it obviously is not. $\endgroup$
    – Majiick
    Commented Jul 26, 2019 at 12:44
  • $\begingroup$ You may want to consider it in terms of energy, what minimizes $mgh$ over the system? Now on terms of hydraulic machines, the force imbalance (at constant pressure) is exactly what gives the huge mechanical advantage when pushing a piston down narrow side. $\endgroup$
    – JEB
    Commented Jul 26, 2019 at 13:32
  • $\begingroup$ I thought the hydraulic machine is basically based on the same principle as a lever, where the smaller piston just has to move a bigger distance because it is moving less volume of air per equal distance moved. $\endgroup$
    – Majiick
    Commented Jul 26, 2019 at 15:49
0
$\begingroup$

Because in terms of pressure per square inch or centimetre, the pressure is the same, and gravity ensures that water always finds its own level. Look at it this way: the surface of the ocean covers many trillions of square inches. Now stick a vertical pipe into the ocean with a bore of slightly more than one inch, so that the area of the water surface inside this pipe is exactly one square inch. The surface of the ocean adds up to trillions of square inches, each with 14.7 lb of atmosphere bearing down on it. The total pressure on the ocean surface therefore adds up to billions of tons, while the pressure on the surface confined within the pipe you have inserted is a mere 14.7 lb. According to your reasoning, the billions of tons total atmospheric pressure acting on the ocean surface should send a jet of water thousands of metres into the air from your pipe, which only has 14.7 lb to resist it. Since this is clearly absurd, you have proof that adding up the total pressure on the many square inches of ocean surface will lead you to false conclusions, so the only pressure that matters in both cases is the pressure per square inch, centimetre, foot or whatever unit you care to use..

Improve this answer
$\endgroup$
1
  • 1
    $\begingroup$ The force per unit area (i.e. pressure) is the same --- pressure per unit area is force per unit area$^2$ and as far as I know isn't a useful quantity $\endgroup$
    – DavidH
    Commented Jul 26, 2019 at 9:52
0
$\begingroup$

You are confusing weight, force and pressure and they are not the same thing.

Pressure is force on a small "control area".

Force is the force over the WHOLE area.

Weight is a force.

Pressure is force per some area. Pounds per square inch (psi).

SO...

With 1 pound per square inch and:

  • With 1 square inch you have 1 pound of force.

  • With 2 square inches you have 2 pounds of force.

  • With 10 square inches you have 10 pounds of force.

But all of these still have only 1 pound on ONE square inch.

So put a 10 pound weight on the big end and a 1 pound weight on the small end and they can balance.

OR push on the small end with 1 psi and you get a force of 10 pounds pushing out on the big end.

P.S. Yes, the water is pushing upward against the air pressure otherwise there would be movement. Replace the air with the same weight of a piston and everything must still balance with no movement...

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.