Concept regarding Barometer

Question

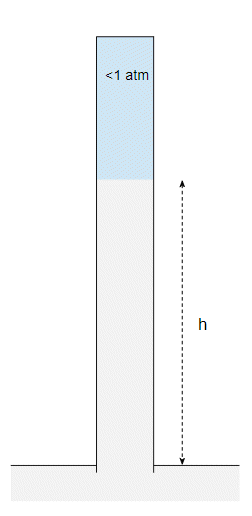
My question is based on the concept of barometer.

Q) To construct a barometer, a tube of length 1m is filled completely with mercury and is inverted in a mercury cup. The barometer reading on a particular day is 76cm. Suppose a 1m tube is filled with mercury upto 76cm and then closed by a cork. It is inverted in a mercury cup and the cork is removed. Height of mercury column in the tube over the surface in the cup will be

A) zero

B) 76cm

C) > 76 cm

D) < 76cm

The answer given is D) < 76 cm and when I also checked on the internet, many sources were saying the same. However, I'm unable to understand what actually happens here. I'm aware of the working of the barometer. In a normal barometer scenario without any tweaks, you fill a LONG tube (in this case 1m) COMPLETELY with mercury, close the mouth so that no AIR enters, then invert it into a cup of mercury. With the mouth of the tube immersed, the temporary closure is removed. It is observed that the mercury level in the tube falls till a point where the gravitational force of the mass of mercury in the TUBE BALANCES the upward force on the mouth of the tube which is nothing but the force caused by atmospheric pressure as the atmospheric pressure is acting on the mercury surface on the cup and since the mouth is at the same horizontal level, the pressure at the mouth is the same ATMOSPHERIC PRESSURE. So, the mercury level in the tube falls UNTIL the weight of mass of mercury remaining in tube is balanced by the upward force due to ATMOSPHERIC PRESSURE. As the region above the SURFACE of mercury in the tube is vaccuum, we can write

                               P = 0 + ρ g h

where ρ is density of mercury and h is height of column of mercury and P is atmospheric pressure.

But now as per question, THERE WILL BE AIR OCCUPYING the remaining region of the tube as only 76 cm out of 100 cm is filled with mercury. Now my question is, HOW IS THIS NOT ATMOSPHERIC PRESSURE? If the tube were just left to itself, we would have said the pressure acting on the surface of merucury is atmospheric pressure. If we close the mouth with a cork WHY SHOULD THIS CHANGE? Why will the pressure at the surface of mercury not be atmospheric pressure? My second question is IF IT IS ATMOSPHERIC PRESSURE; the fluid will be at equilibrium and not move WHEN THE HEIGHT OF MERCURY COLUMN FALLS TO 0 RIGHT? Since

                                 P = P + ρ g h 

will imply h=0.

So how do I analyse this? Why isnt the pressure at surface of mercury atmospheric pressure? What will it be? Can we predict what it will be? How will the answer be D) < 76 cm? I want to clear my concept I just mentioned above and then understand how the answer is D)

Answer 1

Start by imagining you have a piston full of air at atmospheric pressure:

Now imagine you pull downwards on the piston with some force $F$. This pulls the piston down and since it increases the volume of the air in the piston it decreases its pressure to less than one atmosphere. The pressure $P$ will decrease until it balances the applied force so we'll get:

$$ \frac{F}{A} = P_\text{atm} - P $$

where $A$ is the area of the piston.

In your experiment the column of mercury is pulling downwards just like the force in my diagram:

The mercury column would like to fall back down to the level of the mercury around it, and it's the decreased pressure of the air inside the column that holds it up. We'll get a similar equation to the one for our piston:

$$ \rho g h = P_\text{atm} - P $$

where $\rho$ is the density of the mercury. The left hand side, $\rho g h$ is the force pulling downwards on the air (divided by the area of the piston).