Below is a part of the book "Condensed Matter Field Theory" by Altland and Simon.
My question is about deriving the equation with red arrow. This is outlined in the exercise in the figure, but I don't understand the meaning of $$\sigma_{\alpha\beta}\cdot\sigma_{\gamma\delta}=2\delta_{\alpha\delta}\delta_{\beta\gamma}-\delta_{\alpha\beta}\delta_{\gamma\delta}$$ Deos $\sigma_{\alpha\beta}$ means Pauli matrix? Then why it has two subindices?
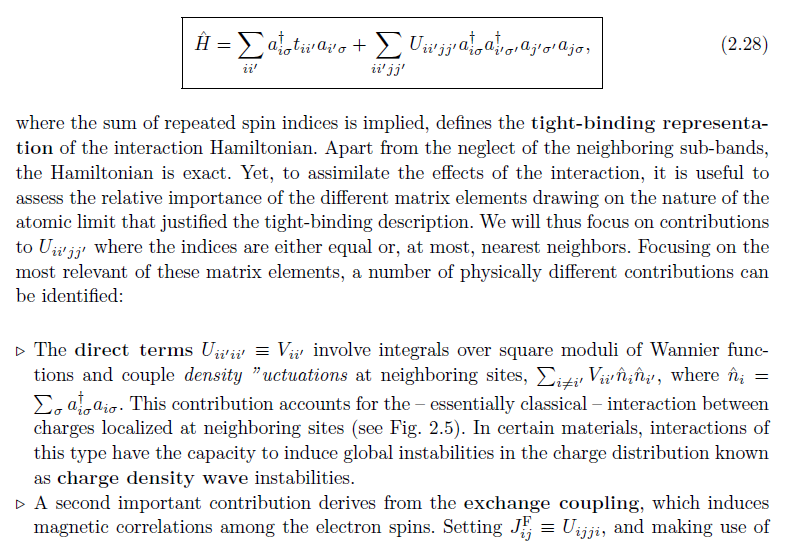
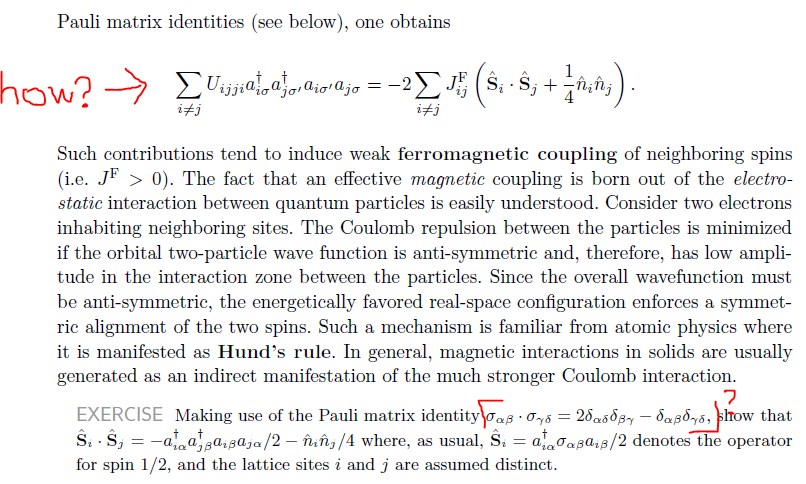
Using $\vec{\sigma}_{\alpha\beta}\cdot \vec{\sigma}_{\gamma\delta} = 2\delta_{\alpha\delta}\delta_{\beta\gamma}-\delta_{\alpha\beta}\delta_{\gamma\delta}$ (which I will prove later), we have (switching for convenience the index labels $\sigma \rightarrow \alpha$ and $\sigma' \rightarrow \beta$)
\begin{align} a_{i\alpha}^\dagger a_{j\beta}^\dagger a_{i\beta}a_{j\alpha} &= -a_{i\alpha}^\dagger a_{i\beta} a_{j\beta}^\dagger a_{j\alpha}\\ &= -a_{i\alpha}^\dagger a_{i\beta} a_{j\gamma}^\dagger a_{j\delta} \delta_{\alpha\delta}\delta_{\beta\gamma}\\ &= -\frac{1}{2} a_{i\alpha}^\dagger a_{i\beta} a_{j\gamma}^\dagger a_{j\delta} \left( \vec{\sigma}_{\alpha\beta}\cdot \vec{\sigma}_{\gamma\delta} + \delta_{\alpha\beta}\delta_{\gamma\delta} \right). \end{align}
Using $\mathbf{S}_{i;\alpha\beta} = \frac{1}{2}a_{i\alpha}^\dagger a_{i\beta}\vec{\sigma}_{\alpha\beta}$ and $n_i = a_{i\alpha}^\dagger a_{i\alpha}$, the above becomes
\begin{align} a_{i\alpha}^\dagger a_{j\beta}^\dagger a_{i\beta}a_{j\alpha} &= -2 \left( \mathbf{S}_i \cdot \mathbf{S}_j + \frac{1}{4} n_i n_j \right). \end{align}
Multiplying this equality by $J^F_{ij}\equiv U_{ijji}$ and summing over $i \neq j$, we find
$$H_{x} = -2\sum_{ij}J^F_{ij}\left( \mathbf{S}_i \cdot \mathbf{S}_j + \frac{1}{4} n_i n_j \right),$$
as desired.
A proof of the Pauli matrix identity can be found on Wikipedia, but I reproduce it below for convenience. Recall that the Pauli matrices, coupled with the identity matrix (which I denote $\sigma^0$), comprise a complete basis of the space of $2\times2$ matrices. As such, any matrix $M$ can be written as
$$M = \sum_{k=0}^4 c_k \sigma^k$$
for some coefficients $c_k$. Using the fact that $\mathrm{Tr}(\sigma^i) = 0$ and $\mathrm{Tr}[\sigma^i\sigma^j] = 2\delta_{ij}$ for $i=1,2,3$, one finds $c_0 = \frac{1}{2}\mathrm{Tr}M$ and $c_i = \frac{1}{2}\mathrm{Tr}[\sigma^i M]$. We therefore have (switching to index notation for later convenience)
$$M_{\alpha\beta} = \frac{1}{2}\mathrm{Tr}M \delta_{\alpha\beta} + \sum_{i=1}^3\frac{1}{2}\mathrm{Tr}[\sigma^i M]\sigma^i_{\alpha\beta}.$$
Writing out the traces in index notation, this becomes
$$M_{\alpha\beta} = \frac{1}{2}M_{\gamma\gamma} \delta_{\alpha\beta} + \sum_{i=1}^3 \frac{1}{2}\sigma^i_{\gamma\delta}M_{\delta\gamma}\sigma^i_{\alpha\beta}.$$
Rewriting $M_{\alpha\beta} = M_{\delta\gamma}\delta_{\alpha\delta}\delta_{\beta\gamma}$ and $M_{\gamma\gamma} = M_{\delta\gamma}\delta_{\gamma\delta}$ and noting that this equality holds for any matrix $M$, we find
$$\delta_{\alpha\delta}\delta_{\beta\gamma} = \frac{1}{2}\delta_{\alpha\beta}\delta_{\gamma\delta}+\frac{1}{2}\vec{\sigma}_{\alpha\beta}\cdot \vec{\sigma}_{\gamma\delta}.$$
Rearranging this gives the desired Pauli matrix identity.
