1
$\begingroup$

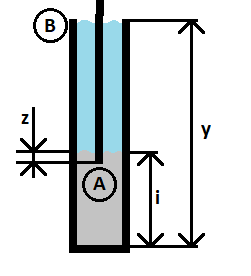
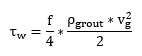I am trying to calculate the pumping pressure needed to backfill a borehole with geothermal grout. The hose is pulled up at the same rate as the borehole fills, so pumping pressure will get lower as the hole is filled. The borehole is initially filled with water and is filled bottom to top.

The way I am calculating it now is like this:

enter image description here

enter image description here

With:
Pb: pressure at B (start of the hose going into the borehole).
i: Percentage of the borehole filled with grout).
ρ: Density.
g: acceleration due to gravity.
y: depth of the borehole.
τw: friction loss from hosewall.
D: diameter of the hose.
f: Friction coefficient.
vg: velocity of the grout in the hose.

enter image description here

Problem is: I don't know if this is correct. Can somebody validate this or come with the right way to calculate this?

Improve this question
$\endgroup$
5
  • $\begingroup$ Have a look at oil drilling manuals, they do those calculations all the time: petrowiki.org/… $\endgroup$
    – patta
    Commented Apr 30, 2019 at 11:56
  • $\begingroup$ Can you explain a little more? what is $-\rho g y$? $\endgroup$
    – patta
    Commented Apr 30, 2019 at 12:11
  • $\begingroup$ You say that $\rho$ stands for density, but which density? $\endgroup$
    – Chet Miller
    Commented Apr 30, 2019 at 12:12
  • $\begingroup$ Density of the grout or density of the water, in −ρgy it is the density of the water. $\endgroup$
    – Kiteman
    Commented Apr 30, 2019 at 12:43
  • $\begingroup$ −ρgy is the hydrostatic pressure at point A $\endgroup$
    – Kiteman
    Commented Apr 30, 2019 at 12:44

2 Answers 2

Reset to default
1
$\begingroup$

A force balance on the grout column gives $$P_B+\rho_{grout}gy(1-i)-P_A-4\frac{\tau_w y}{D}=0$$This neglects any acceleration of the grout fluid and any drag caused by the upward motion of the hose. For the water, the force balance is $$P_A=\rho_{water}gy(1-i)$$ So, $$p_B=-(\rho_{grout}-\rho_{water})gy(1-i)+4\frac{\tau_w y}{D}$$So the density difference between the grout and the water reduces the pressure at B, and the grout fluid drag flow increases the pressure at B.

Improve this answer
$\endgroup$
5
  • $\begingroup$ This argument makes sense for me, only I will put the $i$ as fraction of filled column, as the OP, so you have for example $P_A = \rho_w g y (1-i)$ $\endgroup$
    – patta
    Commented Apr 30, 2019 at 12:51
  • $\begingroup$ @patta That doesn't seem correct to me. $P_A$ is determined by the weight of the column above A. $\endgroup$
    – Chet Miller
    Commented Apr 30, 2019 at 13:20
  • $\begingroup$ agree, but from the drawing, $y$ is the total well depth (not the depth of the column above A). Your calculation works with $y$ as the depth of A $\endgroup$
    – patta
    Commented Apr 30, 2019 at 13:24
  • 1
    $\begingroup$ @patta Oops, missed that. I had read y as the depth at A. I've edited my answer. Thanks. $\endgroup$
    – Chet Miller
    Commented Apr 30, 2019 at 13:48
  • $\begingroup$ now the wolrd is safer! $\endgroup$
    – patta
    Commented Apr 30, 2019 at 14:00
0
$\begingroup$

Some temporary fixes, in random order:

  1. If the bottom is open (in contact with formation fluid) then you need to keep the bottom at its own specific pressure $P_f$ (which depend on the well), to have equilibrium between the well and the rock fluid, otherwise the fluid will invade your well, or opposite. Is that your $-\rho g y$ term? The outflow of water at B then need to be kept under pressure (wellhead)
  2. between A and B you have the hydrostatic pressure of the grout, that helps you in filling.
  3. So let $P_f$ the pressure at the bottom; then you have at point A $P_A= P_f - i \rho_g g y$ and your hose pumping form B, $P_B = P_A - (1-i) \rho_g g y + friction = P_f - \rho_g g y + friction$; this looks simple
  4. The messy one is the water pressure on top
  5. If you have to do that for real, you should consider also the dynamic pressure (when you start and stop pumping) ....
Improve this answer
$\endgroup$
3
  • $\begingroup$ Ok, so you keep the top free water surface at atmospheric pressure? $\endgroup$
    – patta
    Commented Apr 30, 2019 at 12:47
  • $\begingroup$ Yes, it is just a hole in the ground filled with water that needs to be filled with grout to ensure no contamination can reach the groundwater in the earth. $\endgroup$
    – Kiteman
    Commented May 1, 2019 at 8:05
  • $\begingroup$ Ok, so you can go with the solution by @Chet Miller. Note that the grout will be "sucked down" by being heavier than clear water. So you can pour it in with zero pressure (free flowing from a tank) and it will adjust the speed $v_g$ until the well is about filled up. $\endgroup$
    – patta
    Commented May 1, 2019 at 9:12

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.