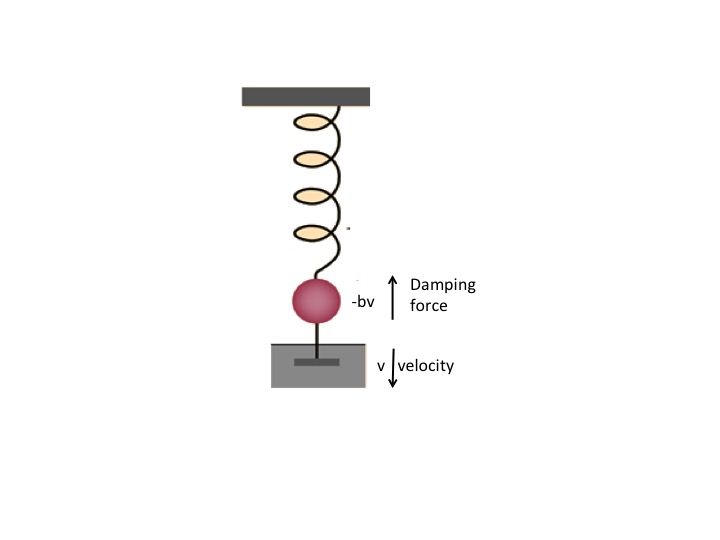
I learn that damping force with regard to forced damped oscillations is given by F = -bv where is the velocity of the object measured from ground frame. Suppose we are in a frame which is moving with a velocity v'. Will the damping force in this new frame be F = -b(v-v') or will it remain -bv. This doubt came when i solving a problem related to forced damped oscillation of a pendulum with its suspension point oscillating. Im arriving at the correct solution by assuming the force is -bv in the non-inertial frame of the moving suspension point instead of -b(v-v') but im not able to understand why?
$\begingroup$
$\endgroup$
Add a comment
|
2 Answers
$\begingroup$
$\endgroup$
The velocity $v$ in the expression $F = -b\,v$ is a relative velocity. Relative to what depends on the physical situation.
Let us imagine a spring with a mass attached to it and placed inside a motionless fluid (see note below). If the speed of the mass relative to the fluid is small, we can in approximate way write
$$F = -b\,v$$
where $v$ is the velocity of the mass with respect to the fluid.
In other situations, $v$ will be a different relative velocity. We know from a general principle that it must be a relative velocity: there are no absolute reference frames, so it is not possible to specify an absolute velocity in an equation that describes a physical process.
- Note: the fluid is "motionless" in an inertial reference frame. I am intending then that the various parts of the fluid do not move with respect to each other.
$\begingroup$
$\endgroup$
Im arriving at the correct solution by assuming the force is -bv in the non-inertial frame of the moving suspension point instead of -b(v-v') but im not able to understand why?
If you are in a reference frame moving at constant velocity v', you are in an inertial reference frame, not a non-inertial frame. The damping force and damping dissipation is the same for all inertial frames. So you are arriving at the correct solution, but the reference frame is inertial, not non-inertial.
Consider the damped harmonic oscillator of the figure below. The spring, mass and damper together form a system. The velocity of the damper is internal to the system. Viscous damping is dissipative, i.e., it dissipates heat.
The system as a whole can have any constant velocity with respect to any inertial frame of reference and this will not affect the damping force nor the dissipative effects of the damper.
In short the damping of the oscillator is the same in all inertial frames of reference.
Hope this helps.
