Spring mass system

Question

While solving some spring block questions one doubt came in my mind ..is it possible to calculate maximum velocity of a point between two springs of different force constant attached to a block of some mass and stretched to a finite distance slowly? enter image description here

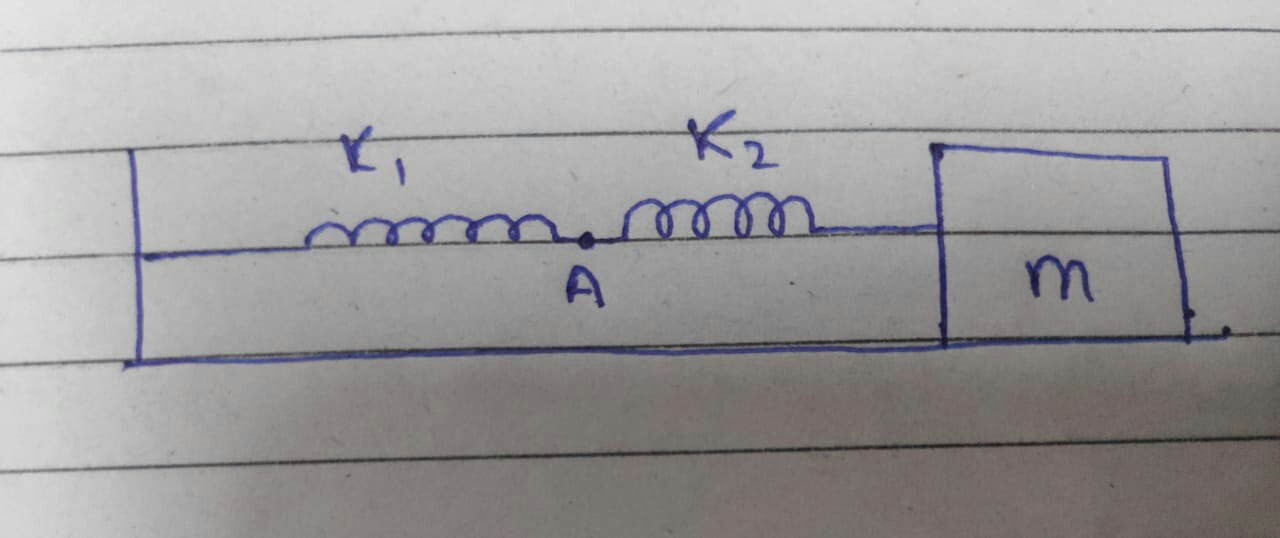
Lets say if in this diagram i have to find the velocity of A...

Will it be the same as maximum velocity of the body ..if we find effective spring constant and apply energy conservation to find maximum velocity of the body?

Answer 1

You can assume $x_1$ and $x_2$ as the extension from both the side Then $x_1+x_2=\text{length stretched}$

Since the force on the point will be zero as it is mass less we can equate the force on it $$k_1x_1=k_2x_2 $$

Now putting $x_1$ in the first equation and get the value of $x_2$.

And since the system is performing SHM Maximum velocity will be $A\omega$ where A is amplitude and $\omega =\sqrt{\frac{k}{m}}$, $k$ will be the effective spring constant.

Since in this case it is in series effective spring constant will be $$\frac{k_1\times k_2}{k_1+k_2}$$

Therefore maximum velocity will be $$x_1\times \sqrt{k/m}$$