Mass-Spring-Damper System Confusion

Question

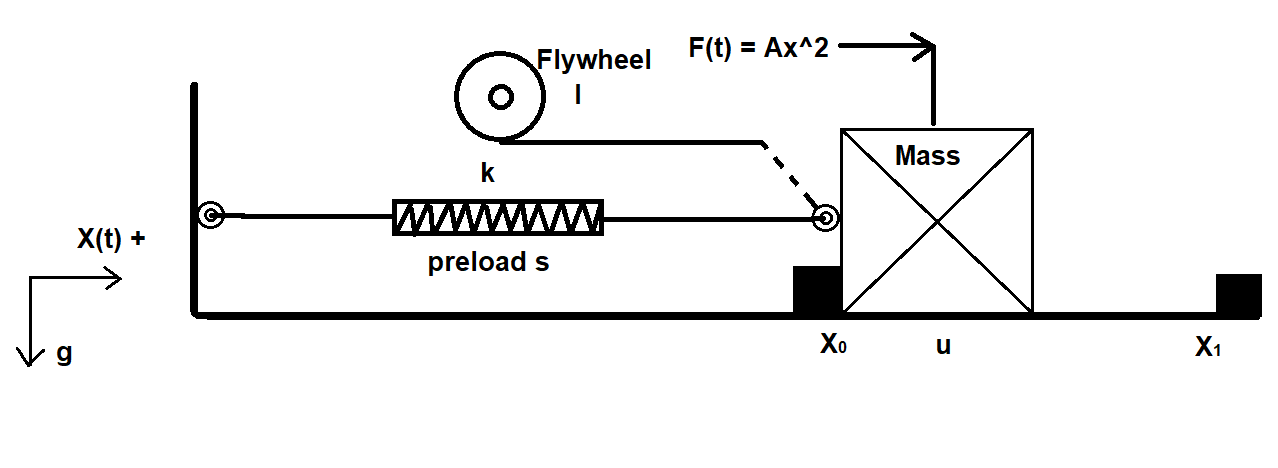
I am a ME student trying to understand this system pictured below. I have a mass sliding on a surface with a coefficient of friction $u$, attached by inextensible massless rope to a tension spring of constant $k$, and also attached to a flywheel with a moment of inertia $I$ which spins as the mass slides $x$+ and acts as a damper. The forcing function $F(t)$ is of the form $Ax^2$. The mass is confined to only slide between two stops and the spring is already preloaded under some tension (i will define this as it is stretched by $s$). Essentially, a small burst pushes the mass $x$+ for some distance, damped by friction and the flywheel and then returns to $x(0)$. I would like to assume it is a slightly underdamped system, where it impacts the end stops with some non-zero velocity, and the stops sort of truncate the natural travel. I would imagine that this problem is best solved by using a work-energy balance, but it looks like it fits a second order DE system well. Could someone provide me some guidance on how to solve for the travel function $x(t)$ for this?

I would like to add that I came up with this problem and it is not a homework assignment I just want to cheat on, I drew the diagrams and would just like to get a better understanding of my basic physics.

Below is the block diagram and a graph showing the assumed behavior:

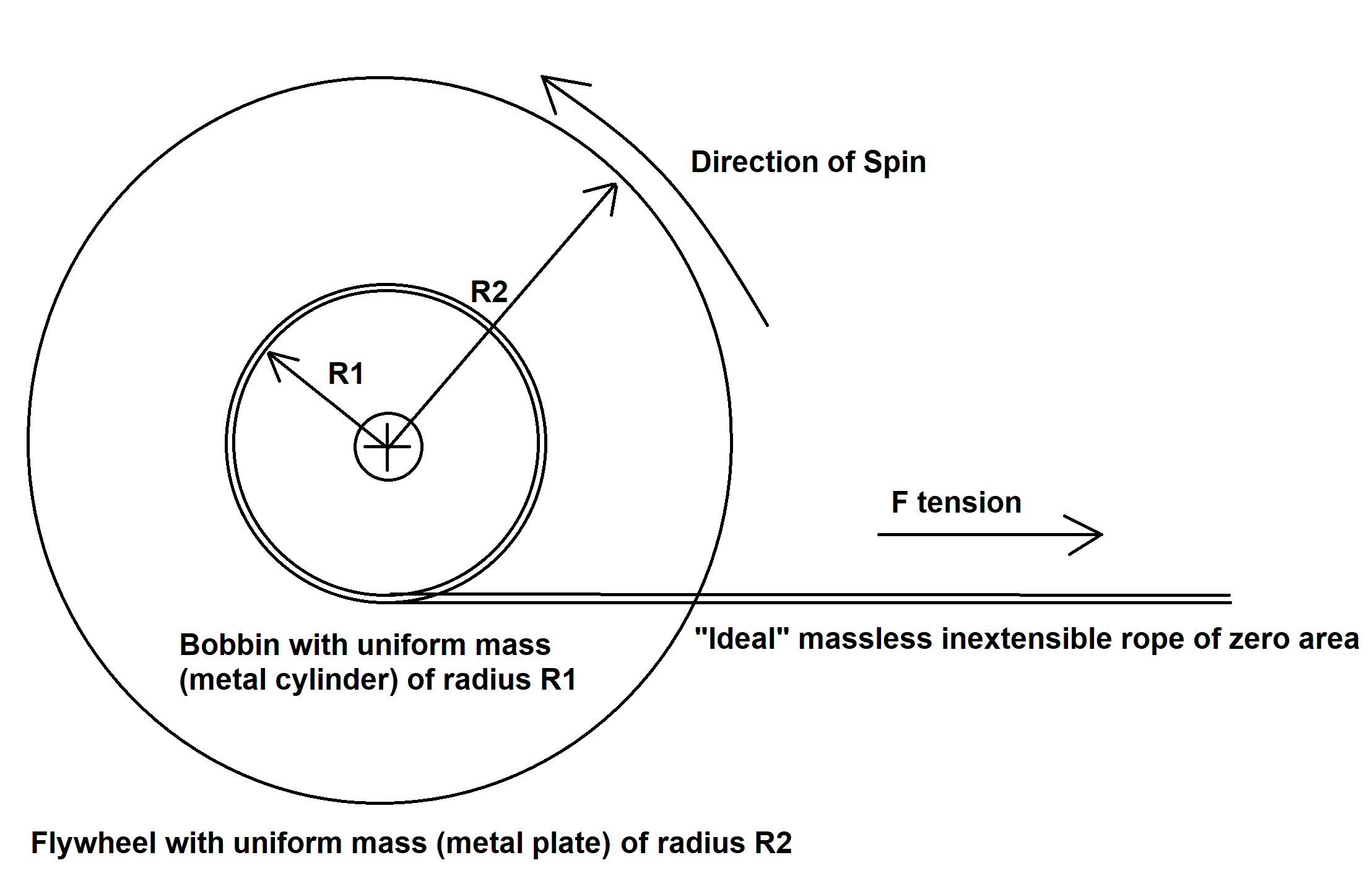
Per the comments below I have added this diagram showing an alternative set up where the rope attaching the mass to the flywheel is wrapped around a bobbin with a smaller diameter than the flywheel.

Answer 1

The Newton equations of motion are

$$m\,\ddot x+k\,(x-x_0)=F(t)+F_c-F_\mu\tag 1$$ $$I\,\ddot \phi+d\,\dot\phi=-F_c\,R\tag 2$$

and the kinematic equations

$$x=R\,\phi\quad\Rightarrow ~,\dot x=R\,\dot\phi~,\ddot x=R\ddot\phi\tag 3$$

you have now three equations for the three unknowns $~\ddot x~,\ddot\phi~$ and the constraint force $~F_c~$

you obtain

$$(I+m\,R^2)\ddot x=\left[ -k \left( x-{x_0} \right) +F \left( t \right) -F_{{\mu}} \right] {R}^{2}-d\,{\dot x} $$

where $~F_\mu=\mu\,m\,g\frac{\dot x}{|\dot x|}~$ and $~F(t)=A\,x^2~$

Answer 2

I would imagine that this problem is best solved by using a work-energy balance, but it looks like it fits a second order DE system well. Could someone provide me some guidance on how to solve for the travel function x(t) for this?

Because there is friction involved kinetic energy is not conserved.

So you need to set up a Newtonian Equation of Motion.

$$F(t)=-k(x-x_0)-mguv-F_{Flywheel}$$ $$ma(t)+k(x-x_0)+mguv+F_{Flywheel}=0\tag{1}$$

For $F_{Flywheel}$ consider the flywheel of radius $R$ and Intertial moment $I$. Motion of the mass requires angular acceleration of the flywheel so that:

$$\tau=I\alpha$$

$$v=\omega R$$

$$\frac{\mathrm{d}v}{\mathrm{d}t}=\alpha R$$

$$F_{Flywheel}R=\tau R$$

$$F_{Flywheel}=\frac{\mathrm{d}v}{\mathrm{d}t}\frac{I}{R}$$

Inserting into $(1)$ we get:

$$Ax^2+k(x-x_0)+mgu\frac{\mathrm{d}x}{\mathrm{d}t}+\frac{\mathrm{d}^2x}{\mathrm{d}t^2}\frac{I}{R}=0\tag{2}$$

This may be solved by means of an integration constant (for second order ODE).