Clock in a sealed box at half the speed of light. How would it know it is moving?

Question

Consider a clock enclosed in a sealed box here on earth syncronized with my wrist watch. I send the boxed clock around the galaxy a couple of times at half the speed of light (or the full speed of light..). I hear that the clocks will be out of sync when the boxed clock comes back on earth.
Is that true?
How would the boxed clock know it was moving when it was actually stationary in reference to the box?
I hear explanations about how when traveling from point A to point B, time and space became one. I do not understand that, but that's besides the point. In this question point A is same as point B. So the clock has come back to where it started. Does the time for the moving clock somehow come back to be in sync with the wrist watch ?
Would the effects of departing the earth at c cancel out because of the effects of approaching when the jurney is over?

Answer 1

If traveling inertially, the clock in the box doesn't know if it's moving.
That's the "Principle of Relativity"!

The following elaborates on Dale's answer.

Geometric analogies are useful.

• On a geometrical plane, a straight-line path from point A to B
  (regardless of the direction from A to B)
  has a length which is computed by chaining together a sequence of short straight-line segments from A to B.
  (This means that the path from A to B doesn't have to be in any particular direction, like vertical or horizontal.)
• In spacetime, an inertial path from event A to B
  (regardless of the inertial velocity from A to B as viewed in your frame) has an elapsed-wristwatch-time which is computed by chaining together a sequence of short inertial wristwatch-ticks from A to B.
  (This means that the inertial path from A to B doesn't have to be in any particular velocity, like "at rest".)

For piecewise paths...

• Triangle inequality: Instead of going a along straight-line-path from A to C, you take (for simplicity) piecewise-straight-line-paths A to M, then M to B. From Euclidean geometry, it turns out that the straight-line-distances satisfy: $$dist(A,M)+dist(M,B) > dist(A,B).$$
  "The straight-line path has the shortest distance."
  So, the car from A to B will have less tire-wear than the car from A to M to B.

• Clock Effect: Instead of going along an inertial-path from A to B, you take (for simplicity) piecewise-inertial-paths A to M, then M to B. From special relativity, it turns out that the inertial-path-elapsed-times satisfy: $$t(A,M)+t(M,B) < t(A,B). $$
  "The inertial path logs the longest elapsed-time."
  So, the inertial-observer from A to B will have aged more than the non-inertial observer from A to M to B.

• Common-Sense Galilean-relativity: Instead of going along an inertial-path from A to B, you take (for simplicity) piecewise-inertial-paths A to M, then M to B. From Galilean relativity, it turns out that the inertial-path-elapsed-times satisfy: $$t(A,M)+t(M,B) = t(A,B).$$
  "The elapsed time logged from A to B is independent of the path from A to B."
  So, the inertial-observer from A to B will have aged as much as the non-inertial observer from A to M to B.

---

Admittedly, it's difficult to visualize the special relativity case because of the non-Euclidean geometry of Minkowski Spacetime. (It is underappreciated that the ordinary position-vs-time graph is also a non-Euclidean geometry... but we've learned to extract information from that diagram.)

To help in "visualizing proper time", here is a spacetime diagram on rotated graph paper.
The key feature in the construction is that the area of the "light-clock diamonds" (marking off the ticks of a wristwatch) are equal in magnitude along different inertial worldlines.
The traveller leaves at (3/5)c and returns at (3/5)c in order to meet up at the inertial-observer's 10-tick anniversary after separation.

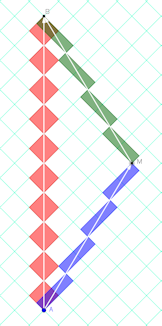
When they reunite, the non-inertial traveler (although piecewise-inertial) is younger than the [always] inertial observer.

Although this diagram is drawn from the stay-at-home frame, the result is independent of the frame of reference.
And there is no Lorentz transformation that will ever straighten out A-M-B to be an inertial path. A ball sitting on a frictionless surface on the traveller's ship will move when the ship necessarily-turns at M. (The analogous situation is true in Galilean relativity.)
(Just like in ordinary geometry... the lengths and angles in a triangle are the same if the figure is rotated. And no rotation will unkink A-M-B to be a straight-line.)

---

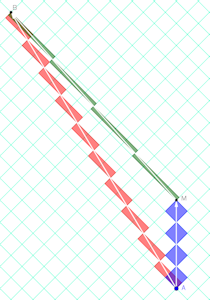
For more information about these spacetime-diagrams on rotated graph paper,
check out my paper ("Relativity on Rotated Graph Paper", Am. J. Phys. 84, 344 (2016); http://dx.doi.org/10.1119/1.4943251 ; early draft: https://arxiv.org/abs/1111.7254 ).
See also my informal presentation at https://www.physicsforums.com/insights/relativity-rotated-graph-paper/ and my GeoGebra presentation at https://www.geogebra.org/m/HYD7hB9v#

Answer 2

I hear that the clocks will be out of sync when the boxed clock comes back on earth. Is that true?

Yes, this is a standard relativity scenario called the “twin’s paradox”. The traveling clock or twin returns younger. There are several ways that work to understand this. The best collection is this one:

http://math.ucr.edu/home/baez/physics/Relativity/SR/TwinParadox/twin_paradox.html

My favorite approach is the spacetime diagram approach because it works for special relativity as well as general relativity. All you have to do is to integrate the metric.

$$\tau = \int d\tau$$

In units where c=1 in an inertial frame $d\tau^2=dt^2-dx^2-dy^2-dz^2$. Simply compute that for each clock and you get the amount of elapsed time.