If traveling inertially, the clock in the box doesn't know if it's moving.
That's the "Principle of Relativity"!

The following elaborates on Dale's answer.

Geometric analogies are useful.

• On a geometrical plane, a straight-line path from point A to B
  (regardless of the direction from A to B)
  has a length which is computed by chaining together a sequence of short straight-line segments from A to B.
  (This means that the path from A to B doesn't have to be in any particular direction, like vertical or horizontal.)
• In spacetime, an inertial path from event A to B
  (regardless of the inertial velocity from A to B as viewed in your frame) has an elapsed-wristwatch-time which is computed by chaining together a sequence of short inertial wristwatch-ticks from A to B.
  (This means that the inertial path from A to B doesn't have to be in any particular velocity, like "at rest".)

For piecewise paths...

• Triangle inequality: Instead of going a along straight-line-path from A to C, you take (for simplicity) piecewise-straight-line-paths A to M, then M to B. From Euclidean geometry, it turns out that the straight-line-distances satisfy: $$dist(A,M)+dist(M,B) > dist(A,B).$$
  "The straight-line path has the shortest distance."
  So, the car from A to B will have less tire-wear than the car from A to M to B.

• Clock Effect: Instead of going along an inertial-path from A to B, you take (for simplicity) piecewise-inertial-paths A to M, then M to B. From special relativity, it turns out that the inertial-path-elapsed-times satisfy: $$t(A,M)+t(M,B) < t(A,B). $$
  "The inertial path logs the longest elapsed-time."
  So, the inertial-observer from A to B will have aged more than the non-inertial observer from A to M to B.

• Common-Sense Galilean-relativity: Instead of going along an inertial-path from A to B, you take (for simplicity) piecewise-inertial-paths A to M, then M to B. From Galilean relativity, it turns out that the inertial-path-elapsed-times satisfy: $$t(A,M)+t(M,B) = t(A,B).$$
  "The elapsed time logged from A to B is independent of the path from A to B."
  So, the inertial-observer from A to B will have aged as much as the non-inertial observer from A to M to B.

---

Admittedly, it's difficult to visualize the special relativity case because of the non-Euclidean geometry of Minkowski Spacetime. (It is underappreciated that the ordinary position-vs-time graph is also a non-Euclidean geometry... but we've learned to extract information from that diagram.)

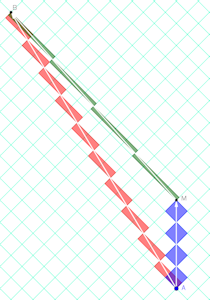
To help in "visualizing proper time", here is a spacetime diagram on rotated graph paper.
The key feature in the construction is that the area of the "light-clock diamonds" (marking off the ticks of a wristwatch) are equal in magnitude along different inertial worldlines.
The traveller leaves at (3/5)c and returns at (3/5)c in order to meet up 10-ticks after separation.

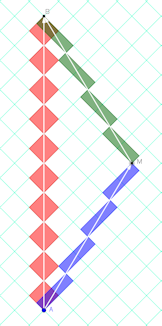
When they reunite, the non-inertial traveler (although piecewise-inertial) is younger than the [always] inertial observer.

Although this diagram is drawn from the stay-at-home frame, the result is independent of the frame of reference.
And there is no Lorentz transformation that will ever straighten out A-M-B to be an inertial path. A ball sitting on a frictionless surface on the traveller's ship will move when the ship necessarily-turns at M. (The analogous situation is true in Galilean relativity.)
(Just like in ordinary geometry... the lengths and angles in a triangle are the same if the figure is rotated. And no rotation will unkink A-M-B to be a straight-line.)