I read this as basic rules or laws you can say in REFLECTION from concave mirror.
$\begingroup$
$\endgroup$
Add a comment
|
5 Answers
$\begingroup$
$\endgroup$
5
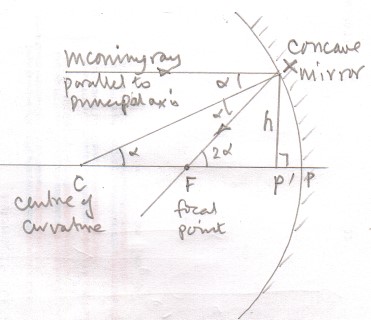
Consider an incoming ray which is parallel to the principal axis which hits a concave mirror at $X$.
The normal to this mirror at $X$ passes through the centre of curvature of the mirror $C$.
$\frac {h}{CP'}= \tan \alpha, \, \frac {h}{FP'}= \tan 2\alpha, \, $
For small $\alpha$ ie incoming ray close to the principal axis
$CP' \approx CP,\, FP' \approx FP,\, \tan \alpha \approx \alpha,\, \tan 2\alpha \approx 2\alpha$ where $P$ is the pole of the mirror.
$\Rightarrow CP \approx 2 FP$
$CP$ is a property of the mirror and $FP$, the focal length of the mirror, is thus (approximately) independent of the angle $\alpha$ as long as $\alpha$ is small.
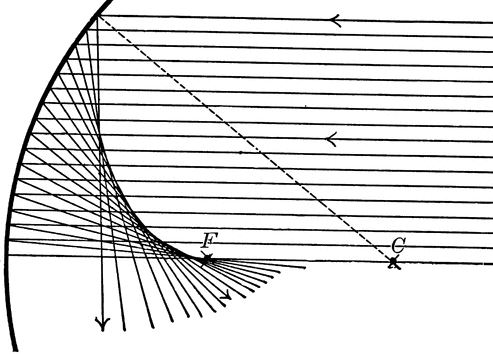
If $\alpha$ is not small then a mirror defect called spherical aberration occurs as shown below.
(On Desmos)
You often see this whilst having a drink with the bright line being called a caustic curve.
-
$\begingroup$ Converging on the focal point never made sense to me in thought experiments, so I never fully got optics. Turns out that actually, the rays don't converge. Thank you so much! $\endgroup$ Commented Apr 25, 2019 at 15:31
-
3$\begingroup$ @NicSzer Rays near the principal axis do converge approximately to a point and if the mirror is parabolic then all rays parallel to the principal axis will converge to a point. $\endgroup$– FarcherCommented Apr 25, 2019 at 15:47
-
$\begingroup$ @Shub Start a new post - How to prove this for a lens? $\endgroup$– FarcherCommented Oct 8, 2022 at 7:21
-
-
$\begingroup$ @Shub - Thanks and I think that your mirror ray diagram in Desmos is brilliant. Well done! $\endgroup$– FarcherCommented Oct 9, 2022 at 17:15
$\begingroup$
$\endgroup$
A spherical concave mirror does not have a single focus. The focal length depends on the point of reflection. The point at which a light ray, initially parallel to the principal axis intersects the principal axis is $\textbf{defined}$ to be the focus. As the point of reflection approached the center of the lens, the focal length converges to half of radius of the mirror. This can be proved with geometry.
However for a concave parabolic mirror, all light rays parallel to the axis pass through a single focus after reflection and this can indeed be proved (you can find the equation of normal to the parabola at a point and then use the laws of reflection to prove this)
answered Jan 2, 2019 at 16:09
$\begingroup$
$\endgroup$
As you know the image of an object, whose distance from mirror is infinite, form at focus and you must have observed that rays from infinity are all parallel to principal axis. Now your question, if rays are parallel to principal axis then they must pass through focus because they are same as rays coming from infinity. Hope it helps.
$\begingroup$
$\endgroup$
Consider any lens or mirror which is part of a sphere. The focal length is half the radius. Next consider any point on the lens or mirror. The line joining this to the center of curvature is obviously perpendicular to the tangent at that point. Any line joining the focus to this point and one parallel to the principle axis make equal angles with the line joining the center of curvature. Since angles of incidence and reflection are equal, the rays parallel to the principal axis pass through focus and vice versa.
answered Jan 2, 2019 at 16:33
$\begingroup$
$\endgroup$
If you could relate this situation to a parabola, where every point on the parabola is at the same distance from the focus as it is to the directrix. So it seems pretty natural that the focus is the point where all those light rays meet when they reflect off curved curved mirrors.
