Is the band theory valid for an electron with energy greater than the potential?

Question

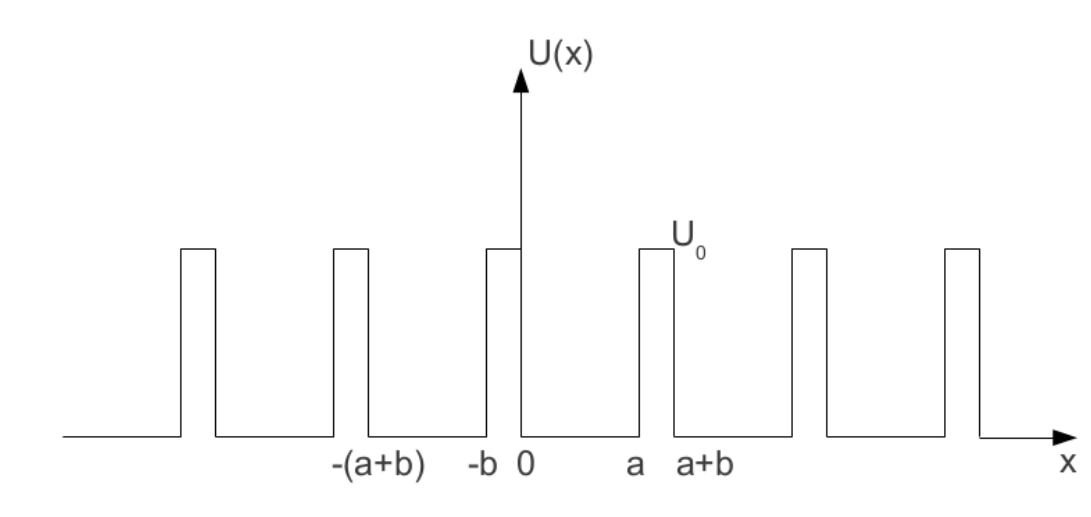
Consider Kroenig Penney model. Here there is a derivation that uses the following potential:

One of the assumption is that the energy of the electron is $E<U_0$. Under this assumption (and others) the following relation is derived:

$$\displaystyle \cos(ka)=\cos(\alpha a)-P{\sin(\alpha a) \over \alpha a}\qquad \left(P={\beta ^{2}ab \over 2}\right)\,\!$$

Which basically explains the band structure of energies of electron in a material.

My question is : what is different in the case where $E>U_0$? In that case is the band theory still valid?

On Alonso Finn textbook the following picture is discussed (it's a periodic potential). It is said that the electron can have different energies, for example E1 E2 and E3. The problem is with E3: I can't understand if it is the case of a metal with electron in conduction band or if it is a different case.

So is the band theory valid for electron with energy E3? Is it simply an electron in conduction band (and therefore following the band theory), or not?

Answer 1

Band theory is valid when $E>U_{0}$. You can repeat the derivation for the case where $E<U_{0}$, but now the wavefunction will be plane wave everywhere instead of being decaying in the barrier.