In a strong interaction, is the total isospin or just its third component conserved? Or are they both conserved?
$\begingroup$
$\endgroup$
Add a comment
|
2 Answers
$\begingroup$
$\endgroup$
6
The third component of isospin is isomorphic to electric charge, which is (so far as we know) an exact symmetry in all systems.
The total isospin is an approximate symmetry of the strong nuclear interaction.
-
$\begingroup$ Weak $W^{+/-}$ flips isospin, right? Anywho, OP's question is restricted to strong interaction. $\endgroup$– MadMaxCommented Sep 28, 2018 at 14:03
-
1$\begingroup$ Weak interactions like $e\to W\nu$ follow more or less the same isospin-conservation rules as strong interactions like $p\to\pi n$ --- even though weak isospin and strong isospin are not quite the same thing. $\endgroup$– rob ♦Commented Sep 28, 2018 at 16:07
-
$\begingroup$ could one come up with an example where total isospin is not conserved in the weak interaction？ $\endgroup$– JinHCommented Feb 3 at 14:53
-
$\begingroup$ @JinH Strong isospin is changed by $∆I=1/2$ in $s\to d$ decays, and by $∆I=1$ in $\pi\to\mu$ decays. If you mean weak isospin, please ask a follow up question; link it here so I'll be sure to notice it. $\endgroup$– rob ♦Commented Feb 3 at 17:16
-
$\begingroup$ @rob thanks, I somehow confused myself, so the isospin of up and down quarks is a completely different quantum number as the isospin of leptons. And of course there are mixing defined by the CKM matrix. $\endgroup$– JinHCommented Feb 3 at 19:14
$\begingroup$
$\endgroup$
1
They are both conserved. Strong interactions conserves all quantum numbers. Electromagnetic interactions doesn't conserve total isospin I, but conserves the third component I3. See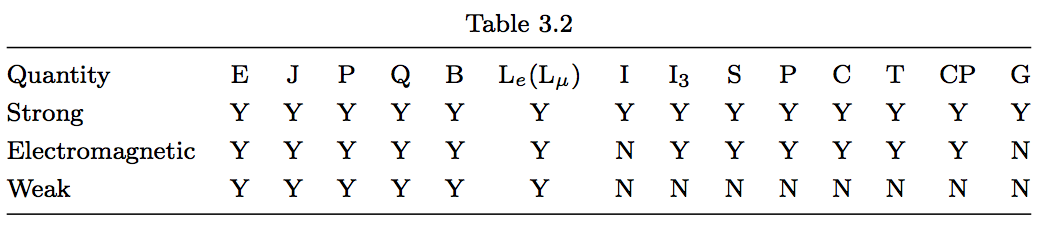 
from an exercise book
-
3$\begingroup$ I'm not sure what you mean by "Strong interactions conserves all quantum numbers." For example, it doesn't conserve the sum of the intrinsic spins. Please say what book this is from. Cutting and pasting stuff on the internet without attribution is rude. $\endgroup$– user4552Commented Oct 21, 2018 at 14:36