I am making the attempt to develop some computational models of Star systems and I was wondering how the a torus shaped planet would compare to a traditional spherical planet in the context of rotational and orbital motion.
$\begingroup$
$\endgroup$
7
-
$\begingroup$ This reminds me of this video youtu.be/UrLey-pX7Bc $\endgroup$– BioPhysicistCommented Sep 16, 2018 at 0:56
-
1$\begingroup$ see io9.gizmodo.com/… $\endgroup$– anna vCommented Sep 16, 2018 at 3:45
-
4$\begingroup$ I really wonder why this is not regarded as mainstream physics, since point masses moving in classical fields is about as mainstream as anything can be. And there is a biggish literature in astrophysics on orbits related to massive torii in AGNs, so torus shaped mass distributions are also mainstream. $\endgroup$– Anders SandbergCommented Sep 16, 2018 at 20:15
-
4$\begingroup$ I think this is not non-mainstream but is too broad as it's basically asking us to re-derive a significant portion of Newtonian mechanics in a different gravitational system. Even the answer given basically says, "there are changes, take a look at my blog" where the post is quite lengthy. $\endgroup$– Kyle KanosCommented Jan 28, 2019 at 11:19
-
3$\begingroup$ Related meta post: physics.meta.stackexchange.com/q/11037 $\endgroup$– user191954Commented Jan 28, 2019 at 12:35
|
Show 2 more comments
1 Answer
$\begingroup$
$\endgroup$
2
To make a ring-shaped planet it needs to rotate very fast to counteract the gravitation trying to crush it into a sphere. There is a fairly big literature on such rings, with Dyson's papers(1893) as the locus classicus. See the introduction of this paper for more references. I did some (admittedly crude) simulations of a torus planet myself back in 2014.
The main issues affecting the question is (1) The effective surface gravity varies along the surface (the surface remains at the same potential): it is higher along the "poles" and lowest along the "equators" on the inside and outside. (2) The torus is fairly elliptical. (3) there is a high rotation rare, and this will produce Coriolis acceleration.
Near the surface and for short distances projectile motion will look fairly terrestrial. Thrown things follow parabolas. As the trajectories get longer the above effects will matter. The Coriolis acceleration $\mathbf{a}=2\mathbf{v}\times\mathbf{\Omega}$ will tend to tilt and twist the trajectory. Along the poles $\mathbf{\Omega}$ and gravity as aligned, and the trajectory will be tilted and twisted. Closer to the equators the twisting gets more asymmetric.
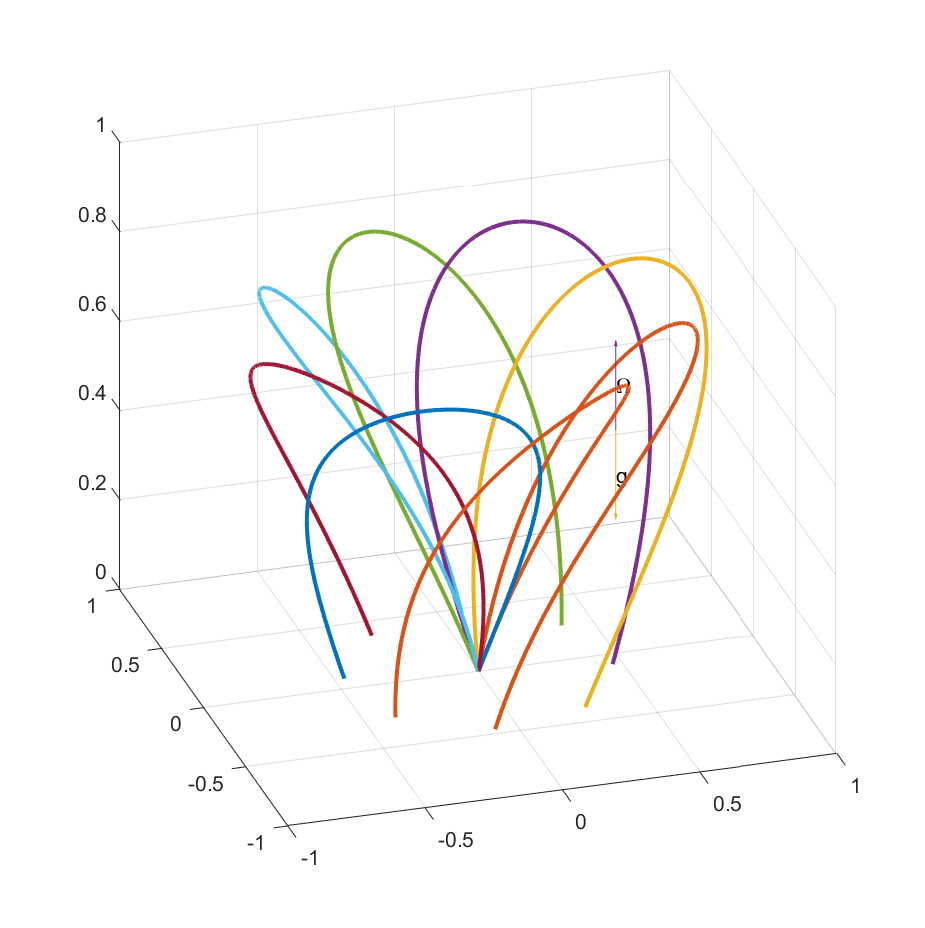
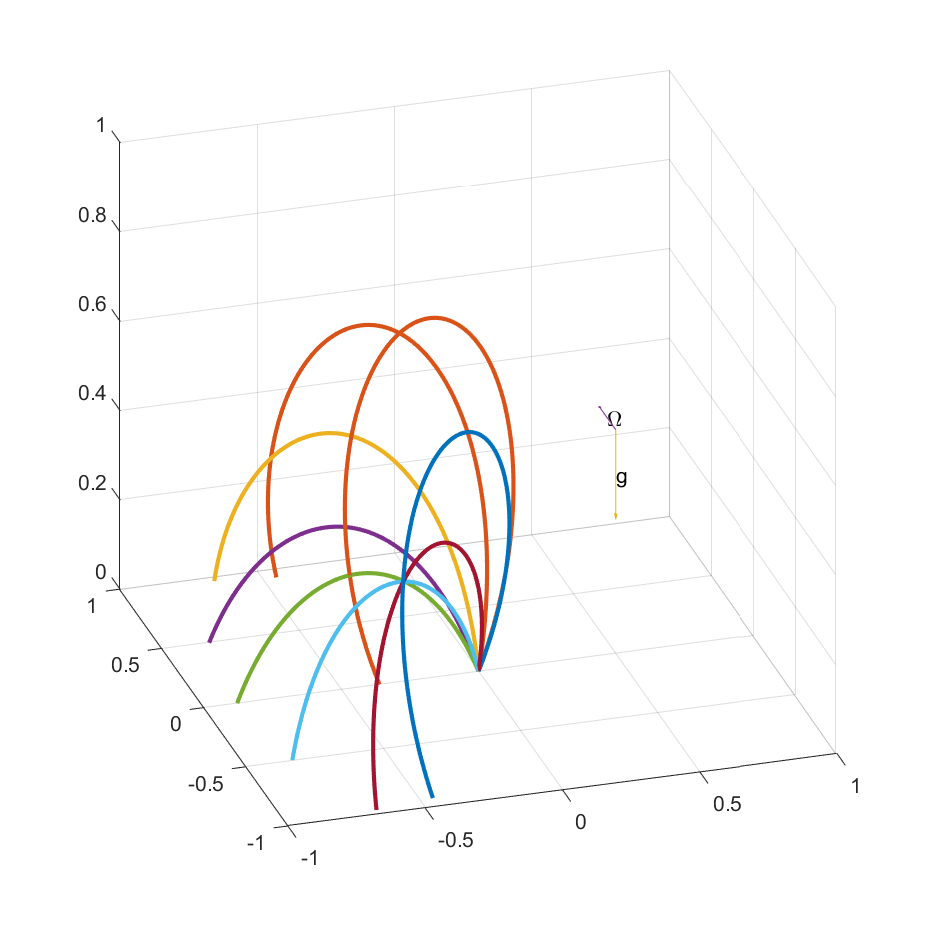
For even longer trajectories the other factors will further twist the trajectory, plus the ground is no longer flat. There will be different gravitational acceleration in different points, and so on. In this range the trajectories are fairly hard to describe.
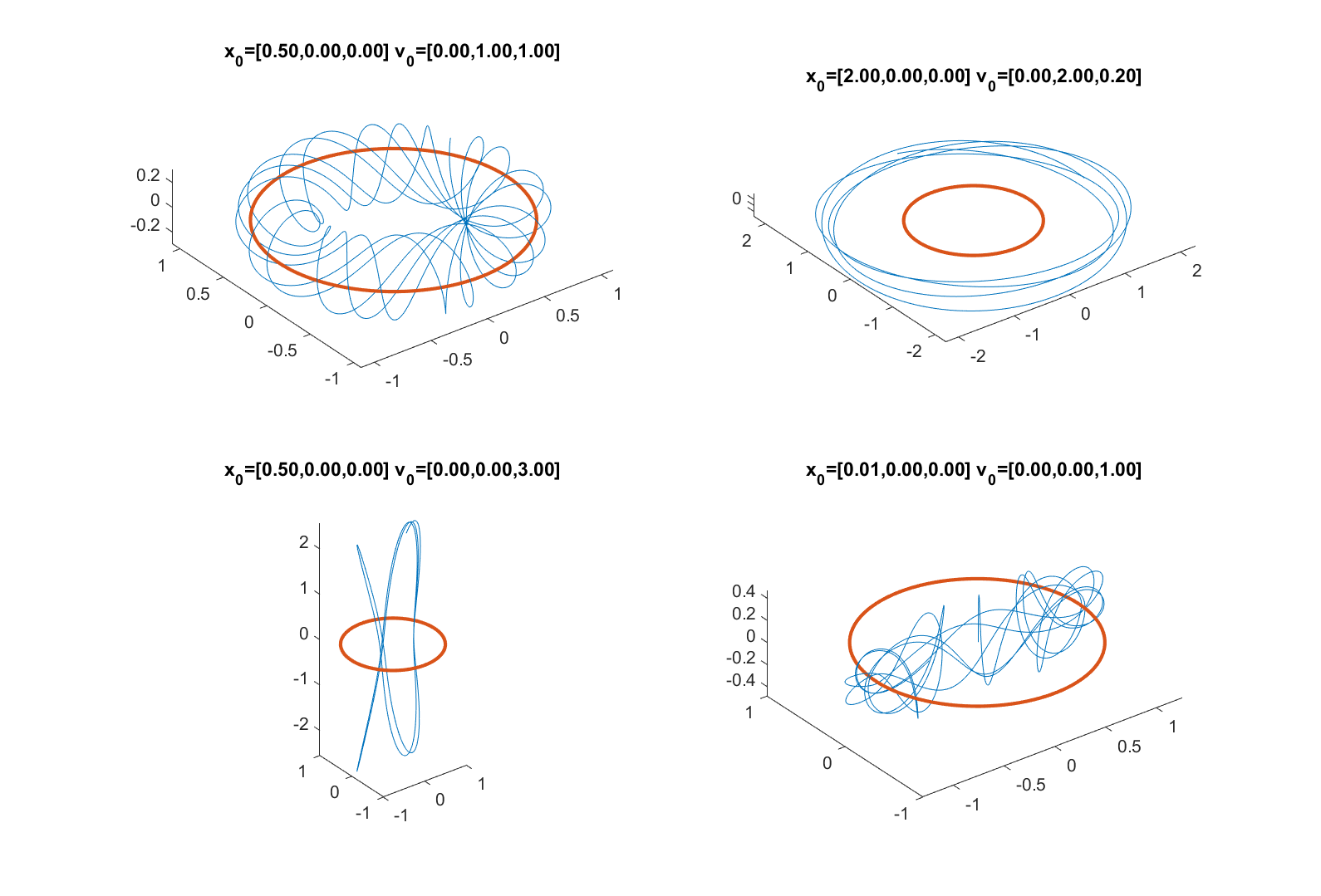
For higher trajectories we get counterparts to satellite orbits. Obviously there are equatorial orbits. The flattened mass distribution induces precession of elliptic orbits. This gets more extreme for tilted orbits. There are also orbits through the hole, and orbits that bob back and forth through the hole in chaotic ways. See the plots in this post or below.
The key thing to note is that gravity is always attractive but a ring produces a field that attracts towards the ring so the centre point is an unstable fixed point: mass is attracted towards the equatorial plane, but pulled outward towards the ring.
answered Sep 16, 2018 at 6:22
-
1$\begingroup$ @PM2Ring - Thanks! I also have some more detail on the ball trajectories on my blog about this, aleph.se/andart2/physics/throwing-balls-on-torus-earth - it turns out one can view the trajectory as an electron in a combined electric and magnetic field, and decompose it into a helix plus a drift velocity. Granted, the effect is small for reasonable trajectories. $\endgroup$ Commented Sep 17, 2018 at 20:08
-
$\begingroup$ not as interesting (or likely?) as a torus, but related: Could you stably orbit around a square (cubic) body? Would the orbit destabilize automatically if not corrected by input? $\endgroup$– uhohCommented Jan 30, 2019 at 0:12