1
$\begingroup$

I have a question, if there is a straight beam of light that moves at the speed of light, is there a way to calculate the speed of bent light? since bending light causes it to lose momentum?

Example, with Atmospheric Refraction where the atmosphere causes light to bend as light passes through material.

Improve this question
$\endgroup$
1
  • $\begingroup$ Bending the light changes the direction of its momentum but not the magnitude of its momentum. The speed of the light is always $c$. $\endgroup$
    – John Rennie
    Commented Jul 18, 2018 at 15:09

2 Answers 2

Reset to default
2
$\begingroup$

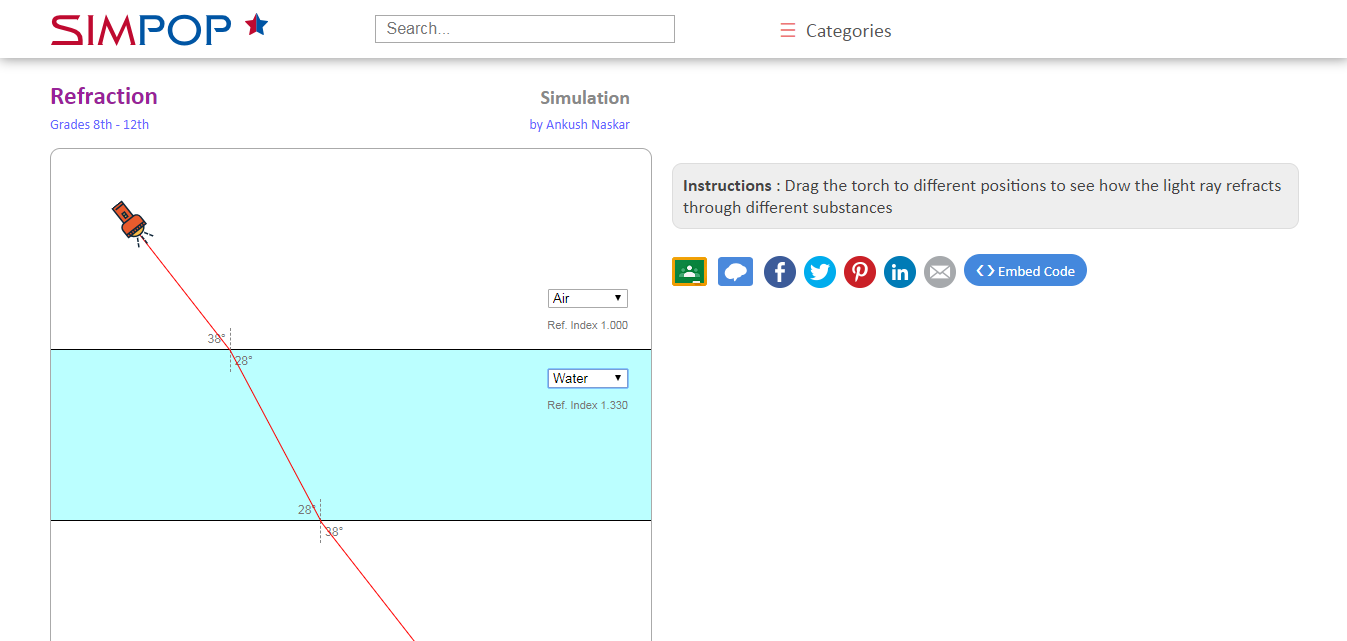
It depends on what is causing the bending. Let's assume you mean the light beam is being bent due to it being $refracted$. If the light beam begins in vacuum and then travels into a material with index of refraction, $n$, then the speed of the light beam will change. That is, assuming the frequency of the light is constant across the barrier, the refraction of the light changes the light's wavelength which changes its speed, $v$, according to $$ v = f*\lambda. $$

Or more simply, one can view it via, $$ v = c/n $$ where $n$ is the index of refraction of the material. You can see that in vacuum, $n = 1$.

I strongly disagree with John Rennie's comment above: he displays a common misconception! The speed of light is NOT "always" $c$. The quantity $c$ is the speed of light in vacuum specifically. When it's not in vacuum, the light beam is traveling at $v < c$. The light beam changes direction precisely because it's traveling at a smaller velocity while at the same frequency.

For some typical indices of refraction: https://www.google.com/search?q=vacuum+index+of+refraction&ie=utf-8&oe=utf-8&client=firefox-b-1-ab

Check out an introduction to optics book, I suggest the one by F. and L. Pedrotti.

Also, play aroud with this PHET simulation. https://phet.colorado.edu/en/simulation/bending-light

Improve this answer
$\endgroup$
10
  • $\begingroup$ Thanks, and what about Atmospheric Refraction, would that still apply to the equations you shown? en.wikipedia.org/wiki/Atmospheric_refraction like do each layer of the atmosphere have a higher refraction value? $\endgroup$
    – C. Jordan
    Commented Jul 18, 2018 at 16:37
  • $\begingroup$ Yes, absolutely! As that wiki article says, the index of refraction decreases as the air density decreases. So since the atmosphere's density decreases with increased elevation, then the light is being bent differently in each atmospheric layer (less and less bending as one goes higher in the atmosphere). $\endgroup$
    – Daddy Kropotkin
    Commented Jul 18, 2018 at 17:31
  • $\begingroup$ This principle also works in fiber optic cables, where traditional ones have a couple of layers (<en.wikipedia.org/wiki/Core_(optical_fiber)>), as well as more interesting ones like graded-index fibers (<en.wikipedia.org/wiki/Gradient-index_optics>) that have a gradient of index of refraction! $\endgroup$
    – Daddy Kropotkin
    Commented Jul 18, 2018 at 17:31
  • $\begingroup$ The links are broken, but thank you again. $\endgroup$
    – C. Jordan
    Commented Jul 18, 2018 at 20:01
  • $\begingroup$ en.wikipedia.org/wiki/Core_(optical_fiber) , en.wikipedia.org/wiki/Gradient-index_optics $\endgroup$
    – Daddy Kropotkin
    Commented Jul 18, 2018 at 21:54
1
$\begingroup$

The speed of light depends on the medium it refracts through. Consider the following equations -

n = c/v

v = c/n

(n is the index of refraction for medium, c is the velocity of light in vacuum, v is velocity of light in medium)

I have created a Light Refraction Simulation that will help you visualize and understand the concept better.

enter image description here

Improve this answer
$\endgroup$
2
  • $\begingroup$ It's not clear how this answers the question. $\endgroup$
    – ZeroTheHero
    Commented Sep 2, 2018 at 17:58
  • $\begingroup$ The question asks for the speed of light in a medium (refracted light). I have answered it by the equation. $\endgroup$
    – Animan
    Commented Sep 3, 2018 at 3:54

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.