Can someone provide me an intuitive explaination to uniqueness theorem in electrostatics?

Question

So far I understood that in one dimension Laplace equation gives out a simple equation $V(x)=mx+b$ which is because if you differentiate this equation two times you will get 0 which is Laplace identity.

After this it says(Griffiths Electrodynamics) thus we need two boundary conditions What does this mean?

In my understanding, $V_1$ and $V_2$ are two solutions two the Laplace equation for potential. And thus$\nabla^2 V=0$ is satisfied by thee. Then it takes potential at a third point which is the difference of $V_1$ and $V_2$ and thus taking Laplacian of $V_3=V_1-V_2$ gives us zero and thus $V_3$ also satisfies the Laplacian and after than whatever he has written I am sorry I don't understand?

Whats the sole purpose of Uniqueness Theorem and Boundary conditions, where and how do we use it?

I went through Rochester and Ph Texas online notes got nothing

Then it provides this proof

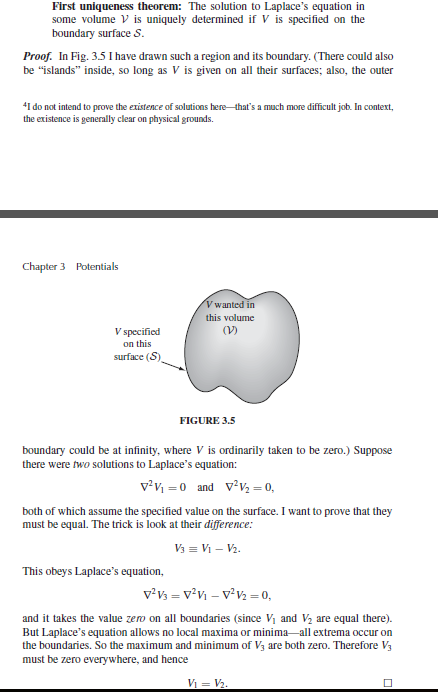
He says there may be islands inside what does that mean?

Answer 1

In an intuitive sense, the uniqueness theorem says that the solution to the Poisson equation is unique - which means once you have a $V$, no matter how you get it, and it solves the equations and the boundary conditions, that is the solution you are looking for.

I will give a more detailed response to your other questions soon.