Case 1 ($O_1$):
We have wire which has current $I$, in this condition the wire is neutral, and we have a charged particle $+q$
I am put them as following.
There will be no attraction since wire is neutral and particle is not moving relative to wire. (and for simplicity we can say wire and $+q$ is stationary to the ground reference system (let say $O_1$))
Case 2($O_2$): Now we are taking this system inside a train, and the train then moves with constant velocity $v$. $O_2$(observer 2) sees this motion outside the train stationary relatively to the ground.
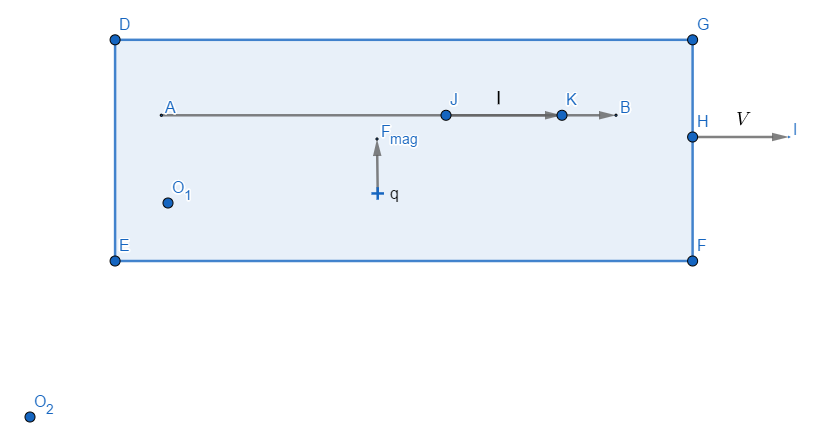
$O_2$ observes that there must be magnetic force between wire and particle $+q$ since particle is moving and there is current in the wire.
Since there is nothing different for $O_1$, there must be no net force between wire and particle, and since $O_2$ outside train sees magnetic force there must be a force equalize the magnetic force for $O_2$.
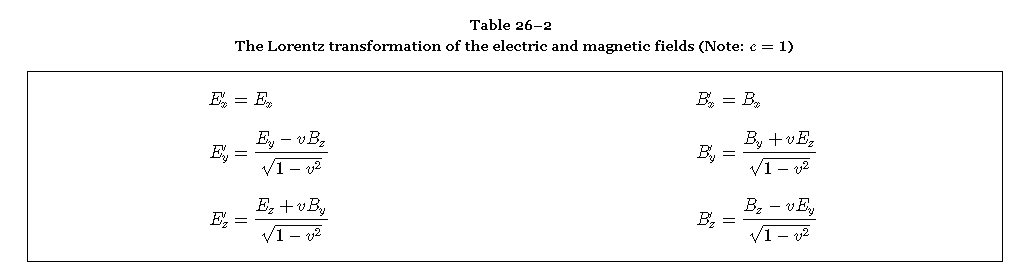
Question: I do not understand what this equalizer force should be and why this occurs? I thought it must be electric force, but how electric force occurs although it was a neutral wire. In addition, the wire and the whole train will contracted due to Lorentz Transformations. However, how this contraction causes a breaking of a symmetry in current density (or something else related to it because I do not know ).
