How are the dark matter (DM) thermalization rates derived in singlet DM model?

Question

This well-cited paper talks about a minimal renormalizable extension to the Standard Model (SM) to incorporate particle dark matter (DM) into it by adding a real scalar field $S$ which (unlike the Higgs doublet $H$) is a singlet under the full SM gauge group. But we have to pay a price, we have to introduce three more free parameters in the theory (in addition to those already present in the Standard model): (i) the mass of the new scalar $m_0$, (ii) dimensionless self coupling of the scalar $\lambda_S$, and (iii) a dimensionless coupling to the Higgs $\lambda$.

The power-counting renormalizable Lagrangian of the model, is therefore, $$\mathscr{L}=\mathscr{L}_{\rm SM}+\frac{1}{2}(\partial_\mu S)^2-\frac{1}{2}m_0^2S^2-\frac{1}{4}\lambda_SS^4-\lambda S^2({\rm H^\dagger H})\tag{1}.$$

Question 1 It is assumed that the DM was in thermal equilibrium at temperatures of the order $m_0$ and above. Is this an ad hoc assumption? Why did the authors have to make this assumption?

Question 2 It is also assumed that the thermalization rate varies as $\sim \lambda^2T$ for $T\gg m_h$ and $\sim \lambda^2T^5m_h^{-4}$ for $T\ll m_h$ where $m_h$ is the Higgs mass. How do they derive these thermalization rates?

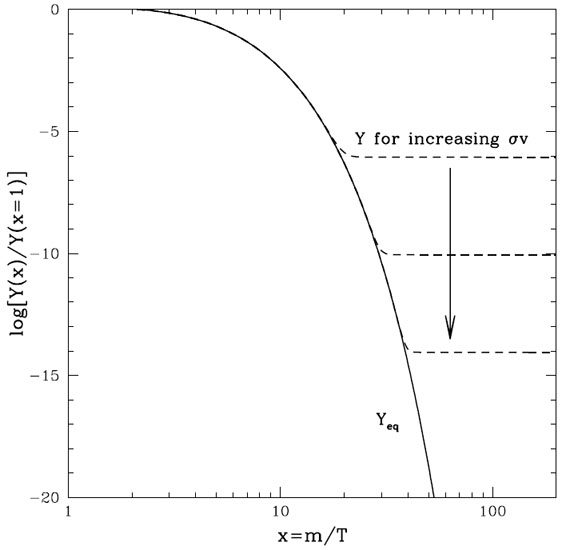
All I know is that the dark matter density evolves as the figure below (taken from here.)

Answer 1

I'm no expert in this field and I would love to be corrected by others. This is also the answer to question 1. But here is what my heuristic understanding is. It can be argued that if a dark matter species $\xi$ continued to remain in thermal equilibrium until the present, its abundance $$\frac{n_{\xi}}{s}\sim \Big(\frac{m_\xi}{T}\Big)^{3/2}e^{-m_{\xi}/T}$$ would have been negligible and contrary to the observation we wouldn't find any appreciable relic abundance. Therefore, the dark matter species $\xi$ must at some point of time (or temperature) had decoupled from the cosmic thermal bath. To achieve the desired relic density, the $\xi$ should freeze-out at a temperature not much less than the dark matter mass $m_{\xi}$. Therefore, it is reasonable to assume that for temperatures $\sim m_{\xi}$ and above $\xi$ was in equilibrium. The first dashed line in the figure tells that freeze-out occurs at a temperature around $\frac{m_{\xi}}{20}$, and remained in equilibrium around $T\sim m_{\xi}$ and above. If instead, we assume that the dark matter departs from equilibrium at a scale $\frac{m_{\xi}}{30}$, its abundance will decrease compared to what it had been when the departure scale was $\frac{m_{\xi}}{20}$.