How SUSY solves the hierarchy problem?

Question

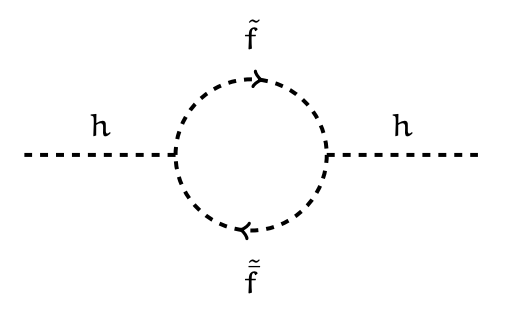
I am struggling to understand the argument for why the introduction of a stop in SUSY can solve the hierarchy problem. The quadratic divergence from the top loop in the higgs mass calculation gives a contribution of $$ \delta m_h^2 = - \frac{|y_f|^2}{16\pi^2} \left[2\Lambda^2 + \ldots \right] $$ If we introduce a scalar $S$, there should be two additional diagrams to consider:

and

The first diagram should give a contribution like

$$ \delta m_h^2 = \frac{\lambda_S}{16\pi^2} \left[\Lambda^2 + \ldots \right] $$

and the second like

$$ \delta m_h^2 = \frac{|\lambda_S|^2}{16\pi^2} \left[\Lambda^2 + \ldots \right] $$

(I am probably off by some constants or something). Now, this seems to me to only cancel out of $y_f = \lambda_S = 1$, which is close to 1 for the top but not exact. Isn't this just another unnatural fine tuning problem?

Answer 1

You are considering three loop diagrams with three couplings: $g_1$ for the Higgs-top Yukawa coupling, $g_2$ for the $h h f \tilde{f}$ coupling, and $g_3$ for the $h f \tilde{f}$ coupling. When you do the loop diagrams, the contribution is schematically $-g_1^2 + g_2 + g_3^2$.

You've pointed out that this is not zero for general values of $g_1$, $g_2$, and $g_3$, but of course it isn't! Without further structure, these couplings have nothing to do with each other. The point of supersymmetry is that it does relate the couplings -- and if you work through the calculations in detail, it relates $g_1$, $g_2$, and $g_3$ in exactly the way needed to make the $O(\Lambda^2)$ part of the loop integrals cancel out.