6
$\begingroup$

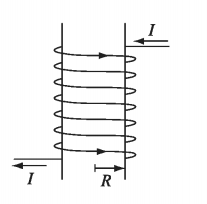
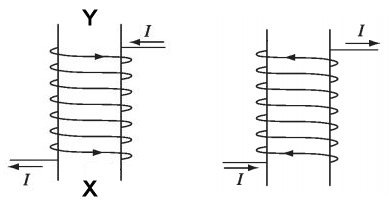
I am reading Griffiths' electrodynamics book, and couldn't understand his argument for the absence of the radial component of the magnetic field of a long solenoid (See figure). Below I quoted his argument:

enter image description here

"First of all, what is the direction of B? Could it have a radial component? No. For suppose $B_s$ were positive; if we reversed the direction of the current, $B_s$ would then be negative. But switching current is physically equivalent to turning the solenoid upside down, and that certainly should not alter the radial field."

As far as I understand, I don't see any reason why the radial component of the magnetic field would not be altered by turning the solenoid upside down!

In fact, I find his argument contradictory: if turning the solenoid upside down is equivalent to switching the current direction, then it makes sense that turning it upside down should reverse the radial component of the magnetic field, as switching the current direction does.

Could you explain to me why the radial component of the magnetic field is $0$ in the context of Griffiths' argument?

Improve this question
$\endgroup$

2 Answers 2

Reset to default
7
$\begingroup$

Suppose that you were in a room and standing at position $X$ with a solenoid on a table in front of you as shown in the left hand diagram.

enter image description here

You measure a magnet field due to the solenoid coming out of the screen.

You now go out of the room and see that the solenoid is as per the right hand diagram.

There are two possibilities as to what has happened:

  1. You are now standing at position $Y$ and the direction of the current has not been changed.

  2. You are now standing at position $X$ and the direction of the current has been reversed.

There is no way that you can tell which of $1$ or $2$ has occurred.

If it was option $1$ then when you measured the radial magnet field it would be coming out of the screen but if it had been option $2$ then the radial magnetic field would have been out of the screen.

Instead of moving position the solenoid could have been rotated through $180^\circ$ but either way you cannot have two possible results dependent on whether the apparatus was "rotated" or the current reversed when visually the situation is the same.

Improve this answer
$\endgroup$
2
$\begingroup$

The argument is perfectly justified.

For your understanding, what makes you think radial component changes on turning solenoid upside down? If radial component is radially outward, turning solenoid will not change that right?

On the other hand, reversing the direction of current would mean reversing the direction of magnetic field, so if it had a component radially outward, reversing current direction will mean a radially inward component.

You see from here both the arguments are justified, the only way to resolve them both is to have no radial component of magnetic field.

Improve this answer
$\endgroup$
3
  • $\begingroup$ This is my biggest issue: why turning the solenoid upside down will not reverse the direction of the radial component? Do we just "know" it by our intuition and the symmetry of the set up? $\endgroup$
    – A Slow Learner
    Commented Feb 12, 2018 at 5:27
  • $\begingroup$ How could turning the solenoid upside down change the radial component? Some kind of interaction with gravity? $\endgroup$
    – Ben51
    Commented Feb 12, 2018 at 6:31
  • $\begingroup$ "why turning the solenoid upside down will not reverse the direction of the radial component", this must be related to the symmetry of the space. Suppose you are in the deep space without anything around you. "turning the solenoid upside down" is equivalent to turning yourself, the observer, upside down. Should that reverse the direction of the radial component? I guess not. $\endgroup$
    – verdelite
    Commented May 15, 2019 at 15:15

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.