Magnetic field outside an infinite solenoid carrying time varying current

Question

I know that this short of question has already been asked a fair amount of time, but I could not find one matching the details I am looking for.

Consider we have an infinitely long solenoid. It is carrying a current, that is, the same current is flowing through each turns of it. And we are told that this current is not a steady current, rather it is varying with time. It may be like: $I \propto \sin(\omega t),\space I\propto t^n$ (with $n$ positive or negative), etc.

Now we can easily calculate the magnetic field inside the solenoid (radius $R$), using the same procedure as for steady current. That is \begin{equation} \oint_l \vec{B}\cdot\vec{dl}=\mu_0\cdot I_{enclosed} \space \space \implies B\cdot L = \mu_0\cdot nL\cdot I(t) \space \space \implies \vec{B}=\mu_0 nI(t)\hat{z} \space \space \space \space \space \space \space (r\lt R ) \end{equation}

And magnetic field everywhere outside the solenoid is obviously zero.

Now what may happen if the current starts varying with time?
First of all, magnetic field inside will start varying with the current. As before only $B_z$ component will remain, $B_r$ and $B_{\phi}$ components will vanish considering symmetry.

But what happens with the magnetic field outside ($r\gt R$) the solenoid?
Previously, in steady current case, $B_r=B_{\phi}=B_z=0$. Now what will happen to $B_r,$ $B_{\phi},$ $B_z$ outside the solenoid?

I think that since the solenoid is infinite magnetic field outside it should be zero again.
What do you say?

Answer 1

Now what may happen if the current starts varying with time? ... But what happens with the magnetic field outside ($r>R$) the solenoid?

It depends on how the current changes in time.

In general, induced electric field is proportional to acceleration of charges that generate it.

As long as the current is a linear function of time, induced electric field in the region close to the solenoid does not change in time and has zero curl. The region is defined as the outside of the solenoid and inside the surface defined by the light wavefront generated at the first instant when the current started changing linearly in time (a far away cylindrical surface).

Electric field that has zero curl implies magnetic field there is constant in time. It need not be zero, but it must not change in time, thus it is at most some static magnetic field due to some distant sources. If we assume there are no such sources, magnetic field outside has to vanish.

If the current does not change linearly, acceleration of charges changes in time, and thus induced electric field outside is not constant in time, but changes in time. From Maxwell's equation

$$ \nabla \times \mathbf B = \mu_0 \epsilon_0 \frac{\partial \mathbf E}{\partial t} $$

it follows that magnetic field outside has nonzero curl in the angular direction, and thus magnetic field in the axial direction cannot vanish.

Answer 2

Let's review the traditional argument that the magnetic field vanishes outside an infinite solenoid. Suppose the solenoid's axis is the z-axis and the radius is $a$. Let $r > a$, and consider a rectangular Ampèrian loop that lies in the xz-plane, where two edges are parallel to the z-axis, one located at $x = a$, the other at infinity. The other two edges are perpendicular to the z-axis. There is no enclosed current, and since the field is parallel to the z-axis, the contribution of the perpendicular edges to the line integral is zero. The contributions from the two edges parallel to the z-axis must cancel. The field at infinity must vanish, so it follows that the field at $r$ must also vanish.

Notice that this argument fails to hold when the current is non-steady. Consider a circular loop of radius $r > a$ centered at the origin and lying in the xy-plane. If the current is non-steady, the magnetic flux through this loop may be varying over time, which by Faraday's law implies the presence of a circumferential electric field at $r$. (Symmetry arguments still imply that the electric field has no radial or longitudinal component.) When applying Ampère's law to a loop described in the previous paragraph, although there's no enclosed current, there is an enclosed time-varying electric field, which (by Maxwell's correction to Ampère's law) functions similarly to a current. This implies that the $z$ component of the magnetic field is not independent of $r$. (Symmetry arguments still imply that the magnetic field is purely longitudinal.)

In addition, because the magnetic dipole moment of a solenoid is given by $\mu = NIA$, where $A = \pi a^2$ is the cross-sectional area of the solenoid, it, too, is time-varying, and this allows us to predict that energy is radiated away from the solenoid in the form of electromagnetic waves (so we expect the fields to oscillate like $\sin(kr - \omega t)$, where $k = \omega/c$). The rate at which energy is escaping an enclosing cylindrical boundary at radial coordinate $r$ is given by the surface integral of the Poynting vector $S$, and as $r \to \infty$, this surface integral shouldn't vanish (since the energy escapes to infinity). Since the area of this cylindrical surface goes like $r$, the asymptotic behaviour of $S$ must go like $1/r$. This in turn implies that the magnitudes of $E$ and $B$ outside the solenoid go like $1/\sqrt{r}$. Furthermore the magnitudes of the radiation fields in general should be proportional to the second time derivative of the dipole moment, i.e., $NIA\omega^2$.

This gives us a pretty good idea of what the fields outside the solenoid look like at large distances, i.e.

\begin{align} B(r, t) &\approx \frac{NIa^2\omega^2}{\sqrt{r}} \Re(C_1 e^{i(kr-\omega t)}) \hat{z} \\ E(r, t) &\approx \frac{NIa^2\omega^2}{\sqrt{r}} \Re(C_2 e^{i(kr-\omega t)}) \hat{\theta} \end{align}

where $C_1, C_2$ are constants. (These constants might still have some residual dependency on the parameters of the system.) But based on this analysis we still have no idea what the fields are like near the solenoid (other than the fact that the magnetic field is longitudinal and the electric field is circumferential).

For that, refer to the the exact solutions for $E$ and $B$, which are given by J. D. Templin, Am. J. Phys. 63, 916 (1995) and involve Bessel functions.

Answer 3

Properties of electric and magnetic inductance tell us that a changing magnetic field causes an electric field around it and a changing electric field causes magnetic field around it. Just like magnetic fields, induced electric fields are continuous: they can spread out to where they are no longer noticed, but they cannot actually end. Only electric fields joining electric charges can start and end at specific points.

The symmetry of a solenoid (if we stay away from the ends) allows only circular fields around the axis and linear fields parallel to the axis. The current produces a very strong axial magnetic field within the solenoid and almost zero outside the solenoid. If the solenoid is thought of as having essentially infinite length, then the outside field is zero. When the current and magnetic field change, circles of electric field are produced around the axis. This electric field is physically what pushes against the wire to slow the change of current and the change of magnetic field, properties of an inductor. If the solenoid were a perfect conductor, then the smallest electric field would be more than enough to stop the change. If not a truly perfect conductor. then some electric field could exist in circles around the solenoid, but not noticeably large. If there is changing circular electric field outside the solenoid, then we could consider an Ampere loop around a portion of this field but completely outside the solenoid. The inner and outer sides of the loop would be parallel to the axis. There would have to be some axial magnetic field induced by this changing electric field.

If we call the solenoid a perfect conductor, there is no magnetic field outside. If we do not, there may be a small amount of axial magnetic field outside.

Answer 4

Multiplying the equation what I wrote here by the time harmonic oscillation factor $e^{-i\omega t}$, I believe the analytical solution in vector potential form is given by $$ A_{\theta}= \left\{ \begin{alignedat}{2} & \frac{1}{2}B_z^0re^{-i\omega t} &\quad& \text{for }r\leq R \\ & \frac{1}{2}\left(B_z^0R\right)\frac{R}{r}e^{-i\omega t} & & \text{for } R<r \end{alignedat} \right. $$ From this solution, we obtain $\vec{B}=\vec{0}$ and $\vec{E}=-\frac{\partial \vec{A}}{\partial t}\neq \vec{0}$ outside the solenoid.

Some preliminaries (EDIT; below added on Tue Feb 7 2023)

When writing formulas here, we use cylindrical coordinates. To investigate the time-varying infinite solenoid problem, the vector potential and scalar potential formulation, that is $(\mathbf{A},\phi)$ formulation, is used to describe it. If axis symmetry is maintained, the scalar electric potential disappears, because $\phi(\theta_A)=\phi(\theta_B)$ if $\theta_A\neq\theta_B$. Thus only $\mathbf{A}$ is the freedom variable to solve for. And the Coulomb gauge condition $\text{div}\mathbf{A}=0$ is taken into account. It is important to note that the displacement current is not taken into account here.

Static infinite solenoid

First we consider a static solenoid of infinite length. But before we go any further, another different problem will be examined, since 2 types of problems are similar in their mathematical formulations, if one type is verified, the other is straightforward.

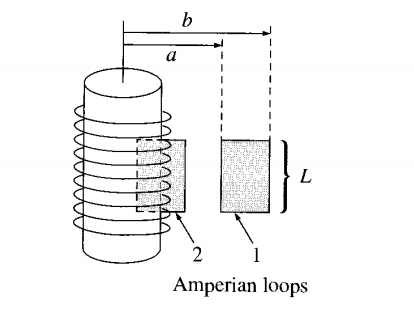
Let us review Ampere's law for a conducting cylinder of finite size with a uniform current flowing through its cross-section;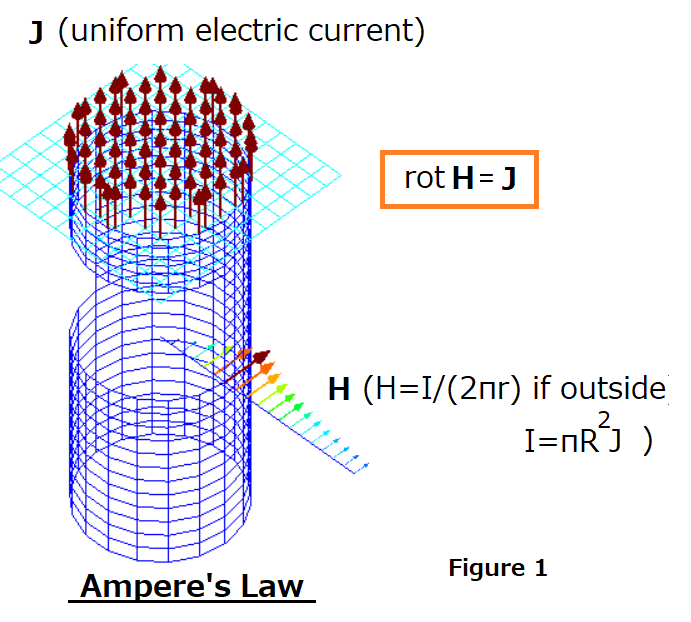 this is the 2nd type of problem. The problem and result are summarized in the figure:

Now let us illustrate a similar figure relating to a static infinite solenoid (this is the 1st primary problem). The problem and result are summarized in the figure: 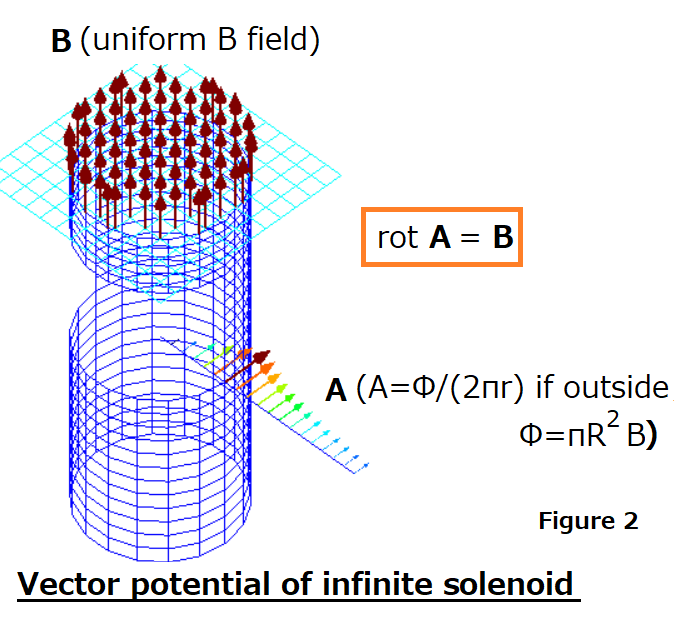As stated above, these 2 problems are similar. Therefore, similar math expressions exist as shown in the figures.

I emphasise important differences between them here.

• $\mathbf{J}$ and $\mathbf{H}$ are observables, and $\mathbf{B}$ is observable but $\mathbf{A}$ is NOT observable.
• $\mathbf{J}$ and $\mathbf{A}$ are vectors while, $\mathbf{H}$ and $\mathbf{B}$ are pseudo-vectors.

Time harmonic varying infinite solenoid

The equation for a time-harmonic oscillating infinite-length solenoid, which I claim to be the solution, was given at the beginning of this note. Here it is verified that it does indeed satisfy Maxwell's equations.

Since the system is axis symmetric, then \begin{align*} \text{rot}\mathbf{A} &= \left(\frac{1}{r}\frac{\partial A_z}{\partial\theta} -\frac{\partial A_{\theta}}{\partial z},\; \frac{\partial A_r}{\partial z}-\frac{\partial A_z}{\partial r},\; \frac{1}{r}\left\{\frac{\partial (rA_{\theta})}{\partial r}-\frac{\partial A_r}{\partial \theta} \right\}\right) \\ &= \left(-\frac{\partial A_{\theta}}{\partial z},\;0,\; \frac{1}{r}\frac{\partial (rA_{\theta})}{\partial r}\right) = \left(0,\;0,\;\frac{1}{r}\frac{\partial (rA_{\theta})}{\partial r}\right), \end{align*} thus, \begin{align*} \mathbf{B}_z&=\left(\text{rot}\mathbf{A}\right)_z= \left\{ \begin{alignedat}{2} & B_z^0e^{-i\omega t} &\quad& \text{for }r\leq R \\ & 0 & & \text{for } R<r \end{alignedat} \right. \\ &= B_z^01(R-r)e^{-i\omega t}, \end{align*} where $1(x)$ is the Heaviside step function, defined as $1(x) = 1\text{ for }x>0\text{ and } 1(x) = 0\text{ for }x\le 0$ and $A_{\theta}(r)$ written at the begininng of this note substituted. Now let us proceed to the calculation of $\text{rot}\mathbf{H}$. Magnetic permeability within the solenoid coil is assumed to be isotropic and constant, that is, it is given as $\mu(r)=\mu_{\text{rod}}1(R-r)$, where $\mu_{\text{rod}}$ is the constant permeablity in the solenoid rod. We can get the magnetic H field as follows: \begin{align*} \mathbf{H}_z=\frac{1}{\mu(r)}\mathbf{B}_z= \frac{1}{\mu(r)}B_z^01(R-r)e^{-i\omega t} =\frac{1}{\mu_{\text{rod}}1(R-r)}B_z^01(R-r)e^{-i\omega t} =\frac{1}{\mu_{\text{rod}}}B_z^01(R-r)e^{-i\omega t} \end{align*} As seen this result, the magnetic field is confined within the solenoid.

The next task is to look for the electric current in this system. Since \begin{equation*} \text{rot}\mathbf{H} = \left(\frac{1}{r}\frac{\partial H_z}{\partial\theta} -\frac{\partial H_{\theta}}{\partial z},\; \frac{\partial H_r}{\partial z}-\frac{\partial H_z}{\partial r},\; \frac{1}{r}\left\{\frac{\partial (rH_{\theta})}{\partial r}-\frac{\partial H_r}{\partial \theta} \right\}\right) \\ = \left(0,\;-\frac{\partial H_z}{\partial r},\;0\right), \end{equation*} we get \begin{equation*} \left.\mathbf{J}\right|_{\theta}= \left.\text{rot}\mathbf{H}\right|_{\theta}= -\frac{\partial H_z}{\partial r} = -\frac{\partial}{\partial r}\left(\frac{1}{\mu_{\text{rod}}}B_z^01(R-r)e^{-i\omega t}\right) = \frac{1}{\mu_{\text{rod}}}B_z^0\delta(r-R)e^{-i\omega t}. \end{equation*} That is, the surface current densitiy $\left.\mathbf{J}\right|_{\theta}$ on the solenoid rod surface is calculated.

The space electric fields can be obtained via $\mathbf{E}=-\text{grad}\phi-\frac{\partial \mathbf{A}}{\partial t}$ : \begin{equation*} \mathbf{E}_{\theta}= -\frac{\partial A_{\theta}}{\partial t} = \left\{ \begin{alignedat}{2} & \frac{i\omega}{2}B_z^0re^{-i\omega t} &\quad& \text{for }r\leq R \\ & \frac{i\omega}{2}\left(B_z^0R\right)\frac{R}{r}e^{-i\omega t} & & \text{for } R<r \end{alignedat} \right. \end{equation*} As seen this result, the electric field outside the solenoid is not zero.

Thus the math form $\mathbf{A}$, given at the beginning of this note, satisfies 3 of Maxwell's equations provided the displacement current is neglected, i.e., $\text{rot}\mathbf{H}=\mathbf{J}$, $\text{rot}\mathbf{E}=-\frac{\partial\mathbf{B}}{\partial t}$ and $\text{div}\mathbf{B}=0$ are satisfied.

Some comments

It is very interesting that vector $\mathbf{A}$, expressed with the function $A_{\theta}\sim\frac{1}{2}\left(B_z^0R\right)\frac{R}{r}$ (for $r<R$), is whirlpooling but at the same time, rot-operator free ($\text{rot}{\mathbf A}=\mathbf{0}$) and div-operator free ($\text{div}\mathbf{A}=0$). Further, this function cannot be expressed in terms of $-\text{grad}\Psi$ type expression if $\Psi$ is single valued. Yes, I agree that the domain is not singly connected.

Outside the static infinite solenoid the magnetic field is 0, but the vector potential is not zero. The vector potential outside the solenoid is an unobservable fictitious object if the system is static. But if the system is time-varying, the non-zero electric field outside the solenoid appears as $-\dot{\mathbf{A}}$; this reminds me of the famous Aharonov-Bohm effect.

Finally, I would like to add a comment about neglecting the displacement current. The reason we can safely neglect the displacement current is because it is very small in the vicinity of the lab solenoid. In the Wikipedia article, there seems to be no concern about the displacement current.