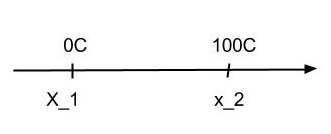In the proof of the relation F=(9/5)C+32 (degrees celsius to fahrenheit) we assume that there is a straight-line (linear) relation between the two temperature scales: F=mC+b. Then we need two "points" to find the equation for that line.
How can we explain to a student the very form (i.e. linear) of the relation among these temperature scales (or between kelvin and degrees celcius, rankine and degrees fahrenheit as well as kelvin and rankine)?
Those temperature scales as defined in the SI system of units are related to the SI unit of temperature the kelvin, K.
On the widely used Celsius temperature scale one degree Celsius is an interval of 1 K, and zero degrees Celsius is 273.16 K.
An interval of one Celsius degree corresponds to an interval of 1.8 Fahrenheit degrees on the Fahrenheit temperature scale.
Hence by definition the relationship between these different scales of temperature is linear and your relationship $F=\frac{9}{5}C+32$ is a consequence of this definition.
The numerical constants are a product of history and the fact that the degree Celsius and the degree Fahrenheit are so widely used.
The fact that although a mercury in glass thermometer calibrated to have 100 equal divisions between the ice point and the steam point measures, the temperature of an object which is only approximately the same as that measured using an alcohol in glass thermometer calibrated to have 100 equal divisions between the ice point and the steam point meant that one temperature scale needed to be defined if more accurate and reproducible temperatures needed to be measured.
Another way of putting this is that if the thermal expansion of mercury (or alcohol or....), the length of the liquid column, is measured relative to the currently defined degree Celsius scale the relationship between expansion and temperature is not (exactly) linear but for some purposes can be taken to be approximately linear.
In order to define a scale of temperature we need to follow three steps:
Actually, your question is related to the third step. Let us say we are controlling the volume of a portion of mercury. We define that when in thermal equilibrium with melting ice the volume $x_1$ of mercury corresponds to $0^oC$. Similarly at boiling water we define $x_2$ corresponding to $100^oC$. Then we choose to linearly interpolate the points between $x_1$ and $x_2$. We have something like the figure bellow:
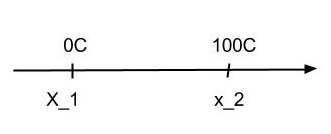
where the intermediate temperatures grows linearly with the intermediate values between $x_1$ and $x_2$.
On the other hand, let us again consider the volume of mercury and let us associate (by definition) the volumes $x_1$ and $x_2$, at melting and boiling point, to the temperatures $32F$ and $212F$. Finally we decide to use linear interpolation again. Hence if we plot temperature in Fahrenheit versus volume we obtain a straight line (dotted line), i.e.,
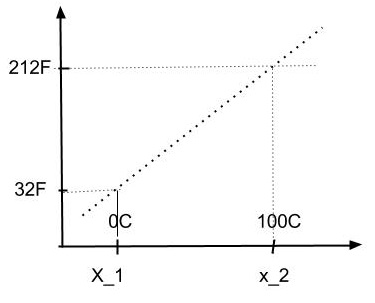
As we can see, the dotted line, which is a straight line also provides a function between the abcissa (C or volume) and the ordinate (F). That is the temperatures in Fahrenheit and Celsius obey a linear relation, $$T_F=A+BT_C.$$ Note that linear relations are valid between two scales as long as both have linear interpolations.
I think this might be one possible way to explain why they both are linearly dependent. This might not be the best reason but is definitely a good explanation.
We measure the temperatures based on the thermometers which work on the principle of expansion of matter. Since we know that the material used in the thermometers expands linearly with temperature we can conclude that the temperature in any scale measured by the thermometer will be linearly related to each other.
If the (approximate) linearity of temperature was inaccurate, then two thermometers with the same two calibration points but constructed differently would record different intermediate temperatures, particularly near mid-way between the two calibration points. Two such different constructions might employ distinct thermo-sensitive materials, for example mercury and alcohol as was done historically.
Since this doesn't occur, the (approximate) linearity is empirically established.
This might make a good experiment for your class.
Since all of these scales could be printed on the same mercury thermometer, and because each scale is made of equal-length units, a linear relationship between scales is the only one possible.
This linear relationship is still true even if there is no mercury in the thermometer.
If we demand that our temperature scale respects the zero'th law of thermodynamics and it is an integrating factor to the heat vectorfield, then our temperature scale is unique up to linear rescaling (affine rescaling not even allowed): the elaboration required for such a statement is adequately contained in my answer to this question, so I will not repeat it here.
