Length of funnel for smooth air flow

Question

I'd like to know if there is a simple way to approximate the minimum length a funnel should be in order to have smooth airflow within. More specifically, my problem is that I want to place a 70cm diameter fan a distance away from a 4x4m panel of filters, with the fan above the center of the filters, blowing air as equally as possible through the filters (and not just through the innermost filters.

Here's a rough drawing of what I'm trying to make:

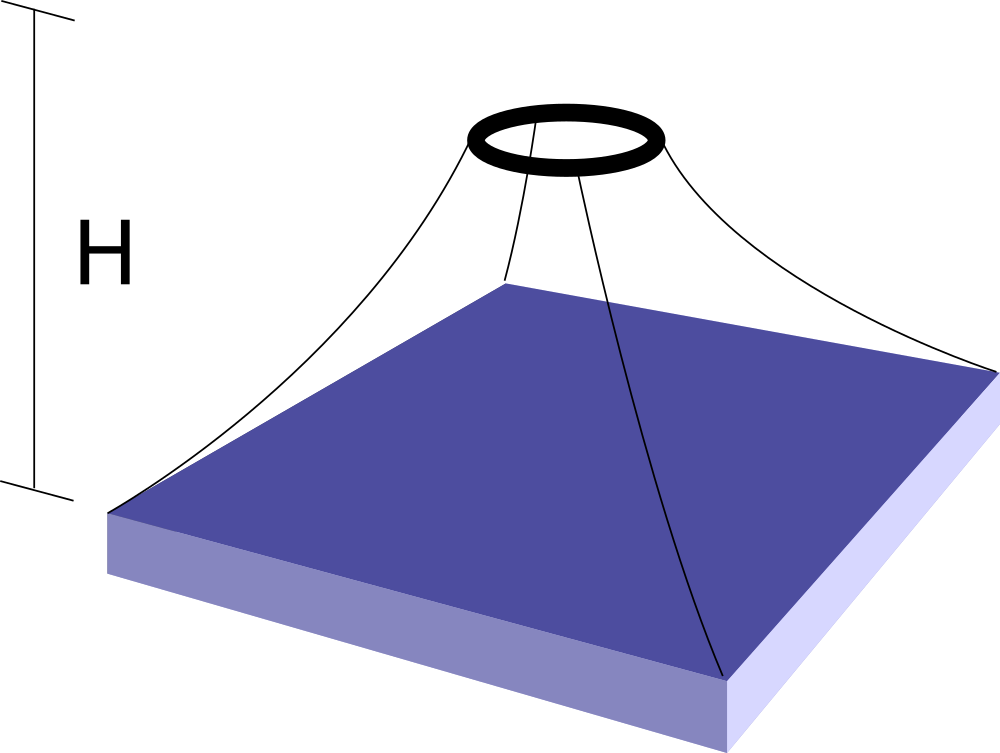I'm interested in knowing what H should be to allow the air from the fan to pass through the filters at the bottom evenly. I wondered if perhaps there was an equation that gives an approximate answer under certain assumptions (e.g. assuming increase in cross-sectional area of funnel is constant per unit height, instead of curved as I have drawn it, assuming walls are rigid, etc.) without resorting to solving PDEs or CFD, etc. Or am I approaching this problem wrongly?

Answer 1

If you assume that the divergence angle of the cone is relatively small and that the flow is relatively incompressible, you can approximate the downward velocity component as roughly uniformly distributed, and, from the continuity equation, you can then solve for the radial velocity component at each cross section. You can then estimate the magnitude of the velocity at the center and at the edge, just above the layer of filters. Using Bernoulli, this will enable you to determine the difference in pressure between the center and edge. The result you get for the pressure difference will be proportional to the dynamic pressure, multiplied by the square of the rate of change of radius with height. The flow non-uniformity through the layer of filters will be equal to this pressure difference divided by the pressure drop across the layer of filters. If you want to crudely bound this answer, just make the pressure drop across the layer of filters large compared to the dynamic pressure.