Ignoring dark matter, Kepler's third law would yield different results for the mass of the black hole depending on the distance of the orbiting star from the black hole.
Is it possible to make the calculation without incorporating dark matter? Perhaps we could look at a star that is located along the highest point in the galaxy rotation curve, before dark matter seems to take over?
Supermassive black holes are found near the centres of many galaxies. Dark matter is distributed much more widely, and although centrally concentrated, the contribution of dark matter to the "rotation curve" near the centre of a galaxy is very small. i.e. the integral of the dark matter density distribution over a sphere of modest radius is quite small compared with the baryonic mass within the same sphere. It is only when you move out beyond a few kpc (in a galaxy like the Milky Way) that dark matter starts to dominate the mass interior to that radius.
For that reason it is perfectly feasible to use Kepler's third law to model the dynamics of gas and stars close to the centre of a galaxy and use this to estimate the mass of the central black hole. Of course you do have to take into account that at each radius on the rotation curve, the Keplerian velocity is that due to all of the mass interior to that radius, which might include the black hole (a point mass) and an extended disk of accreting material (from which the rotation is conveniently measured).
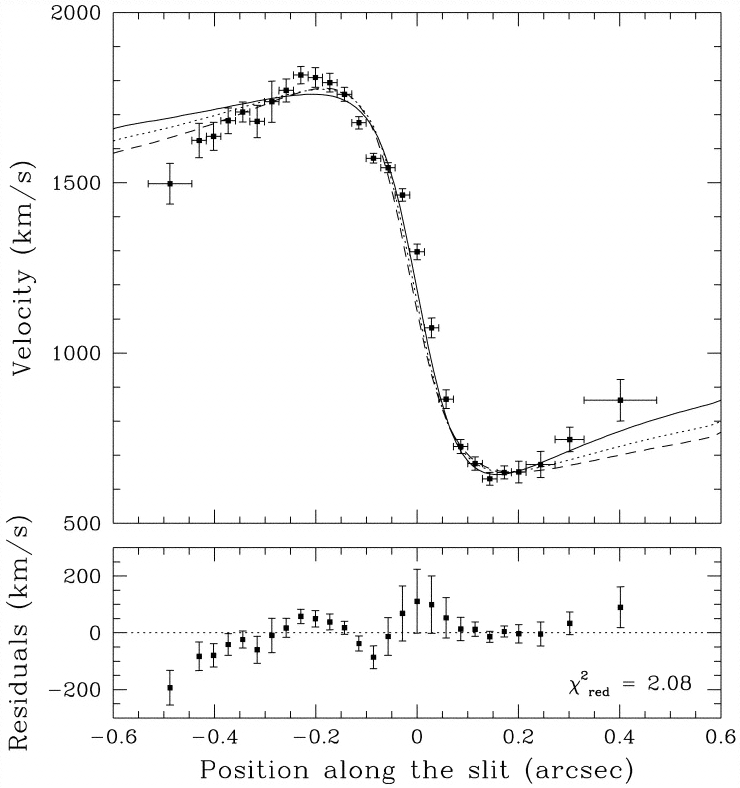
The plot below (from Machetto et al. 1997) shows a rotation curve for gas near the centre of M87. The curves on this plot are "Keplerian disk models".
The entire horizontal extent of this plot covers only the central $\sim 300$ light years of the galaxy, and on this scale the dark matter is dynamically unimportant.
If we are far from any central mass, there is no difference between Newtonian gravity and general relativity. If we are close to the central mass, effects like frame-dragging and perehelion procession start showing up, and Kepler's laws are no longer obeyed.
