The technically correct answer is: As far out as the mass distribution is well approximated by a mass density that is growing linearly in radius (a).
A more observational answer would be: For most galaxies, as far out as we can measure (b).
Many astronomers will say: Out to about two or three times the optical radius (c).
And a practical answer could be: About half-way to the next galaxy (d).
Want more? Read on.
Allow me to first comment on your question: "I know that the galaxy rotation curve doesn't obey general relativity" --- but of course it does! It's just that stars and gas orbit galaxies faster than what we would have expected if visible (baryonic) mass was all there was. Actually, Newtonian gravity is perfectly fine to calculate those rotation curves, no need to involve fancy general relativity.
(a): Let's use a simple 2D model for your galaxy for illustration. Then you can simply equate centripetal force and gravitational force:
$$\frac{mv^2}{r}=\frac{GM(r)m}{r^2}$$
where $m$ is your star's mass and $M(r)$ is the mass of the galaxy out to radius $r$. Solve for the rotation curve:
$$v(r)=\sqrt{\frac{GM(r)}{r}}$$
You know from mechanics 101 that outside a body of mass you can simply set $M(r)=M$ without a need for complicated integration, so for systems like our Solar System you get Kepler's law:
$$v(r)=\sqrt{\frac{GM}{r}}\propto \sqrt{1/r}$$
This is also what you'd expect if stars and gas was all there was in galaxies, because then the mass would be centered around the core of the galaxy, and you'd expect Kepler's law for the rotation curve. But instead, we observe $v(r)\sim \mathrm{const}$. To get that from these equations, you realize that you need $M(r)\propto r$ such that $r$ cancels in the fraction and you get the constant $v$. This gives you the basic mass distribution of the dark matter halo: its mass is growing linearly with radius. As long as that is the case, your rotation curve will be flat.
(b): To get a better overview of our measurements, I recommend this nice and very readable review by Yoshiaki Sofue, a master expert on rotation curves. Out at high radii, there's no stars anymore to trace, but instead the rotation curves come from Doppler shifts of gas clouds. He has somewhat funny plots where he plots lots of rotation curves all on top of each other, and all flat as far out as we can measure.
(c): See e.g. the brief mention in this review (section 6.4)
(d): Indeed you are correct that of course the curve can not stay flat forever as that would imply an infinite amount of dark matter. Once you're half-way to the next galaxy (weighted by their mass for unequal pairs), gravity becomes centered towards that other galaxy. Stars will then stop orbiting your original galaxy, and the notion of a rotation curve fails to make sense.
One particular rotation curve is that of the Andromeda Galaxy. From one of Sofue's papers comes this measurement:
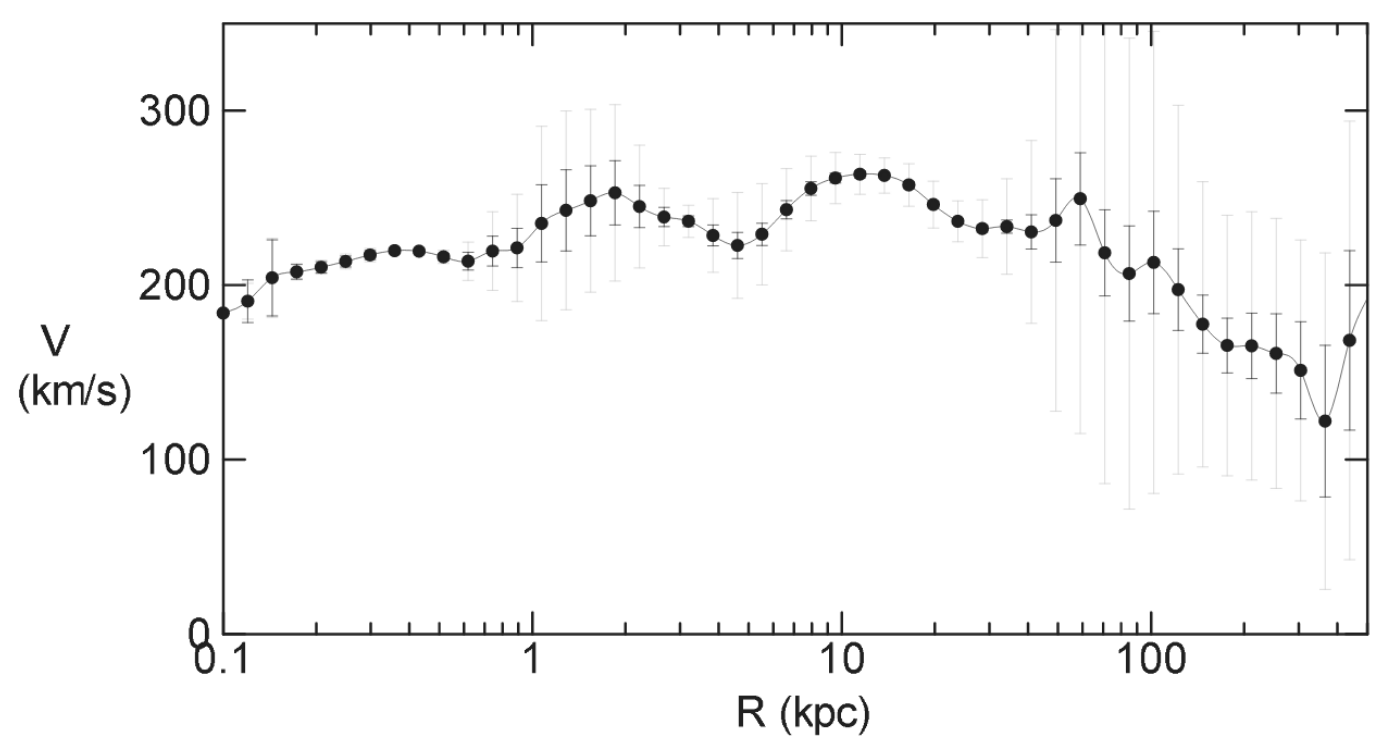 And you can see the rotation curve going down once you get close to about half way between the Milky Way and Andromeda (about 350-400kpc). Yes, that means our dark matter halos are touching.
And you can see the rotation curve going down once you get close to about half way between the Milky Way and Andromeda (about 350-400kpc). Yes, that means our dark matter halos are touching.

