1
$\begingroup$

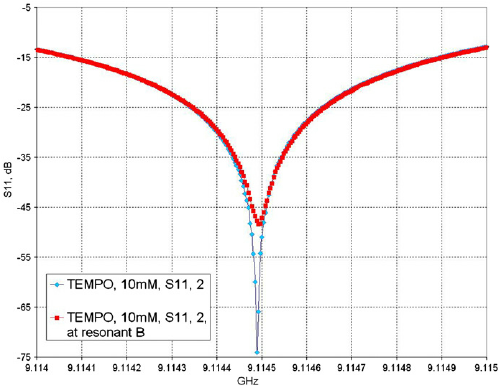
I built a resonator designed to resonate in the 10MHz-1GHz range. To verify the resonant frequency I use a network analyzer with capacitor in series with the cavity to approximate the expected load.

The physicists at my work claim that the signal should measure -20dB or less. Why is this?

My thought process:

\begin{align} \text{Gain}_\text{dB} &= 20 \log \left(\frac{V_\text{out}}{V_\text{in}} \right) = -20 \\ \rightarrow \log \left(\frac{V_\text{in}}{V_\text{out}} \right) &= 1 \\ V_\text{in} &=10*V_\text{out} \end{align} so the attenuated output signal indicates the standing wave in the resonator? Or is the -20dB a general rule of thumb?

Network Analyzer screenshot (Note: This is not my data! This is just an example to assist in clarifying my question)

enter image description here

Improve this question
$\endgroup$
0

2 Answers 2

Reset to default
4
$\begingroup$

I see from your graph that you are measuring S11, ie. return loss.

Return loss is a measure of how well matched a circuit is, if a circuit is not well matched then a lot of the power you insert will be reflected back to the source.

When you measure return loss you are measuring how much of the power you insert is reflected back to the source compared to the power that was inserted, so the ratio of reflected power to inserted power in dB.

Ideally you want (in most cases) no reflection at all, ie. -infinite dB, but that is not possible in practice.

the "-20dB" is a rule of thumb in electrical engineering, if you have a return loss of 20dB or more then in most cases it means your circuit is sufficiently well matched.

Improve this answer
$\endgroup$
1
  • $\begingroup$ There's an aspect of this answer that is very commonly confusing. $S_{11}$ is defined as the ratio of the reflected and input voltage wave amplitudes, i.e. $S_{11} \equiv V_\text{reflect}/V_\text{input}$. When quoting $S_{11}$ in the dB scale, we're actually quoting $10 \log_{10}(|S_{11}|^2)$. $\endgroup$
    – DanielSank
    Commented Jan 28, 2019 at 5:21
1
$\begingroup$

Suppose the ideal case (input and output impedances are equal, and thus your rule for the gain applies), then the "log" in your rule is the logarithm with base 10, not the Napierian one. Remember the 20 comes from 10 (the base of the logarithm) times 2 (the exponent that relates intensity or amplitude to power of the signal) times the log10 of the relation of intensities. A -20 dB gain means only that (for the ideal case) the output signal is 10 times lower than the input signal (without resonance, most of the signal would be lost). And therefore, it is in fact a "rule of thumb", or more like an engineering rule, that implies that your output signal must be at least 1/10th of your input signal.

Improve this answer
$\endgroup$
4
  • $\begingroup$ You are right I'll edit the base 10 mistake. I'm still slightly confused, why is it important that the output signal be at least 1/10th of the input signal? Is the 1/10th indicative of resonance? $\endgroup$
    – goat1123
    Commented Jul 12, 2017 at 5:35
  • $\begingroup$ "...most of the signal would be lost" What do you mean by that? $\endgroup$
    – DanielSank
    Commented Jul 12, 2017 at 5:49
  • 2
    $\begingroup$ One nitpick: The factor 10 in the definition of the unit dB just comes from the fact that dB is decibel not bel. I don't think it has much to do with the base of the logarithm, other than that we generally like the decimal system. $\endgroup$
    – Emil
    Commented Jul 12, 2017 at 7:16
  • 1
    $\begingroup$ You are right, Emil... my bad there. As for the other 2 responses, 1/10th is not indicator of resonance, since some resonance is ALWAYS ACHIEVED (at this scale, resonance is achieved over a continuous spectrum of frequencies). As I said, it's just an engineering rule to state which frequencies should be considered resonant in the system and which shouldn't. But 1/10th is just a criterion, it may vary depending on your design needs. For DanielSank, if you apply a signal through a resonator, frequencies outside the resonance range will be more heavily attenuated over time. That's what I meant. $\endgroup$
    – LukeSW
    Commented Jul 21, 2017 at 3:06

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.