What is the difference between traction and stress?

Question

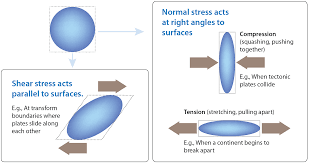
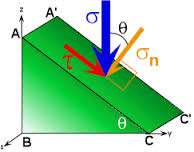
As far as I can tell, stress and traction seem to refer to similar ideas. According to Chapter 8 of Twiss and Moore's Structural Geology, traction is

Force per unit area on a surface of a specified orientation (Twiss, 129)

while stress is

A pair of equal and opposite tractions acting across a surface of specified orientation (Twiss, 129)

I don't understand this latter concept. Why have a term at all if the forces are equal but opposite? Then they should cancel out and be irrelevant right?

What is stress and how is it different from traction?

Answer 1

Your book is giving you an oversimplified description (because it is written for neophytes), and that is part of what is confusing you. Stress is a 2nd order tensor entity (called the stress tensor), and, in component form, requires 6 numbers to specify the state of stress at a specific location in space. The stress tensor can be used to determine the traction acting on any surface of specified orientation. So, once you know these 6 components, you can determine the normal and tangential traction on a surface. The components of the stress tensor can be arranged in a symmetric 3x3 matrix and, when matrix multiplied by a 3x1 column vector representing a unit normal to a specified surface, delivers a 3x1 column vector representing the components of the traction exerted by the material on one side of the surface acting on the material on the other side of the surface. This is called the Cauchy stress relationship. I hope that this makes some kind of sense to you.

Answer 2

Maybe it's best illustrated by these two pictures (which sometimes say more than 1000 words):

The first picture represents stress, of which there are three different kinds, as you can see (therefore stress is a second order tensor quantity).

The second picture represents traction. The red $\tau$ vector is the component of the traction in the direction of the surface.

As you can read, in the definition of traction your book is talking about a force on a surface, while in the definition of stress force across a surface is mentioned.

Answer 3

Denote traction vector as $t_j$, stress tensor as $\sigma_{ij}$ and the normal vector of a surface as $n_i$. Then $$t_j=n_i\sigma_{ij}$$ which is called Cauchy’s relation.

Answer 4

The traction vector is not the force parallel to the surface. The direction of the traction vector depends on the stress tensor and the orientation of the boundary, as stated earlier. The formula that relates the stress tensor to the traction vector is based on equilibrium requirements.

Answer 5

1. Stress is second order tensor whereas traction is a vector.
2. Stress is always defined at a point but Traction is always defined on a specific plane
3. Traction is a more general term.
4. Stress has 6 component but traction has 3 component.
5. The stress tensor can be used to determine the traction acting on any surface of specified orientation. So, once you know these 6 components, you can determine the normal and tangential traction on a surface

Answer 6

Traction is the force parallel to a surface. It cannot create any stress on that plane, in the direction of traction. Hence,Traction to be used as a substitute for stress creates confusion in mind. Only the normal component of an applied force create stress.An inclined applied force is resolved into normal force and traction , the normal component creates stress, not the traction component.The stress works in the direction of applied force. Hence stress working on a inclined plane is reduced by sin(theta) component where theta is the angle between applied force and plane. The above stress is then resolved into sin and cos component to get normal stress and shear stress. Shear stress is not the result of traction force. This is my personal opinion.