Why does tension not do work in this spool reel problem? [duplicate]

Question

In the solution they are just using the conservation of energy principle, i.e.: $PE_1$ + $KE_1$ = $PE_2 $+$ KE_2$ leaving out any work (smooth surface).

I don't understand how tension doesn't do any work. The rope is attached to the spool and the spool is rotating and sliding.

Is it only because the displacement of tension is 0 because as it unravels, the points of contact change? That's the only logical explanation I can come up with...

EDIT:

"They" are the authors of the textbook. And here's the solution where they use $T_1 + V_1 = T_2 + V_2$, where $T=KE$ and $V=PE$.

EDIT 2:

Full problem statement.

Answer 1

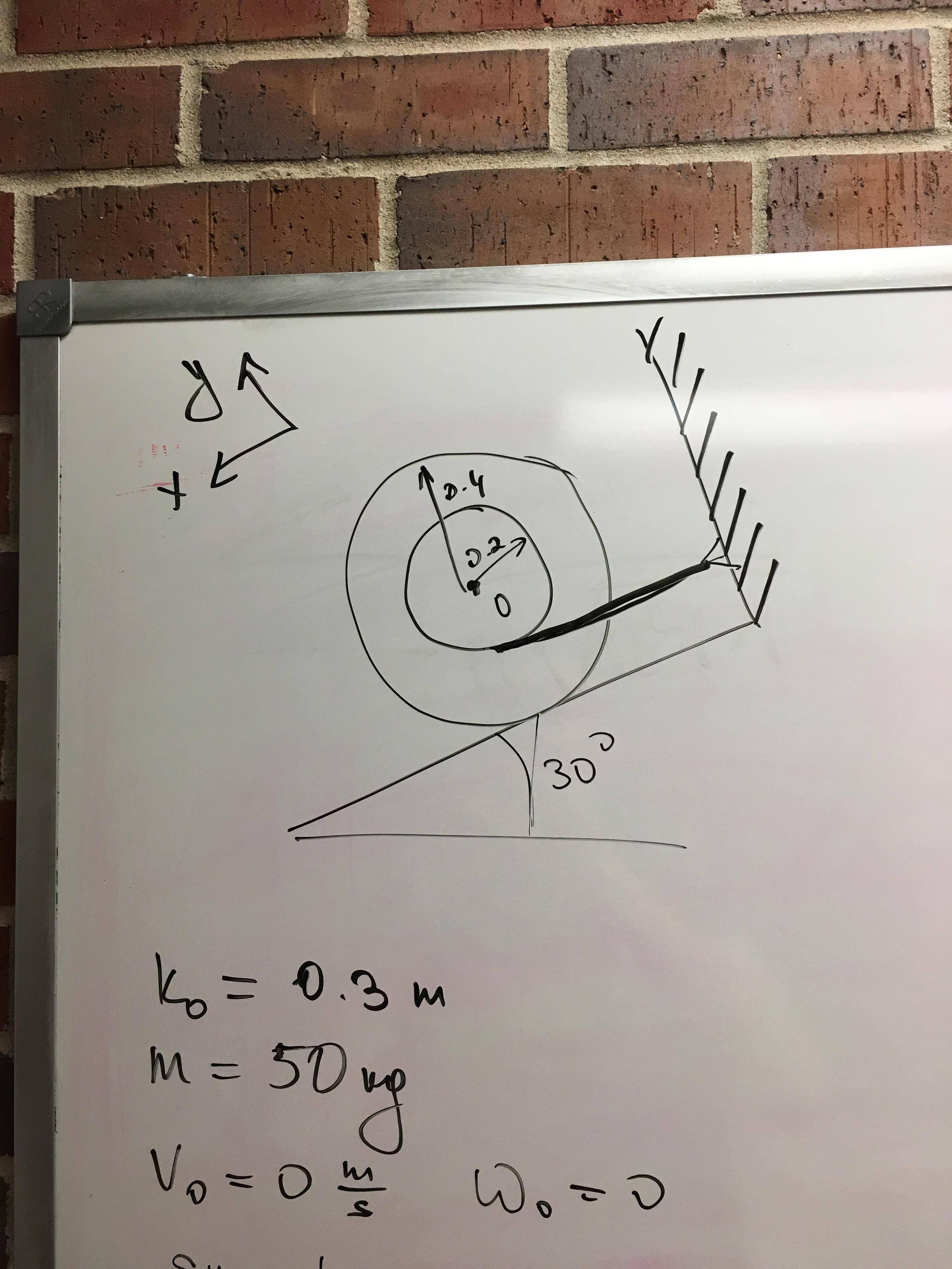
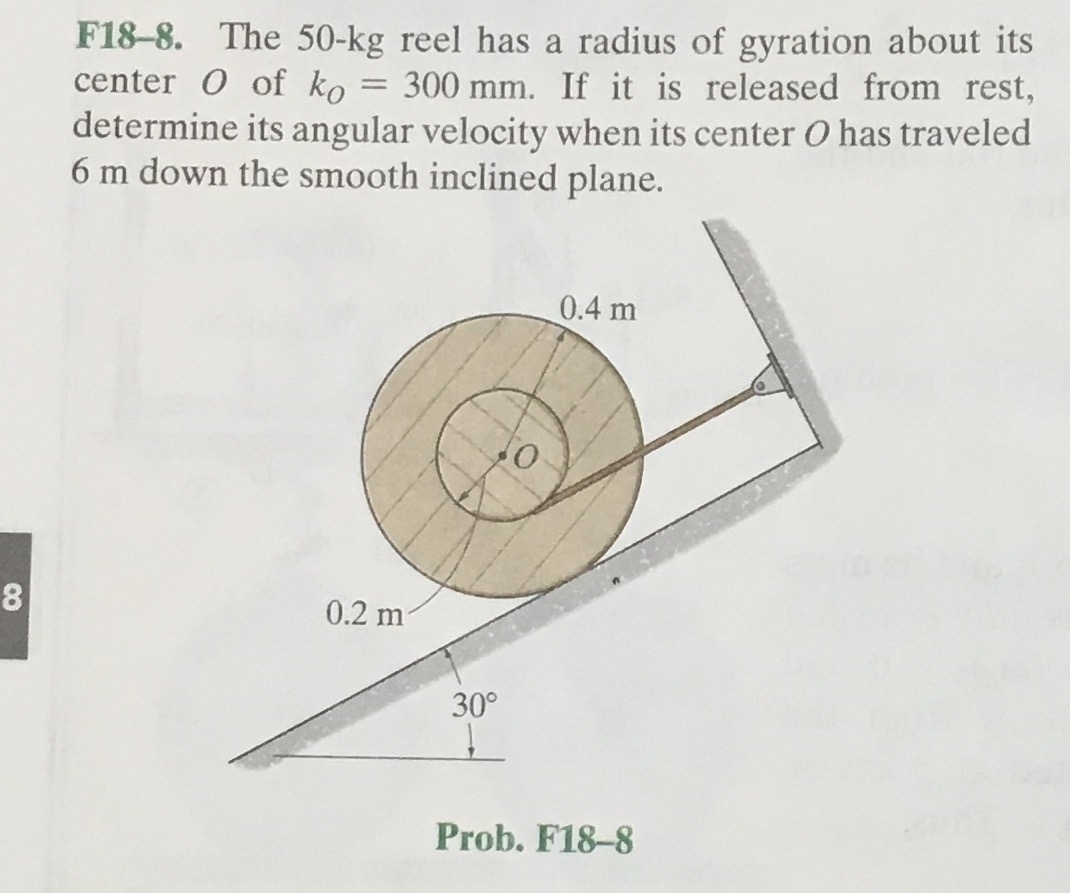
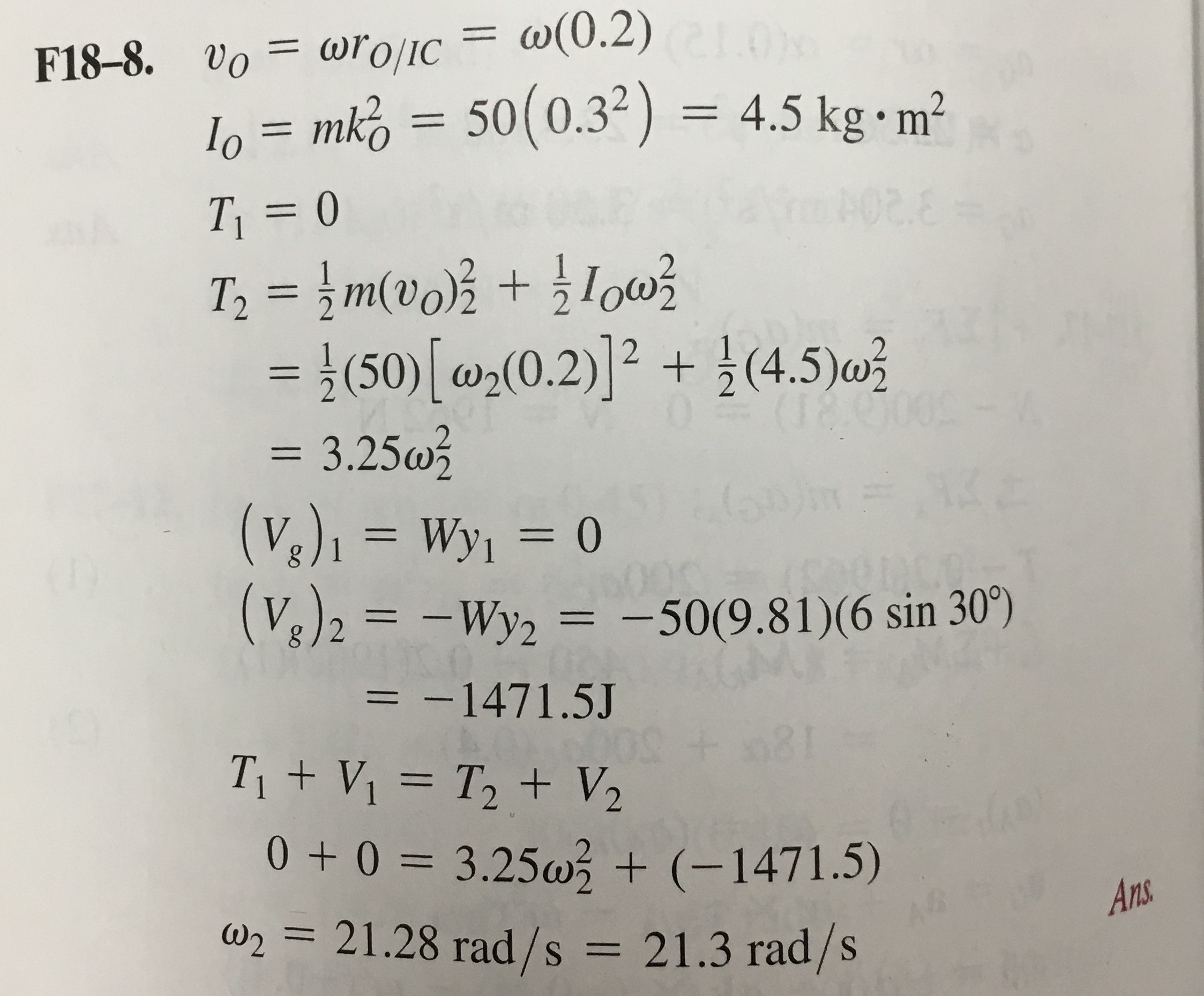Edit:

I seem to have misunderstood the question. The frictionless case here is not between the spool and string, but rather the spool and slope. There is static friction between the string and spool as pointed out by Armando. If we take this to mean that the string doesn't "slip" on the spool, then it doesn't change much and the equation the solution uses is correct (as far as I can tell).

If you can't see how the tension doesn't do any work, picture this. Imagine a motorcycle chain around a gear that is on a frictionless axle. You attach the motorcycle chain to a mass that falls a certain height. $X$ Newtons would be the weight of the mass and how then do you calculate the rotational kinetic energy of the gear ? You could equate the loss in gravitational potential energy to the gained rotational kinetic energy of the gear. Since the chain doesn't slip/rub against the gear, we have eliminated sources of sliding friction while maintaining an analog of the static friction. No work is lost in the tension because there is no sliding and no heat generation (static friction produces no heat). The chain doesn't move relative to the gear just like the rope doesn't move relative to the spool.

In the solution they are just using the conservation of energy principle, i.e.: $PE_1 + KE_1 = PE_2+ KE_2$ leaving out any work (smooth surface).

If I understand this sentence correctly, then I think my answer is accurate. No tension will be present if there is no friction. The friction is what "pulls" on the string. No friction = nothing pulling the string because the string would slide frictionless round and round the spool.

Short Answer:

If you neglect friction, the rope slides/unravels perfectly and has nothing to do with the spool moving.

Long Answer:

Alright, think of it this way: if the rope wasn't connected to the wall and instead lay on the slope, would it just flop there and unravel ? Or picture a roll of tape instead of a spool of string, does it become intuitive then that the less sticky the tape, the more easily the tape unravels ?

The key is picturing and understanding that nothing is stopping the string from unfurling except microscopic forces i.e., friction. Mainly friction between the string unravelling and string wound up and friction between string and spool surface. If the spool was perfectly smooth and the string was also perfectly smooth, you would feel no tension if you held the string and let the spool go, because there is nothing "attaching" the string to the spool.

In my home country, we have a toy/game called "gasing" which translates to "top" or "spinning top". In this game, manipulating this friction is key to perfecting your skills in producing an awesome spinning top. You could powder the string, add wax, wind it tight, etc. The key is friction.

Answer 2

The work energy theorem states that $W_{net} = K_f − K_i = ∆K$. It is usually explicitly stated that is it valid for a particle, but not always. It should not really matter. You can zoom out and a finite body will look like a particle. The work energy theorem is still valid. And it is in this case too. If you forget the object's finite size and look at the behavior of the center of mass, you get the correct answer:

$W_{net}=mg\sin\theta\space L-TL=\frac{1}{2}mv_f^2$

Thus, if you zoom out, $T$ looks like it is doing actual work. If you zoom in and look at the object as a system of particles of finite size, then you can see that $T$ is not doing work because it is applied to a particle that is not moving: at any given moment you have $dW=Tdx$, but $dx=0$. It is still true that the total work will be equal to the change in total kinetic energy:

$W=mg\sin \theta \space L=\Delta K_{CM}+\Delta K_{rotation}$

You might ask, what happened to the work made by $T$ that we considered non-zero and contributed to the kinetic energy of the center of mass (let us call it $W_T^{CM}$)? It is still there: $W_T^{CM}=-TL=-\Delta K_{rotation}$ (if $T$ were not there the CM would be moving faster). The reason is that for an extended object you can write (the index $i$ labels each particle in the object):

$dW_T=\sum T dx_i=\sum T dx_i^{CM}+\sum T dx_i^{internal} =dW_T^{CM}+dW_T^{internal}$

where $dx_i^{internal}$ is the displacement of the particle $i$ relative to the CM. In your exercise $dW_T=0$, but you can still imagine that $T$ contributes to both internal (rotational) kinetic energy and CM kinetic energy, because $dW_T^{CM}=-dW_T^{internal}\ne0$.