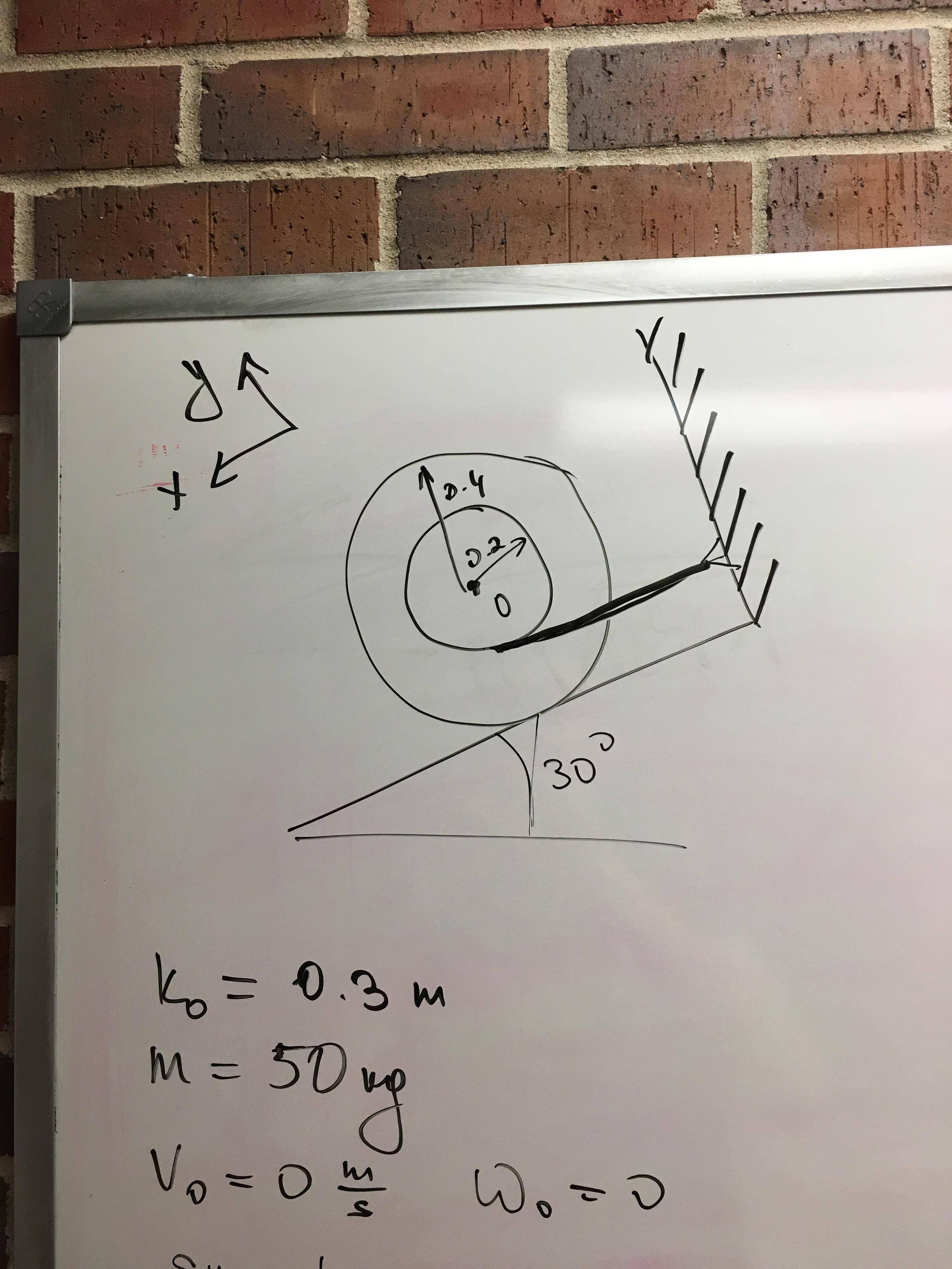
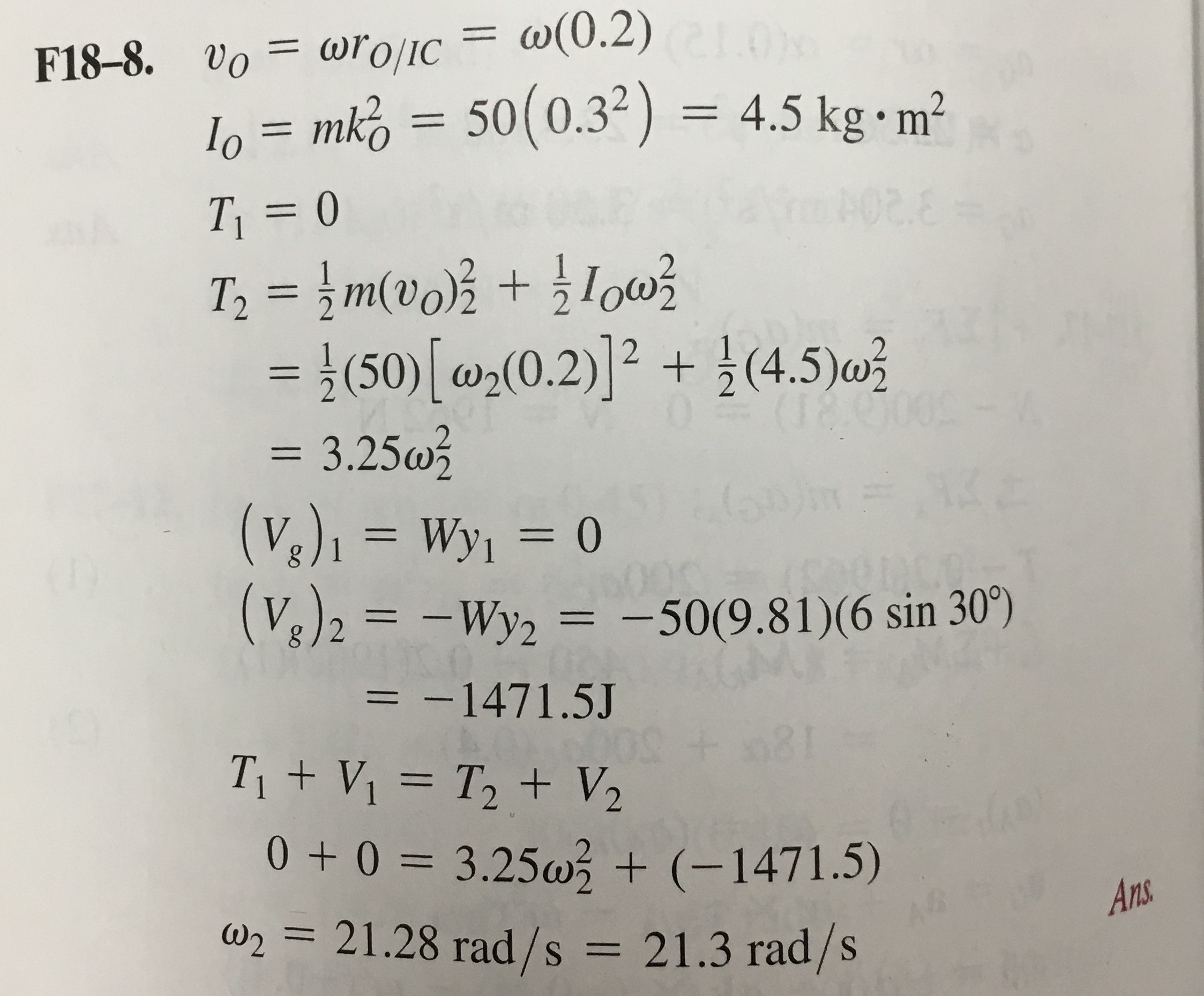In the solution they are just using the conservation of energy principle, i.e.: $PE_1$ + $KE_1$ = $PE_2 $+$ KE_2$ leaving out any work (smooth surface).
I don't understand how tension doesn't do any work. The rope is attached to the spool and the spool is rotating and sliding.
Is it only because the displacement of tension is 0 because as it unravels, the points of contact change? That's the only logical explanation I can come up with...
EDIT:
"They" are the authors of the textbook. And here's the solution where they use $T_1 + V_1 = T_2 + V_2$, where $T=KE$ and $V=PE$.
EDIT 2:
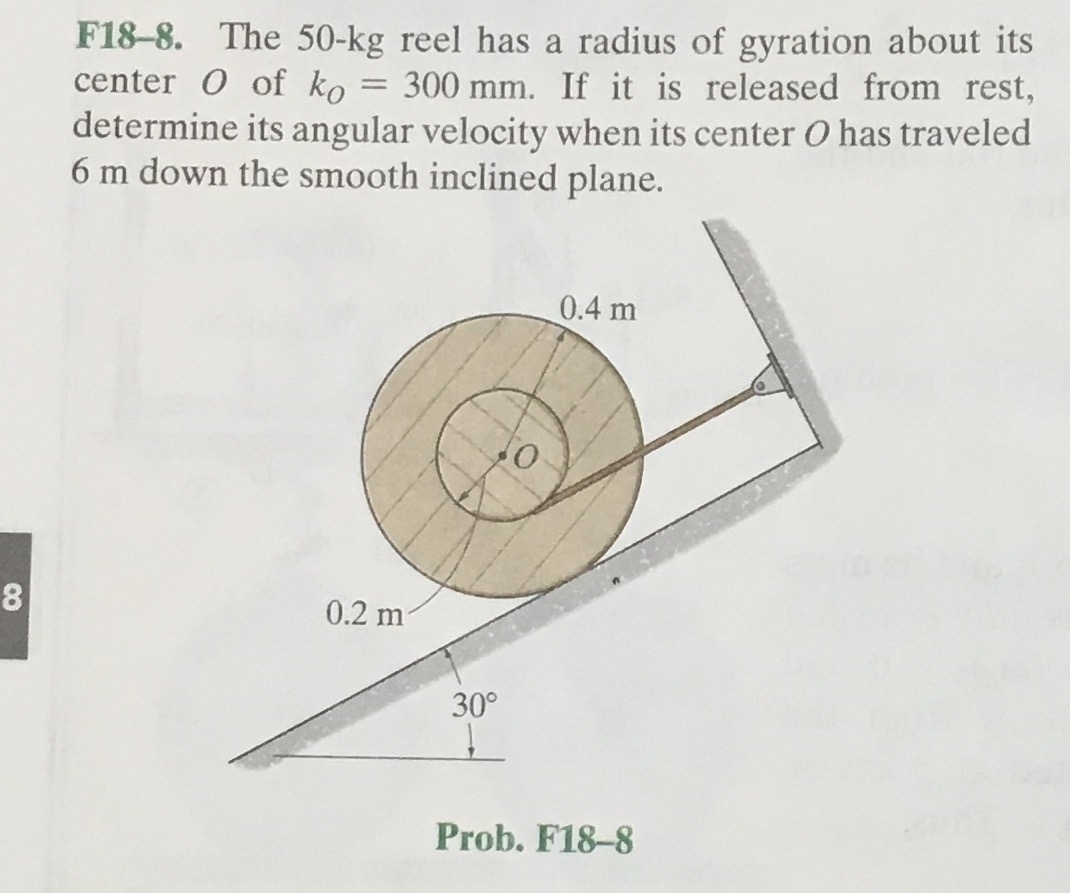
Full problem statement.