Calculating invariant amplitude from Feynman rules

Question

I am having a bit of difficulty understanding how to go from known Feynman rules to an invariant amplitude. My understanding was that you took the vertices, imposed conservation of momentum (integrated over outgoing momenta whilst inserting a conservation delta function) and then took the squared-modulus of the result.

However, when trying to do an exercise; I have gotten stuck!

The Feynman rules for the process I am interested in are as follows:

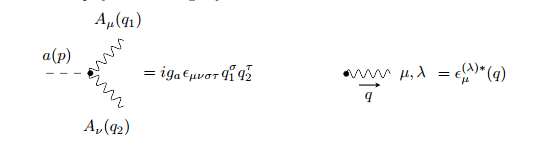
Where $\epsilon_{\mu}^{(\lambda)}(q)$ is the polarization vector for a photon with $4$-momenta $q$ and polarization $\lambda$. For this process, I believe that I have the following:

$$\frac{1}{64\pi^{2}m}\int|\mathcal{A}|^{2}\:\mathrm{d}\Omega$$

Where I am integrating over the entire $4\pi$ solid angle. However, calculating $|\mathcal{A}|^{2}$ is giving me some trouble, I am simply not entirely sure where to start.

Is the following correct:

$$\mathcal{A}_{\lambda_{1},\lambda_{2}}=\iint_{\mathbb{R}^{4}\times \mathbb{R}^{4}}ig_{a}\epsilon_{\mu\nu\sigma\tau}q_{1}^{\sigma}q_{2}^{\tau}\epsilon_{\mu}^{(\lambda_{1})*}(q_{1})\epsilon_{\nu}^{(\lambda_{2})*}(q_{2})\delta^{(4)}(p-q_{1}-q_{2})\:\mathrm{d}^{4}q_{1}\:\mathrm{d}^{4}q_{2}?$$

If so, how should I go about evaluating such an expression, and if not, what is the expression that I should be evaluating; and more importantly, how do I get there!?

---

EDIT: I am given two hints for this problem:

In order to evaluate the sum over polarizations, use: $$\sum_{\lambda}\epsilon_{\mu}^{(\lambda)*}(q)\epsilon_{\nu}^{(\lambda)}(q) = -\eta_{\mu\nu}$$

And:

You may also want to use the identity: $$\epsilon_{\mu\nu\lambda\rho}\epsilon^{\mu\nu\sigma\tau} = -2(\delta_{\lambda}^{\sigma}\delta_{\rho}^{\tau} 2 \delta_{\lambda}^{\tau}\delta_{\rho}^{\sigma})$$

But I am unsure how to use them. I'm guessing the first comes from taking the average over possibly polarizations $\lambda_{1}$, $\lambda_{2}$, but I'm not sure where the second could come from.

Answer 1

There are two related quantities we might want to compute here:

• The amplitude $\mathcal{A}$ for a particular process.
• The total decay rate of the initial particle.

When computing an amplitude, we must specify precisely which initial state we start with and which final state we end up with. So we can talk of the amplitude that a particle with momentum $p$ decays into two particles with momenta $q_1$ and $q_2$ and polarizations $\lambda_1$ and $\lambda_2$, but we can't talk of the amplitude that a given particle decays into some other particle.

However, we do want to know such things as the probability that a given particle decays into some other particle (in a given time) – this is the total decay rate. To find this, we consider all possible specific processes that would lead to such a decay, calculate the amplitude for each, square each of these amplitudes, and then sum the squares. This final summation is just the ordinary rule for adding probabilities of mutually exclusive events.

The amplitude for the process that a particle with momentum $p$ decays into two particles with momenta $q_1$ and $q_2$ and polarizations $\lambda_1$ and $\lambda_2$, is, by your Feynman rules,

$$ \mathcal{A}(q_1,q_2,\lambda_1,\lambda_2) = i g_a \varepsilon_{\mu \nu \sigma \tau} q_1^\sigma q_2^\tau \epsilon^{(\lambda_1)\mu*}\epsilon^{(\lambda_2)\nu *} \,.$$

We now want to square this amplitude, and then sum over the possible momenta and polarizations of the final state. We also need to include a momentum-conserving delta function. In the rest frame of the initial particle, this fixes the 3-momenta of the final state particles to be equal and opposite, and fixes also the magnitude of this momentum. We are hence left with a sum over only the direction of emission of one of the particles. The decay rate is then given by

$$ \Gamma \propto \sum_{\lambda_1}\sum_{\lambda_2} \int \mathrm{d}\Omega\,|\mathcal{A}(q_1,p-q_1,\lambda_1,\lambda_2)|^2 \,.$$

From here it should become clearer how to make use of the two hints given to you.