Just wanted an explanation for why the frequency graph for the doppler effect (as a source approaches an observer) looks like so:
Let the central $x$-axis value be the time at which the source is infront of the observer
I would've thought it was a parabolic curve with a central maximum at the point where the source is directly in front of the observer
It is not a parabolic curve.
You need the component of the velocity $\vec v_{\rm s}$ along the line joining the source and observer which is $v_{\rm s} \cos \theta$.
$\theta$ changes with time being $\frac \pi 2$ at the point of inflection of the frequency against time graph and this can give you the frequency of the source.
The speed of the source relative to the observer (and the frequency of the source) you can find by using the frequencies when the source is a long way away from the observer, before and after the fly pass.
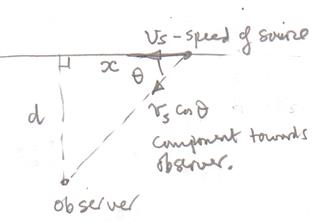
You can also show this with the Pythagorean theorem. Assuming that the distance is the hypotenuse, the leg lengths of the triangle are the distance perpendicular from the path the source takes, and the distance between the point when the source is closest to the observer and the source's current position.
The formula for the length of the hypotenuse of a right triangle with leg lengths $a$ and $b$ is $a^{2} + b^{2} = c^{2}$.
You can use the function $f \left( x \right) = \sqrt{\left( x - a \right)^{2} + b^{2}}$ to model the displacement from the wave source from the perspective of a person standing at $a$ with $b$ being the offset from the point where the source is directly in front of the observer, as you said. You'll notice it makes a hyperbola. Because this is the displacement.
