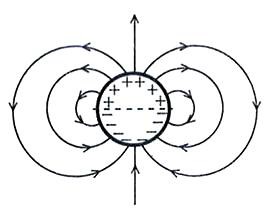
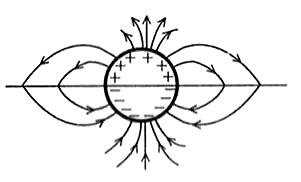
Long cylindrical shell carries positive surface charge σσ in the upper half and negative surface charge −σ−σ in the lower half. The electric field lines around the cylinder will look like figure given in: (figure are schematic and not drawn to scale)
Note: not a homework question another exam question. (there were 4 options and I am confused between these two )
So my approach was: 1) the field lines go from positive to negative charge 2) they must be tangential to surface of cylindrical shell 3) their shape- this is where I am confused.
in what form must they are? and why is the second figure wrong?
a) due to that sudden change in direction? if yes then how is this related to the nature of electric field lines
Also, can someone cite some study material where I can find other problems related to this type and particularly interaction of field lines with metals
Like: when charge kept inside a body with a non-uniform cavity.