No electric field lines yet electric field at a point

Question

My textbook says

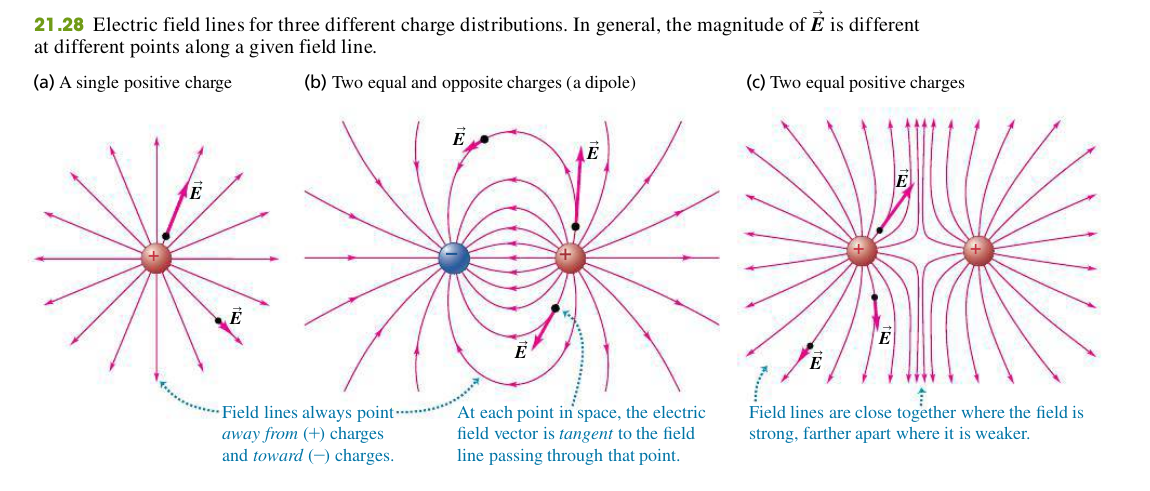
In regions where the field magnitude is large, such as between the positive and negative charges in Fig. 21.28b, the field lines are drawn close together. In regions where the field magnitude is small, such as between the two positive charges in Fig. 21.28c, the lines are widely separated

My question: According to me, We must draw infinite electric field lines around a charge body because the electric field is present at every point ; we don't draw these lines because the process will become so tedious. If I am right Then it doesn't make sense to draw close lines where electric field is strong because lines are closed everywhere we just don't show that.

Answer 1

The electric field produced by charge does not consist of discrete field lines. The actual electric force field permeates all of the space around the charge.

For example, the figure below might just as well be used to visualize the relative strength of the field about a point charge (your book fig A), where the degree of shading is intended to convey the field strength, with no gaps. However, shading alone wouldn't provide information on the direction of the field, particularly for more complex charge distributions than a singe point charge, as do discrete field lines with arrows.

The "price" to pay for using discrete lines is the space between the lines that gives the impression (to many others and not just you) that there is no field in the space between the lines.

Hope this helps.

Answer 2

Yes a charged particle produces infinite electric field lines but this does not mean that their density is constant everywhere in the space.

Field lines is an imaginary concept. It only helps us to understand direction and strength of a force field. (gives a qualitative idea)

More strength (force) means denser field lines. For direction of force we take tangent on the desired point (lying in the field line).

$$\vec{E}=\frac{kQ}{r^{2}}\hat{r}$$

Answer 3

As your textbook says:

the field lines are drawn close together

In other words, this is only a visualization. Just like you draw force arrows, whose length is proportional to the magnitude of the force, for example if you want to understand an inclined plane or a hoist, but you will never find any "arrows" if you look between the slipping mass and the inclined plane, or if you cut the rope of the hoist apart. Assuming that the field consists of field lines is like saying that Mickey Mouse does really exist.

Of course, the field line diagram visualisation is inspired by those experiments where you put iron powder on a sheet of paper where there is a magnet below, and the powder accumulates where the field is strongest and seems to form "lines". But that does not mean that the field is "composed of field lines", because it still depends on the setup of the specific experiment (you should distribute the powder somehow "evenly", and you need iron dust, but with plastic powder, or even copper powder it will fail) and there is no 1:1 correspondence (the strings of iron powder don't show arrows).