Can we observe the p-n Junction voltage Drop?

Question

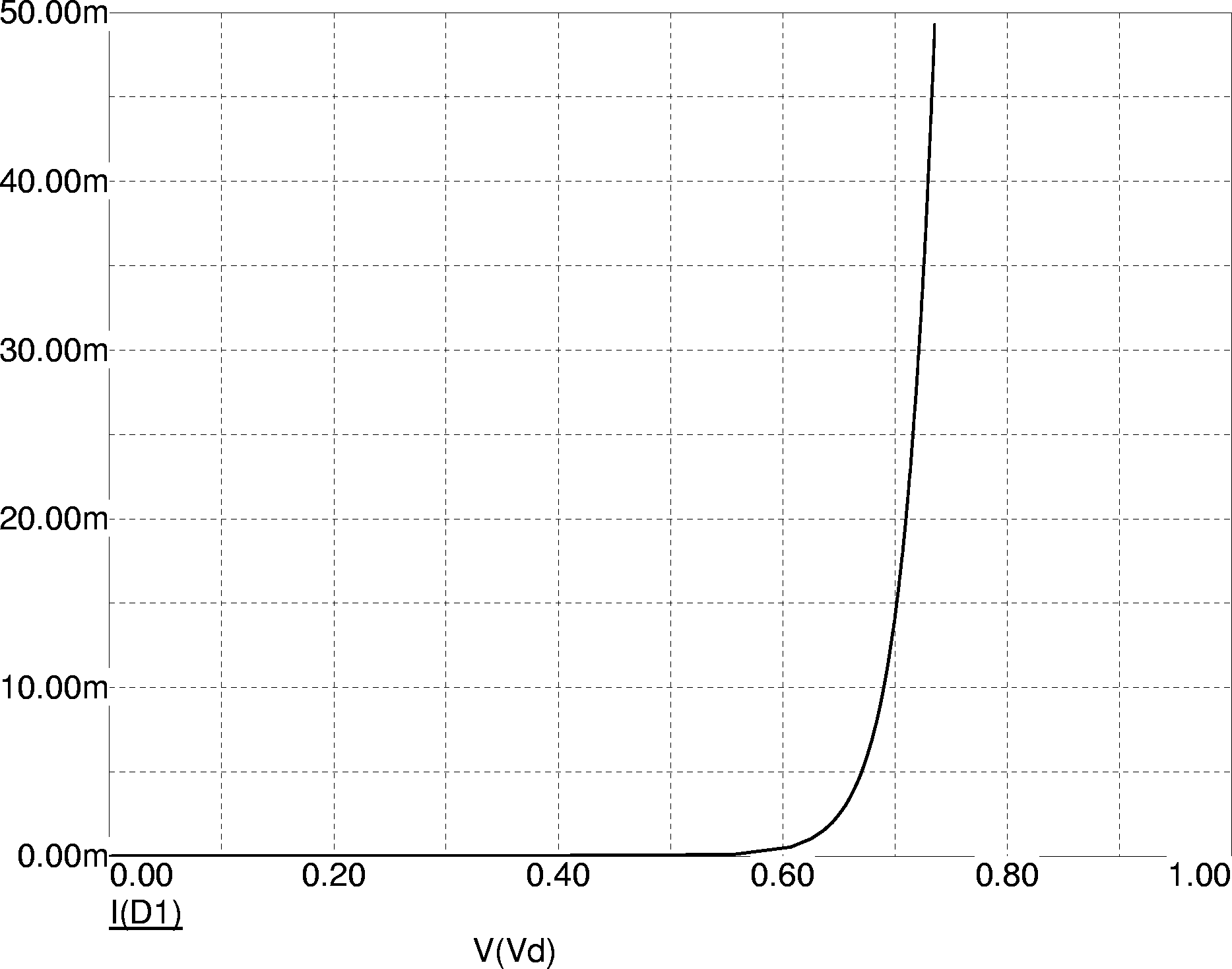
The question is in the title, to clarify: I know that in a P-N-junction, at the boundary, there is going to be a voltage drop in thermal equilibrium. I also know that we can't measure this voltage drop by measuring the potential difference over the whole semiconductor device: If the diode is in thermal equilibrium, and we clamp a voltmeter to the endpoints of the diode, we will measure zero voltage.

But has at anytime somebody anyhow actually observed the voltage drop inside the diode, right at the pn-junction? Or is it just assumed that diode's work this way, because the model yields fine results and can explain the current-voltage behaviour of the diode?

What I imagined was a measurement that gives specific values of the potential inside the diode, at specific points in the diode, shown graphically in an $\Phi$ vs $x$ Diagramm, where x is the spatial distance in the junction.

Answer 1

You can measure the voltage drop across by measuring the voltage required to make a current flow in the forward biased direction.

If you measure the current as a function of voltage for a forward bias you get something like:

(image is from this question)

The knee voltage ($0.65 - 0.7$V in this case) is a measurement of the potential across the junction.